Question

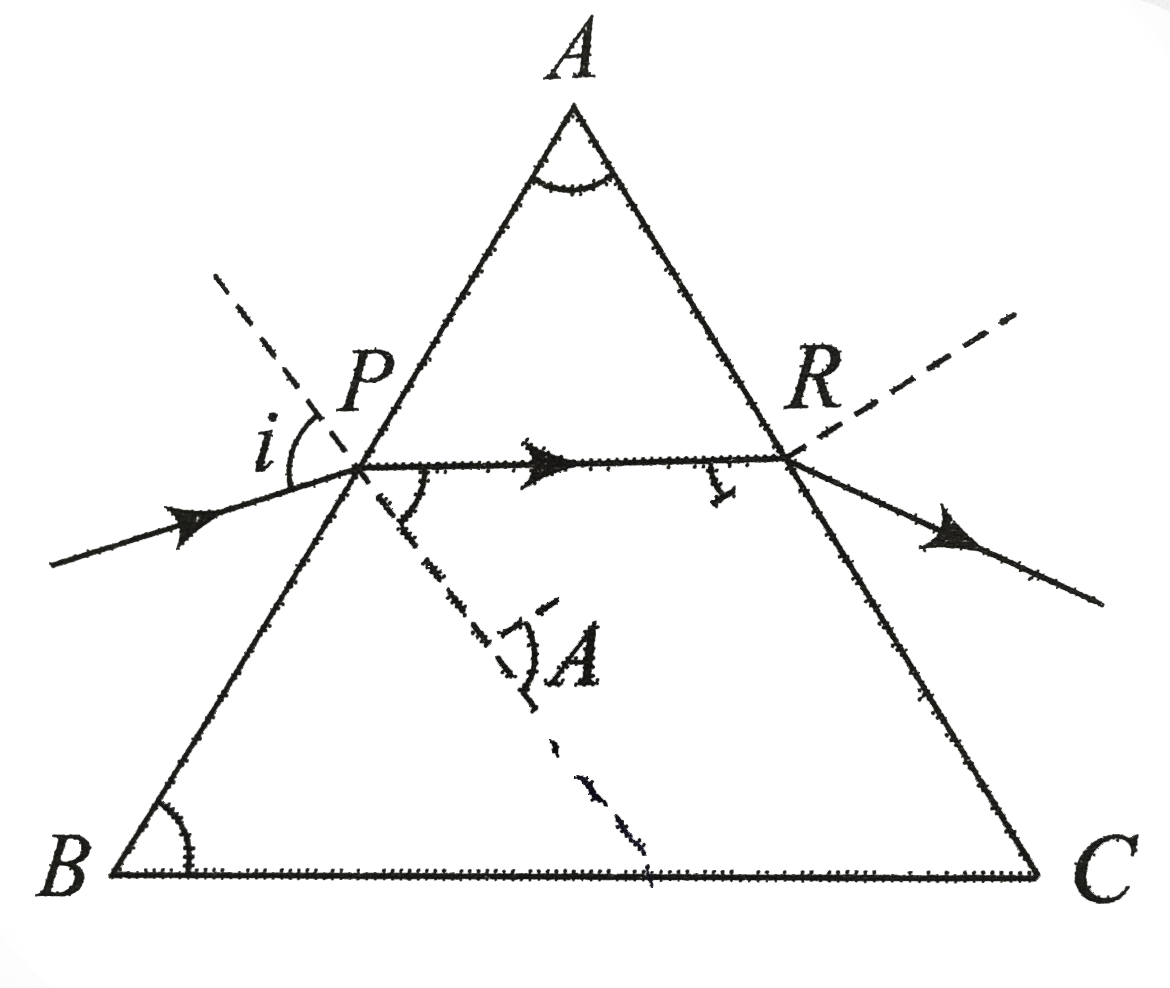
A ray of light undergoes deviation of ${30}^{\circ }$ when incident on an equilateral prism of refractive index $\sqrt{2}$. The angle made by the ray inside the prism with the base of the prism is

Options

A. `15^(@)`
B. `0^(@)`
C. `45^(@)`
D. `30^(@)`

Answer

B

Explanation

To solve the problem, we need to find the angle made by the ray inside the prism with the base of the prism when a ray of light undergoes a deviation of $${30}^{\circ }$$ while passing through an equilateral prism with a refractive index of $$\sqrt{2}$$.

1. Identify Given Values:
- Angle of deviation (\(\delta\)) = \(30^\circ\)
- Refractive index (\(\mu\)) = \(\sqrt{2}\)
- Angle of the prism (\(A\)) = \(60^\circ\) (since it is an equilateral prism)

2. Use the Formula for Refractive Index:
The relationship between the refractive index, angle of prism, and angle of deviation can be expressed as:
\( \mu = \frac{\sin\left(\frac{A + \delta}{2}\right)}{\sin\left(\frac{A}{2}\right)} \)

3. Substitute the Known Values:
Substitute \(A = 60^\circ\) and \(\delta = 30^\circ\) into the formula:
\( \sqrt{2} = \frac{\sin\left(\frac{60^\circ + 30^\circ}{2}\right)}{\sin\left(\frac{60^\circ}{2}\right)} \)
This simplifies to:
\( \sqrt{2} = \frac{\sin\left(45^\circ\right)}{\sin\left(30^\circ\right)} \)

4. Calculate the Sine Values:
- \(\sin(45^\circ) = \frac{1}{\sqrt{2}}\)
- \(\sin(30^\circ) = \frac{1}{2}\)

5. Substitute the Sine Values:
Substitute the sine values back into the equation:
\( \sqrt{2} = \frac{\frac{1}{\sqrt{2}}}{\frac{1}{2}} \)
Simplifying this gives:
\( \sqrt{2} = \frac{1}{\sqrt{2}} \cdot 2 = \frac{2}{\sqrt{2}} = \sqrt{2} \)
This confirms that our values are consistent.

6. Determine the Angle Inside the Prism:
At minimum deviation, the ray travels parallel to the base of the prism. Therefore, the angle made by the ray inside the prism with the base of the prism is:
\( \text{Angle with the base} = 0^\circ \)

Final Answer:
The angle made by the ray inside the prism with the base of the prism is \(0^\circ\).