A portion is fired from origin with velocity →v=v0ˆj+v0ˆk in a uniform magnetic field →B=B0ˆj. In the subsequent motion of the proton
its Z co-ordinate can never be negative
its x co-ordinate can never be positive
its x and z co-ordinates cannot be zero at the same time
its y co-ordinate will be proportional to its time of flight
The correct Answer is:b,d
To solve the problem, we need to analyze the motion of a positron fired from the origin with a specific velocity in a uniform magnetic field. Let's break down the solution step by step.
Step 1: Understand the Initial Conditions
The positron is fired from the origin with a velocity vector given by:
→v=v0^j+v0^k
This indicates that the positron has equal components of velocity in the y-direction (j) and z-direction (k).
Step 2: Identify the Magnetic Field
The uniform magnetic field is given by:
→B=B0^j
This means the magnetic field is directed along the y-axis.
Step 3: Determine the Angle Between Velocity and Magnetic Field
The angle between the velocity vector and the magnetic field vector can be calculated. The velocity has components in both the j and k directions, while the magnetic field is only in the j direction. The angle θ between the velocity vector and the magnetic field vector can be found using the dot product:
cos(θ)=→v⋅→B|→v||→B|
Calculating the magnitudes:
|→v|=√(v0)2+(v0)2=v0√2
|→B|=B0
Now, the dot product:
→v⋅→B=(v0^j+v0^k)⋅(B0^j)=v0B0
Thus,
cos(θ)=v0B0v0√2B0=1√2⟹θ=45∘
Step 4: Analyze the Motion
Since the angle between the velocity and the magnetic field is neither 0° nor 90°, the motion of the positron will be helical. The positron will move in a circular path in the plane perpendicular to the magnetic field while also moving linearly along the direction of the magnetic field.
Step 5: Determine the Components of Motion
1. Linear Motion: The component of velocity parallel to the magnetic field (v_y) will result in linear motion along the y-axis:
vy=v0
The position in the y-direction as a function of time (t) will be:
y(t)=v0t
2. Circular Motion: The component of velocity perpendicular to the magnetic field (v_z) will result in circular motion in the x-z plane. The radius of the circular motion can be determined by the Lorentz force:
F=q(→v×→B)
The circular motion will occur in the x-z plane, and the position will depend on the radius and angular frequency.
Step 6: Conclusion
The overall motion of the positron is helical, with:
- Linear motion along the y-axis.
- Circular motion in the x-z plane.
Final Answer
The correct option regarding the motion of the positron is that it will execute helical motion, with its y-coordinate being proportional to time and the x-coordinate being negative.
The positron is fired from the origin with a velocity vector given by:
→v=v0^j+v0^k
This indicates that the positron has equal components of velocity in the y-direction (j) and z-direction (k).
The uniform magnetic field is given by:
→B=B0^j
This means the magnetic field is directed along the y-axis.
The angle between the velocity vector and the magnetic field vector can be calculated. The velocity has components in both the j and k directions, while the magnetic field is only in the j direction. The angle θ between the velocity vector and the magnetic field vector can be found using the dot product:
cos(θ)=→v⋅→B|→v||→B|
Calculating the magnitudes:
|→v|=√(v0)2+(v0)2=v0√2
|→B|=B0
Now, the dot product:
→v⋅→B=(v0^j+v0^k)⋅(B0^j)=v0B0
Thus,
cos(θ)=v0B0v0√2B0=1√2⟹θ=45∘
Since the angle between the velocity and the magnetic field is neither 0° nor 90°, the motion of the positron will be helical. The positron will move in a circular path in the plane perpendicular to the magnetic field while also moving linearly along the direction of the magnetic field.
1. Linear Motion: The component of velocity parallel to the magnetic field (v_y) will result in linear motion along the y-axis:
vy=v0
The position in the y-direction as a function of time (t) will be:
y(t)=v0t
F=q(→v×→B)
The circular motion will occur in the x-z plane, and the position will depend on the radius and angular frequency.
The overall motion of the positron is helical, with:
- Linear motion along the y-axis.
- Circular motion in the x-z plane.
The correct option regarding the motion of the positron is that it will execute helical motion, with its y-coordinate being proportional to time and the x-coordinate being negative.
Topper's Solved these Questions
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Comprehension type Questions
16 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Matrix Matching type Questions
12 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Only One Option is Correct
45 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
- MEASUREMENT AND ERRORSBOOK - DC PANDEY ENGLISHCHAPTER - MEASUREMENT AND ERRORSEXERCISE - Subjective
19 Videos
Similar Questions
In uniform magnetic field, if angle between →vand→Bis0∘<0<90∘, the path of particle is helix. Let v1 be the component of →valong→Bandv2 be the component perpendicular to →B. Suppose p is the pitch. T is the time period and r is the radius of helix. Then
T=2πmqB,r=mv2qB,P=(v1)T
Assume a charged particle of charge q and mass m is released from the origin with velocity →v=v0ˆi−v0ˆk in a uniform magnetic field →B=−B0ˆk.
Angle between v and B is
View SolutionIn uniform magnetic field, if angle between →vand→Bis0∘<0<90∘, the path of particle is helix. Let v1 be the component of →valong→Bandv2 be the component perpendicular to →B. Suppose p is the pitch. T is the time period and r is the radius of helix. Then
T=2πmqB,r=mv2qB,P=(v1)T
Assume a charged particle of charge q and mass m is released from the origin with velocity →v=v0ˆi−v0ˆk in a uniform magnetic field →B=−B0ˆk.
Axis of helix is along
View SolutionIn uniform magnetic field, if angle between →vand→Bis0∘<0<90∘, the path of particle is helix. Let v1 be the component of →valong→Bandv2 be the component perpendicular to →B. Suppose p is the pitch. T is the time period and r is the radius of helix. Then
T=2πmqB,r=mv2qB,P=(v1)T
Assume a charged particle of charge q and mass m is released from the origin with velocity →v=v0ˆi−v0ˆk in a uniform magnetic field →B=−B0ˆk.
Pitch of helical path described by particle is
View SolutionA conductor AB of length l moves in xy plane with velocity →v=v0(ˆi−ˆj). A magnetic field →B=B0(ˆi+ˆj) exists in the region. The induced emf is
View SolutionAn alpha particle moving with the velocity →v=uˆi+ˆj ,in a uniform magnetic field →B=Bˆk . Magnetic force on alpha particle is
View SolutionA particle of charge q and mass m released from origin with velocity →v=v0ˆi into a region of uniform electric and magnetic fields parallel to y-axis. i.e., →E=E0ˆjand→B=B0ˆj. Find out the position of the particle as a functions of time
Strategy : Here →E∣∣→B
The electric field accelerates the particle in y-direction i.e., component of velocity goes on increasing with acceleration
ay=Fym=Fem=qE0m
The magnetic field rotates the particle in a circle in x-z plane (perpendicular to magnetic field) The resultant path of the particle is a helix with increasing pitch.
Velocity of the particle at time t would be
→v(t)=vxˆi+vyˆj+vzˆk
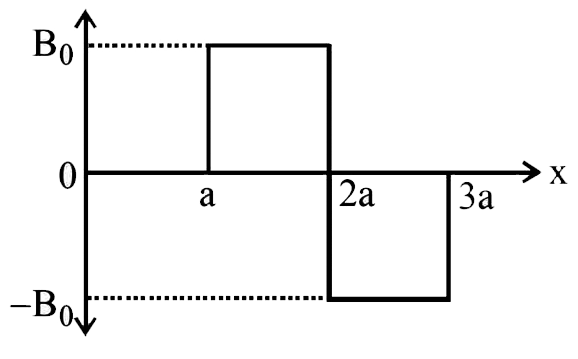
View SolutionA magnetic field →B=B0ˆj , exists in the region a<x<2a , and →B=−B0ˆj , in the region 2a<x<3a, where B0 is a positive constant . A positive point charge moving with a velocity →v=v0ˆi , where v0 is a positive constant , enters the magnetic field at x=a . The trajectory of the charge in this region can be like
View SolutionA charged particle with charge to mass ratio (qm)=(10)319Ckg−1 enters a uniform magnetic field →B=20ˆi+30ˆj+50ˆkT at time t = 0 with velocity →V=(20ˆi+50ˆj+30ˆk)m/s. Assume that magnetic field exists in large space.
During the further motion of the particle in the magnetic field, the angle between the magnetic field and velocity of the particle
View SolutionA charged particle with charge to mass ratio (qm)=(10)319Ckg−1 enters a uniform magnetic field →B=20ˆi+30ˆj+50ˆkT at time t = 0 with velocity →V=(20ˆi+50ˆj+30ˆk)m/s. Assume that magnetic field exists in large space.
The frequency (in Hz) of the revolution of the particle in cycles per second will be
View SolutionA charged particle with charge to mass ratio (qm)=(10)319Ckg−1 enters a uniform magnetic field →B=20ˆi+30ˆj+50ˆkT at time t = 0 with velocity →V=(20ˆi+50ˆj+30ˆk)m/s. Assume that magnetic field exists in large space.
The frequency (in Hz) of the revolution of the particle in cycles per second will be
View Solution
