In simple harmonic motion of a particle, maximum kinetic energy is 40 J and maximum potential energy is 60 J. then
minimum potential energy will be 20 J
potential energy at half the displacement will be 30J
kinetic energy at half the displacement is 40 J
potential energy or kinetic energy at some intermediate position cannot be found the given data
The correct Answer is:A, B
To solve the problem, we need to analyze the simple harmonic motion (SHM) of a particle with the given maximum kinetic energy (KE) and maximum potential energy (PE).
1. Identify Given Values:
- Maximum Kinetic Energy (KE_max) = 40 J
- Maximum Potential Energy (PE_max) = 60 J
2. Calculate Total Mechanical Energy (E):
- In SHM, the total mechanical energy (E) is the sum of maximum kinetic energy and maximum potential energy.
E=KEmax+PEmax=40J+60J=100J
3. Determine Minimum Potential Energy (PE_min):
- At the mean position, the potential energy is minimum (PE_min) and the kinetic energy is maximum.
- Since total mechanical energy is conserved:
PEmin=E−KEmax=100J−40J=60J
4. Calculate Potential Energy at Half the Displacement (X = A/2):
- At half the amplitude (X = A/2), we can find the kinetic energy (KE) and potential energy (PE) using the conservation of energy.
- The potential energy at this position can be calculated as:
PE=E−KE
- To find KE at X = A/2, we use the relationship:
KE=12mω2(A2−X2)
- Substituting X=A2:
KE=12mω2(A2−(A2)2)=12mω2(A2−A24)=12mω2(3A24)
- Therefore, the kinetic energy at half the displacement is:
KE=34KEmax=34×40J=30J
5. Calculate Potential Energy at Half the Displacement:
- Now using the total mechanical energy:
PE=E−KE=100J−30J=70J
6. Summarize the Results:
- Minimum Potential Energy (PE_min) = 60 J
- Potential Energy at half the displacement = 70 J
- Kinetic Energy at half the displacement = 30 J
Final Answers:
- Minimum Potential Energy = 60 J
- Potential Energy at half the displacement = 70 J
- Kinetic Energy at half the displacement = 30 J
- Maximum Kinetic Energy (KE_max) = 40 J
- Maximum Potential Energy (PE_max) = 60 J
- In SHM, the total mechanical energy (E) is the sum of maximum kinetic energy and maximum potential energy.
E=KEmax+PEmax=40J+60J=100J
- At the mean position, the potential energy is minimum (PE_min) and the kinetic energy is maximum.
- Since total mechanical energy is conserved:
PEmin=E−KEmax=100J−40J=60J
- At half the amplitude (X = A/2), we can find the kinetic energy (KE) and potential energy (PE) using the conservation of energy.
- The potential energy at this position can be calculated as:
PE=E−KE
- To find KE at X = A/2, we use the relationship:
KE=12mω2(A2−X2)
- Substituting X=A2:
KE=12mω2(A2−(A2)2)=12mω2(A2−A24)=12mω2(3A24)
- Therefore, the kinetic energy at half the displacement is:
KE=34KEmax=34×40J=30J
- Now using the total mechanical energy:
PE=E−KE=100J−30J=70J
- Minimum Potential Energy (PE_min) = 60 J
- Potential Energy at half the displacement = 70 J
- Kinetic Energy at half the displacement = 30 J
- Minimum Potential Energy = 60 J
- Potential Energy at half the displacement = 70 J
- Kinetic Energy at half the displacement = 30 J
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Comprehension types
18 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Matrix matching type questions
13 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - JEE Advanced
34 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
In simple harmonic motion,the particle is
View SolutionA 20g particle is oscillating simple harmonically with a period of 2sec and maximum kinetic energy 2J. The total mechanical energy of the particle is zero , find
a Amplitude of oscillation
b. potential energy as a function of displacement x relative to mean position.
View SolutionThe particle executing simple harmonic motion has a kinetic energy K0cos2ωt. The maximum values of the potential energy and the energy are respectively
View SolutionIn position A kinetic energy of a particle is 60 J and potential energy is -20 J. In position B, kinetic energy is 100 J and potential energy is 40 J. Then, in moving the particle from A to B
View SolutionA pendulum is executing simple harmonic motion and its maximum kinetic energy is K1. If the length of the pendulum is doubled and it perfoms simple harmonuc motion with the same amplitude as in the first case, its maximum kinetic energy is K2 Then:
View SolutionThe diagram below shows a pendulum having a bob of mass 80 g. A and C are extreme positions and B is the mean position. The bob has velocity 5m/s at position B. Assuming there is no loss of energy, select the correct statements from the options given below: [ g = 10 N/kg ]
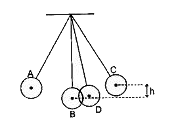
At point A the bob will have only kinetic energy.
The maximum potential energy gained by the bob will be 1000J.
The maximum height 'h' reached by the bob will be 125 cm.
At point D the bob will have maximum kinetic energy.
The maximum potential energy gained by the bob will be 1 J
At point B the energy possessed by the bob is 1000 J.
View SolutionA particle starts oscillating simple harmonically from its equilibrium position then, the ratio of kinetic energy and potential energy of the particle at the time T/12 is: (T=time period)
View Solution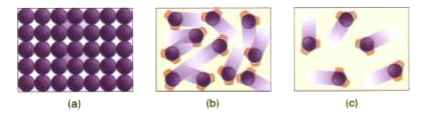
Which of the above state has particles with maximum kinetic energy?
View SolutionA body is executing simple harmonic motion. At a displacement x, its potential energy is E1 and a displacement y, its potential energy is E2. The potential energy E at a displacement (x+y) is
View SolutionA linear harmonic oscillator has a total mechanical energy of 200J. Potential energy of it at mean position is 50J. Find
(i) the maximum kinetic energy,
(ii)the minimum potential energy,
(iii) the potential energy at extreme positions.
View Solution
