Three bodies having masses 5 kg, 4 kg and 2 kg is moving at the speed of 5 m/sand2m//s and 5 m/s respectively along X-axis.The magnitude of velocity of centre of mass is
1.0m/s
4m/s
0.9m/s
1.3m/s
The correct Answer is:B
To find the magnitude of the velocity of the center of mass of the three bodies, we can use the formula for the velocity of the center of mass (V_cm):
Vcm=m1v1+m2v2+m3v3m1+m2+m3
Where:
- m1,m2,m3 are the masses of the bodies,
- v1,v2,v3 are the velocities of the bodies.
Step 1: Identify the masses and velocities
- Masses:
- m1=5kg
- m2=4kg
- m3=2kg
- Velocities:
- v1=5m/s
- v2=2m/s
- v3=5m/s
Step 2: Calculate the total momentum
Calculate the total momentum of the system:
Total Momentum=m1v1+m2v2+m3v3
Substituting the values:
=(5kg×5m/s)+(4kg×2m/s)+(2kg×5m/s)
Calculating each term:
=25kg m/s+8kg m/s+10kg m/s
Adding these together:
=25+8+10=43kg m/s
Step 3: Calculate the total mass
Calculate the total mass of the system:
Total Mass=m1+m2+m3
Substituting the values:
=5kg+4kg+2kg=11kg
Step 4: Calculate the velocity of the center of mass
Now, substitute the total momentum and total mass into the center of mass velocity formula:
Vcm=43kg m/s11kg
Calculating this gives:
Vcm≈3.91m/s
Step 5: Round to the appropriate significant figures
Rounding to two decimal places, we find:
Vcm≈3.91m/s
Final Answer
The magnitude of the velocity of the center of mass is approximately 3.91 m/s.
---
- m1,m2,m3 are the masses of the bodies,
- v1,v2,v3 are the velocities of the bodies.
- Masses:
- m1=5kg
- m2=4kg
- m3=2kg
- v1=5m/s
- v2=2m/s
- v3=5m/s
Calculate the total momentum of the system:
Calculate the total mass of the system:
Now, substitute the total momentum and total mass into the center of mass velocity formula:
Rounding to two decimal places, we find:
The magnitude of the velocity of the center of mass is approximately 3.91 m/s.
Topper's Solved these Questions
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(NEET)
22 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Integer type questions
14 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
Similar Questions
Two particles of mass 1 kg and 0.5 kg are moving in the same direction with speed of 2m/s and 6 m/s respectively on a smooth horizontal surface. The speed of centre of mass of the system is
View SolutionTwo bodies of different masses 2kg and 4kg are moving with velocities 2m/s and 10m/s towards each other due to mutual gravitational attraction. Then the velocity of the centre of mass is
View SolutionTwo particles of mass 1kg and 2kg are moving along the same line with speeds 2m/s and 4m/s respectively. Calculate the speed of the centre of mass of the system if both the particles are moving in the same direction.
View SolutionTwo particles of mass 1kg and 2kg are moving along the same line with speeds 2m/s and 4m/s respectively. Calculate the speed of the centre of mass of the system if both the particles are moving in the same direction.
View SolutionTwo bodies of mass 2 kg and 5 kg have position (1 m, 2 m, 1 m) and (3 m, 2 m, -1 m) respectively. The position vector of centre of mass is
View SolutionTwo particles A and B of equal size but of masses mkg and 2mkg are moving directly towards each other with speeds 21m/s and 4m/s respectively. After collision, A moves with a speed of 1m/s in the original direction. Then:
View SolutionTwo particles A and B of mass 3kg and 4kg moving on a smooth horizontal surface with speed 5m/s and 2m/s respectively collides each other. Coefficient of restitution is e=12, then
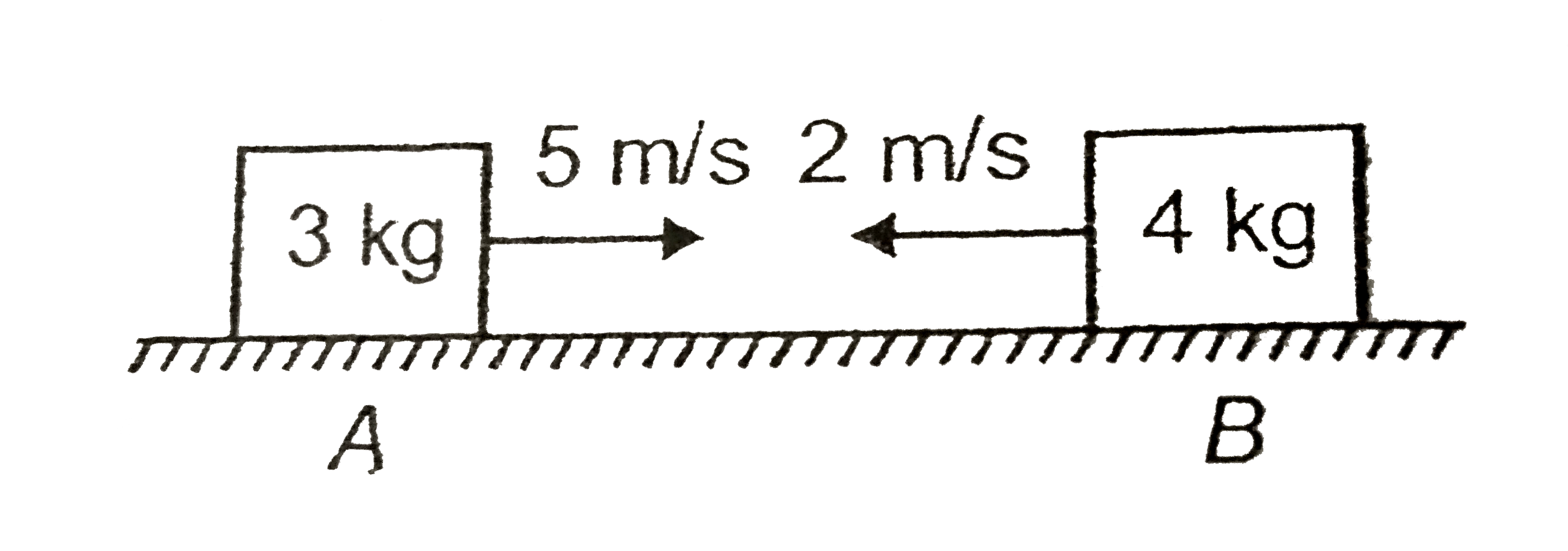
Velocity of B after collision
View SolutionTwo particles A and B of mass 3kg and 4kg moving on a smooth horizontal surface with speed 5m/s and 2m/s respectively collides each other. Coefficient of restitution is e=12, then
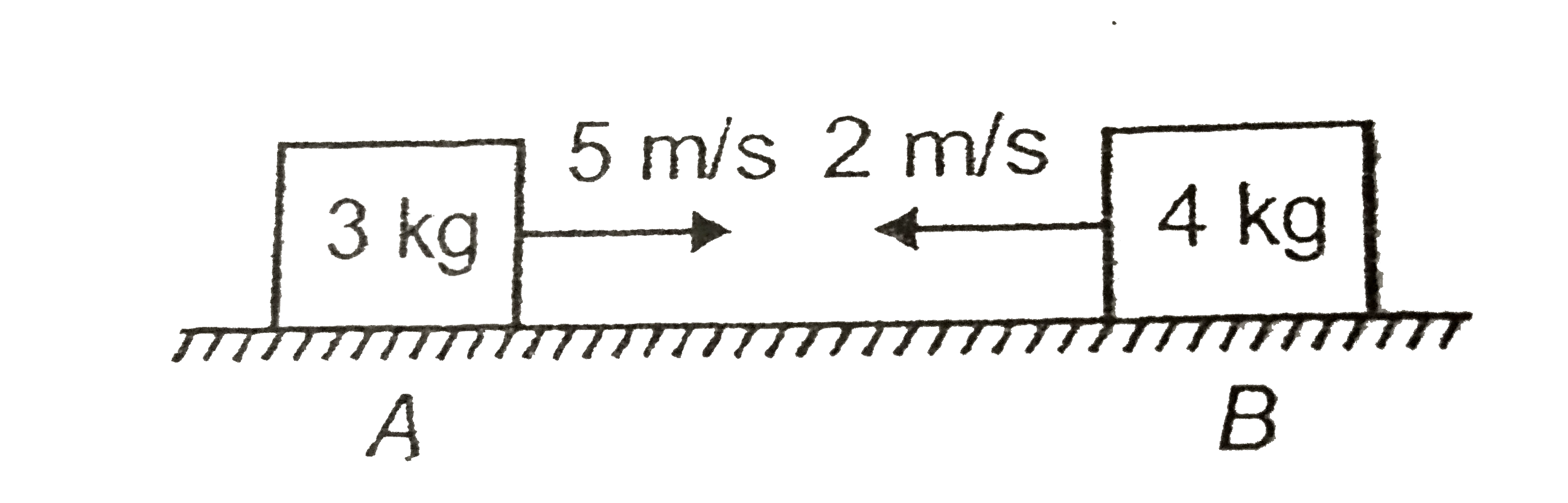
Velocity of A after collision
View SolutionVelocity vectors of three masses 2kg,1kg and 3 kg are →v1=(ˆi−2ˆj+ˆk)ms,→V2=(2ˆi+2ˆj−ˆk)ms and →v3 respectively. If velocity vector of center of mass of the system is zero then value of →v3 will be
View SolutionTwo bodies A and B of masses 5.00 kg and 10.0 kg respectively moving in opposite directions with velocities 4.00 m/s and 0.50 m/s respectively make head-on collision in free space. The force of their mutual interaction varies according to the given graph. Select incorrect statement.

View Solution
