Find the magnitude of the centripetal acceleration of a particle on the tip of a blade, 0.30 metre in diameter, rotating at 1200 revolution per minute.
To find the magnitude of the centripetal acceleration of a particle on the tip of a blade, we will follow these steps:
Step 1: Find the radius of the blade
The diameter of the blade is given as 0.30 meters. The radius R can be calculated using the formula:
R=Diameter2
Substituting the given diameter:
R=0.302=0.15 meters
Step 2: Convert revolutions per minute to revolutions per second
The rotation speed is given as 1200 revolutions per minute (rpm). To convert this to revolutions per second (rps), we divide by 60:
n=120060=20 revolutions per second
Step 3: Calculate the angular velocity
The angular velocity ω in radians per second can be calculated using the formula:
ω=2πn
Substituting the value of n:
ω=2π×20=40π radians per second
Step 4: Calculate the centripetal acceleration
The centripetal acceleration AC is given by the formula:
AC=ω2R
Substituting the values of ω and R:
AC=(40π)2×0.15
Calculating (40π)2:
(40π)2=1600π2
Now substituting this back into the equation:
AC=1600π2×0.15
Calculating this gives:
AC=240π2 m/s2
Using π≈3.14:
AC≈240×(3.14)2≈240×9.8596≈2366.3 m/s2
Final Answer
The magnitude of the centripetal acceleration is approximately:
AC≈2366.3 m/s2
---
The diameter of the blade is given as 0.30 meters. The radius R can be calculated using the formula:
R=Diameter2
Substituting the given diameter:
R=0.302=0.15 meters
The rotation speed is given as 1200 revolutions per minute (rpm). To convert this to revolutions per second (rps), we divide by 60:
n=120060=20 revolutions per second
The angular velocity ω in radians per second can be calculated using the formula:
ω=2πn
Substituting the value of n:
ω=2π×20=40π radians per second
The centripetal acceleration AC is given by the formula:
AC=ω2R
Substituting the values of ω and R:
AC=(40π)2×0.15
Calculating (40π)2:
(40π)2=1600π2
Now substituting this back into the equation:
AC=1600π2×0.15
Calculating this gives:
AC=240π2 m/s2
Using π≈3.14:
AC≈240×(3.14)2≈240×9.8596≈2366.3 m/s2
The magnitude of the centripetal acceleration is approximately:
AC≈2366.3 m/s2
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Solved Examples
2 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Miscellaneous Examples
5 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
Find the magnitude of the linear acceleration of a particle moving in a circle of radius 10 cm with uniform speed completing the circle in 4s.
View SolutionFind the magnitude of the linear acceleration of a particle moving in a circle of radius 10 cm with uniform speed completing the circle in 4s.
View SolutionThe diameter of the front and the rear wheels of a tractor are 63 cm and 1.54 m respectively. The rear wheel is rotating at 24611 revolutions per minute. Find :
the revolutions per minute made by the front wheel.
View SolutionThe diameter of the front and the rear wheels of a tractor are 63 cm and 1.54 m respectively. The rear wheel is rotating at 24611 revolutions per minute. Find :
the distance travelled by the tractor in 40 minutes.
View SolutionThe wheel of a car is rotating at the rate of 1200 revolutions per minute. On pressing the accelerator for 10 seconds, it starts rotating at 4500 revolutions per minute. The angular acceleration of the wheel is
View SolutionFind the acceleration of a particle placed on the surface of the earth at the equator due to earth's rotation. The diameter of earth =12800 km and it takes 24 hour for the earth to complete one revolution about its axis.
View SolutionSuppose that the rotation of the earth was increased such that the centripetal acceleration was equal to the gravitational acceleration at the equator (i) Find the speed of a person standing at the equator and (ii) How long would a day be ?
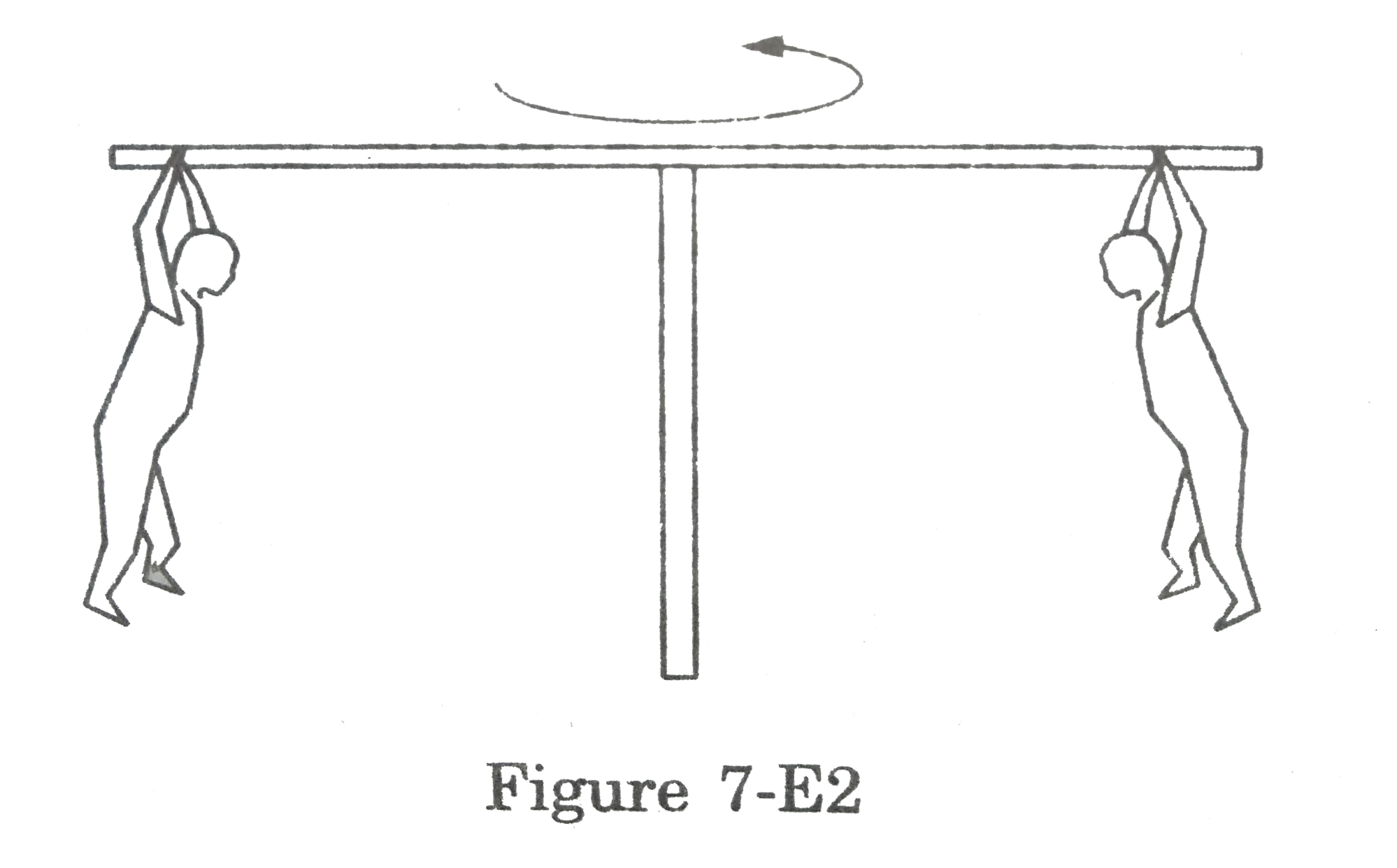
View SolutionIn a children's park a heavy rod is pivoted at the centre and is made to rotate about the pivot so that the rod always remains horizontal. Two kids hold the rod near the ends and thus rotate with the rod figure. Let the mas of each kid be 15 kg, the distance between the points of the rod where the two kids hold it be 3.0 m and suppose that the rod rotates at the rate of 20 revolutions per minute. Find the force of friction exerted by the rod on one of the kids.
View SolutionThe angular velocity of a particle moving in a circle of radius 50cm is increased in 5 min from 100 revolutions per minute to 400 revolutions per minute. Find the tangential acceleration of the particle.
View SolutionA particle is moving with speed v=2t2 on the circumference of a circle of radius R. Match the quantities given in Table-1 with corresponding results in Table-2
Table-1Table-2(A)Magnitude to tangential acceleration of particle(P)decreases with time(B)Magnitude of centripetal acceleration of particle(Q)increases with time(C)Magnitude of angular speed of particle with respect to centre of circle(R)remains constant(D)Angle between the total acceleration and centripetal acceleration of partilce(S)depends upon the value of radius R
View Solution
