Two vectors having equal magnitudes A make an angle θ with each other. Find the magnitude and direction of the resultant.
2Acosθ2, along bisector
Acosθ2,at45∘ from on a vector
2Acosθ2, along bisector
Acosθ2, along bisector
The correct Answer is:A
To find the magnitude and direction of the resultant of two vectors of equal magnitude A that make an angle θ with each other, we can follow these steps:
Step 1: Understand the Vectors
Let the two vectors be represented as →A1 and →A2, both having a magnitude A and an angle θ between them.
Step 2: Use the Law of Cosines
The magnitude of the resultant vector R can be calculated using the formula:
R=√A2+A2+2A⋅A⋅cos(θ)
This simplifies to:
R=√2A2(1+cos(θ))
Step 3: Simplify the Resultant Magnitude
Factoring out A2:
R=√2A2(1+cos(θ))=A√2(1+cos(θ))
Step 4: Use the Cosine Identity
Using the identity 1+cos(θ)=2cos2(θ2):
R=A√2⋅2cos2(θ2)=A⋅2⋅cos(θ2)=2Acos(θ2)
Step 5: Find the Direction of the Resultant
To find the direction of the resultant vector, we can use the formula for the angle α that the resultant makes with one of the vectors. The tangent of this angle can be given by:
tan(α)=Asin(θ)A+Acos(θ)=sin(θ)1+cos(θ)
Step 6: Use the Sine and Cosine Identity
Using the identity sin(θ)=2sin(θ2)cos(θ2) and 1+cos(θ)=2cos2(θ2):
tan(α)=2sin(θ2)cos(θ2)2cos2(θ2)=tan(θ2)
Step 7: Conclude the Direction
Thus, we find that:
α=θ2
Final Result
The magnitude of the resultant vector R is:
R=2Acos(θ2)
And the direction of the resultant vector is:
α=θ2
Let the two vectors be represented as →A1 and →A2, both having a magnitude A and an angle θ between them.
The magnitude of the resultant vector R can be calculated using the formula:
R=√A2+A2+2A⋅A⋅cos(θ)
This simplifies to:
R=√2A2(1+cos(θ))
Factoring out A2:
R=√2A2(1+cos(θ))=A√2(1+cos(θ))
Using the identity 1+cos(θ)=2cos2(θ2):
R=A√2⋅2cos2(θ2)=A⋅2⋅cos(θ2)=2Acos(θ2)
To find the direction of the resultant vector, we can use the formula for the angle α that the resultant makes with one of the vectors. The tangent of this angle can be given by:
tan(α)=Asin(θ)A+Acos(θ)=sin(θ)1+cos(θ)
Using the identity sin(θ)=2sin(θ2)cos(θ2) and 1+cos(θ)=2cos2(θ2):
tan(α)=2sin(θ2)cos(θ2)2cos2(θ2)=tan(θ2)
Thus, we find that:
α=θ2
The magnitude of the resultant vector R is:
R=2Acos(θ2)
And the direction of the resultant vector is:
α=θ2
Topper's Solved these Questions
Similar Questions
Two vectors having equal magnitudes A make an angle θ with eahc other. Find the magnitude and direction of the resultant.
View SolutionThe resultant of two vectors , having equal magnitudes E make an angle of 120∘ with each other . What is the magnitude and direction of the resultant ?
View SolutionFind the magnitude and direction of the resultant of given vectors
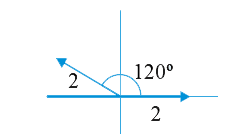
View SolutionTwo vectors having equal magnitude of 5 units, have an angle of 60∘ between them. Find the magnitude of their resultant vector and its angle from one of the vectors.

View SolutionTwo vectors 3 units and 5 units are acting at 60∘ to each other .What is the magnitude and direction of the resultant ?
View SolutionTwo vectors of equal magnitude of 5 unit have an angle 600 between them. Find the magnitude of (a) the sum of the vectors and (b) the difference of the vectors.
 .
.  .
.
View SolutionTwo vectors having magnitude 12 and 13 are inclined at and angle45∘ to each other.find their resultant vector.
View SolutionTwo vectors have magnitudes 6 and 8 units, respectively. Find the magnitude of the resultant vector if the angle between vectors is (a) 60∘ (b) 90∘ and ( c ) 120∘.
View SolutionTwo vectors of equal magnitude are acting through a point. The magnitude of resultant is equal to the magnitude of either vectoe. Find the angle between the vectors.
View SolutionTwo vectors of equal magnitude P are inclined at some angle such that the difference in magnitude of resultant and magnitude of either of the vectors is 0.732 times either of the magnitude of vectors. If the angle between them is increased by half of its initial value then find the magnitude of difference of the vectors
View Solution
