An AC source is connected to a capacitor. The current in the current is I. Now a dielectric slab is inserted into the capacitor , then the new current is
equal I
more than I
less than I
may be more than or less than I
The correct Answer is:B
To solve the problem step by step, we will analyze the effect of inserting a dielectric slab into a capacitor connected to an AC source.
1. Understanding Initial Conditions:
- We have an AC source connected to a capacitor with an initial capacitance C.
- The initial current flowing through the circuit is I.
2. Effect of Inserting a Dielectric:
- When a dielectric slab is inserted into the capacitor, the capacitance increases. The new capacitance C′ can be expressed as:
C′=K⋅C
where K is the dielectric constant of the material.
3. Capacitive Reactance:
- The capacitive reactance XC is given by the formula:
XC=1ωC
- Since the capacitance increases to C′, the new capacitive reactance X′C becomes:
X′C=1ωC′=1ω(K⋅C)=1K⋅1ωC=XCK
- This shows that X′C decreases because K>1.
4. Current in the Circuit:
- The current I in the circuit is related to the voltage V and the capacitive reactance XC by the formula:
I=VXC
- After inserting the dielectric, the new current I′ is given by:
I′=VX′C=VXCK=K⋅VXC=K⋅I
- Since K>1, it follows that I′>I.
5. Conclusion:
- Therefore, the new current I′ after inserting the dielectric slab is greater than the initial current I.
Final Answer:
The new current I′ is greater than the initial current I.
---
- We have an AC source connected to a capacitor with an initial capacitance C.
- The initial current flowing through the circuit is I.
- When a dielectric slab is inserted into the capacitor, the capacitance increases. The new capacitance C′ can be expressed as:
C′=K⋅C
where K is the dielectric constant of the material.
- The capacitive reactance XC is given by the formula:
XC=1ωC
- Since the capacitance increases to C′, the new capacitive reactance X′C becomes:
X′C=1ωC′=1ω(K⋅C)=1K⋅1ωC=XCK
- This shows that X′C decreases because K>1.
- The current I in the circuit is related to the voltage V and the capacitive reactance XC by the formula:
I=VXC
- After inserting the dielectric, the new current I′ is given by:
I′=VX′C=VXCK=K⋅VXC=K⋅I
- Since K>1, it follows that I′>I.
- Therefore, the new current I′ after inserting the dielectric slab is greater than the initial current I.
The new current I′ is greater than the initial current I.
Similar Questions
An AC source is connected to a capacitor. Will the rms current increase, decrease or remain constant if a dielectric slab is inserted into the capacitor?
View SolutionA capacitor and a bulb are connected in series with a source of alternating emf. If a dielectric slab is inserted between the plates of the capacitor, then
View SolutionA series RLC circuit is connected to an ac generator. The instant at which current in the circuit is zero, the energy stored in the capacitor and inductor are:
View SolutionTwo identical capacitor C1 and C2 are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of C2. The potential across C1 will :
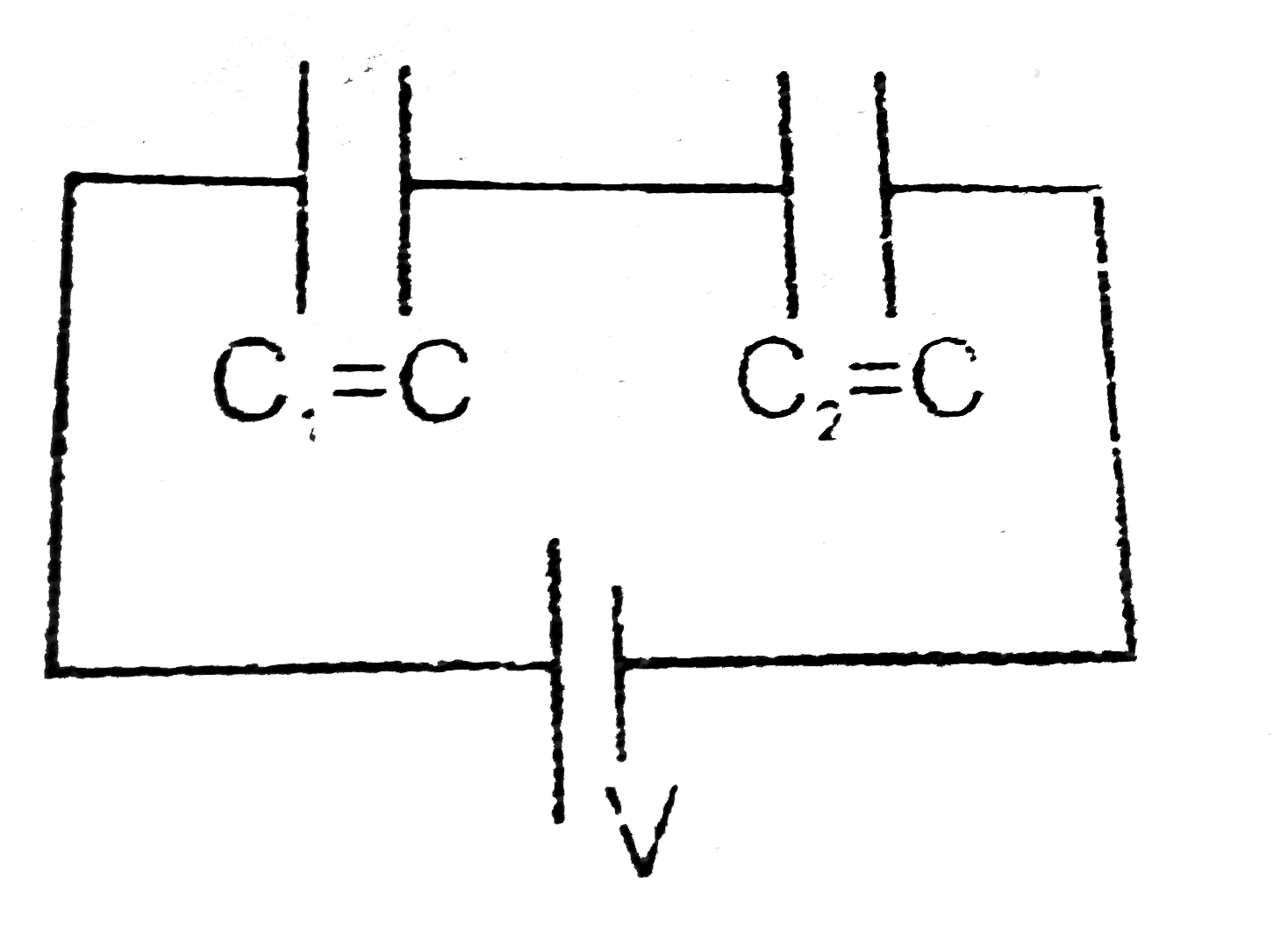
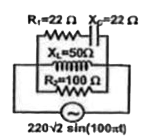
View SolutionThe current through the capacitor in the given circuit is
View SolutionWhen an AC source is connected to a capacitor there is a steady-state current in the circuit. Does it mean that the charges jump from one plate to the other to complete the circuit?
View SolutionAfter charging a capacitor the battery is removed. Now by placing a dielectric slab between the plates
View SolutionA parallel plate capacitor is connected across a battery. Now, keeping the battery connected, a dielectric slab is inserted between the plates. In the process,
View SolutionIf an ac source is connected across ideal capacitor and current passing through it is denoted by curve (a) then instantaneous power is denoted by curve
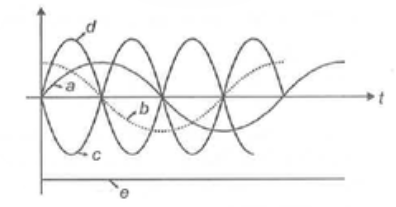
View SolutionA variable frequency AC source is connected to a capacitor. How will the displacement current change with decrease in frequency?
View Solution
