A 100Ω resistance and a capacitor of 100Ω reactance are connected in series across a 220 V source. When the capacitor is 50% charged, the peak value of the displacement current is
2.2 A
0.45833333333333
4.4 A
11√2 A
The correct Answer is:A
To solve the problem step by step, we need to find the peak value of the displacement current when a 100 Ω resistance and a capacitor with 100 Ω reactance are connected in series across a 220 V source.
Step 1: Calculate the peak voltage (V_max)
The given voltage is the RMS voltage (V_rms = 220 V). To find the peak voltage (V_max), we use the formula:
Vmax=Vrms×√2
Substituting the value:
Vmax=220×√2≈220×1.414≈311.13V
Step 2: Calculate the impedance (Z)
In a series circuit with resistance (R) and capacitive reactance (X_C), the total impedance (Z) is given by:
Z=√R2+X2C
Here, R = 100 Ω and X_C = 100 Ω. Thus,
Z=√1002+1002=√10000+10000=√20000=100√2Ω
Step 3: Calculate the peak current (I_max)
The peak current (I_max) can be calculated using Ohm's law for AC circuits:
Imax=VmaxZ
Substituting the values we found:
Imax=311.13100√2≈311.13141.42≈2.20A
Step 4: Conclusion
The peak value of the displacement current when the capacitor is 50% charged is approximately 2.20 A.
The given voltage is the RMS voltage (V_rms = 220 V). To find the peak voltage (V_max), we use the formula:
In a series circuit with resistance (R) and capacitive reactance (X_C), the total impedance (Z) is given by:
The peak current (I_max) can be calculated using Ohm's law for AC circuits:
The peak value of the displacement current when the capacitor is 50% charged is approximately 2.20 A.
Similar Questions
Knowledge Check
A 0.2kΩ resistor and 15μF capacitor are connected in series to a 220 V, 50 Hz ac source. The impadance of the circuit is
A250ΩB268ΩC29.15ΩD291.5Ω
Two bulbs 60 W and 100 W designed for voltage 220 V are connected in series across 220 V source. The net power dissipated is
View SolutionA 0.21 H inductor and a 12Ω resistance are connected in series to a 220 V, 50 Hz ac source.
the phase angle between the current and the source voltage is
View SolutionA 9/100(π) inductor and a 12Ω resistanace are connected in series to a 225 V, 50 Hz ac source. Calculate the current in the circuit and the phase angle between the current and the source voltage.
View SolutionA capacitor of capacitance 100μF and a coil of resistance 50Ω and inductance 0.5H are connected in series with a110V,50HzAC source. Find the rms value of the current.
View SolutionAn electric lamp of 100Ω, a toaster of resistance 50Ω and a water filter of resistance 500Ω are connected in parallel to a 220V source. What is the resistance of an electric iron connected to the same source that takes as much current as all three appliances and what is the current through it ?
View SolutionThe current in 1Ω resistance and charge stored in the capacitor are
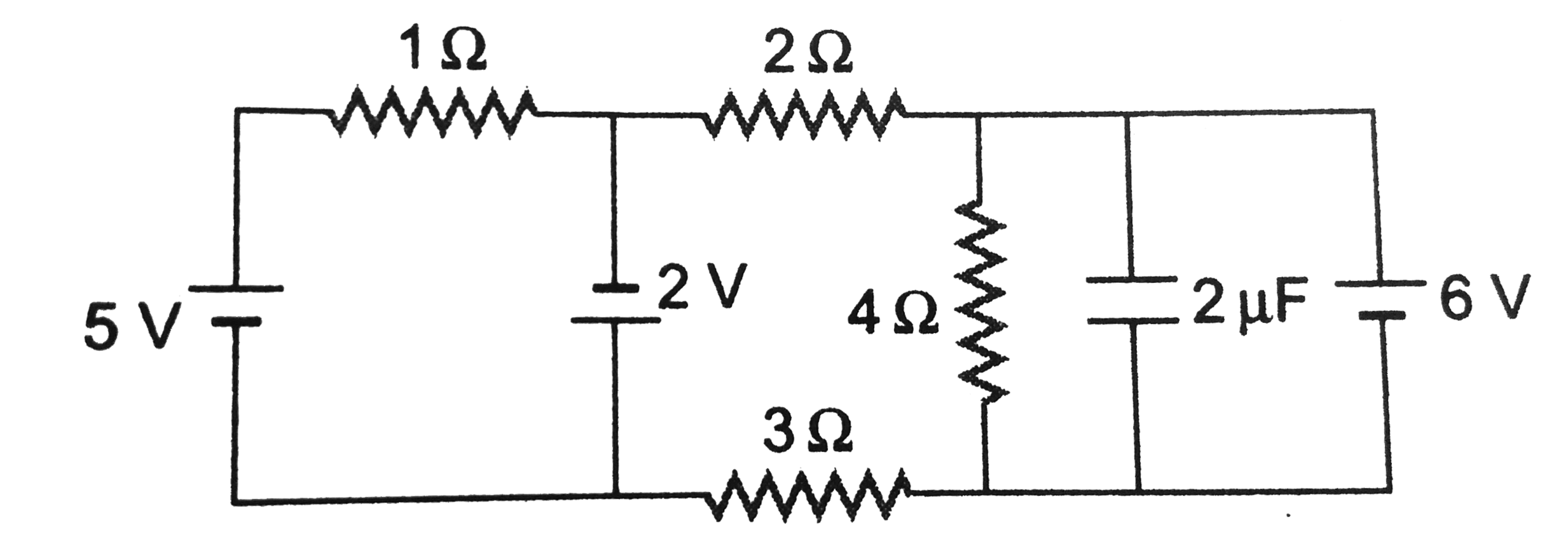
View SolutionA resistor of 200Ωand a capacitor of 15.0μF are connected in series to a 220V,50Hz source.
(a) Calculate the current in the circuit .
(b) Calcutalte the voltage (rms) across the resistor and the inductor. Is the algebraic sum of these voltages more than the source voltage? If yes, resolve the paradox.
View Solution
