A linear object of length 4 cm is placed at from the plane surface of hemispherical glass of radius 10 cm. The hemispherical glass is surrounded by water. Find the final position and size of the image.

For Ist surface,
μ1=43,μ2=32,u=−20cmandR=+10cm
Using, μ2v−μ1μ=(μ2−μ1)R
⇒(3/2)v−(4/3)(−20)=(3/2−4/3)10⇒v=−30cm
Using, A'B'AB=μ1vμ2u⇒A'B'(4cm)=(4/3)(−30)(3/2)(−20)
⇒A'B'=5.3cm
A' B' behaves as the object for plane surface,
μ1=32,μ2=43andR=∞,u'=−40
⇒μ1v'=μ2u'⇒(4/3)v'=(3/2)(−40)
Solving it, we will get, v'=-35.4 cm
Now, using ABA'B'=(μ1v')(μ2u')
AB(5.3)=(3/2)(−35.4)(4/3)(−40)
⇒A"B"=5.3 cm
The final images in all the above cases are shown in the figure.

Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.1
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.2
10 Videos
- NUCLEIBOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
Similar Questions
Knowledge Check
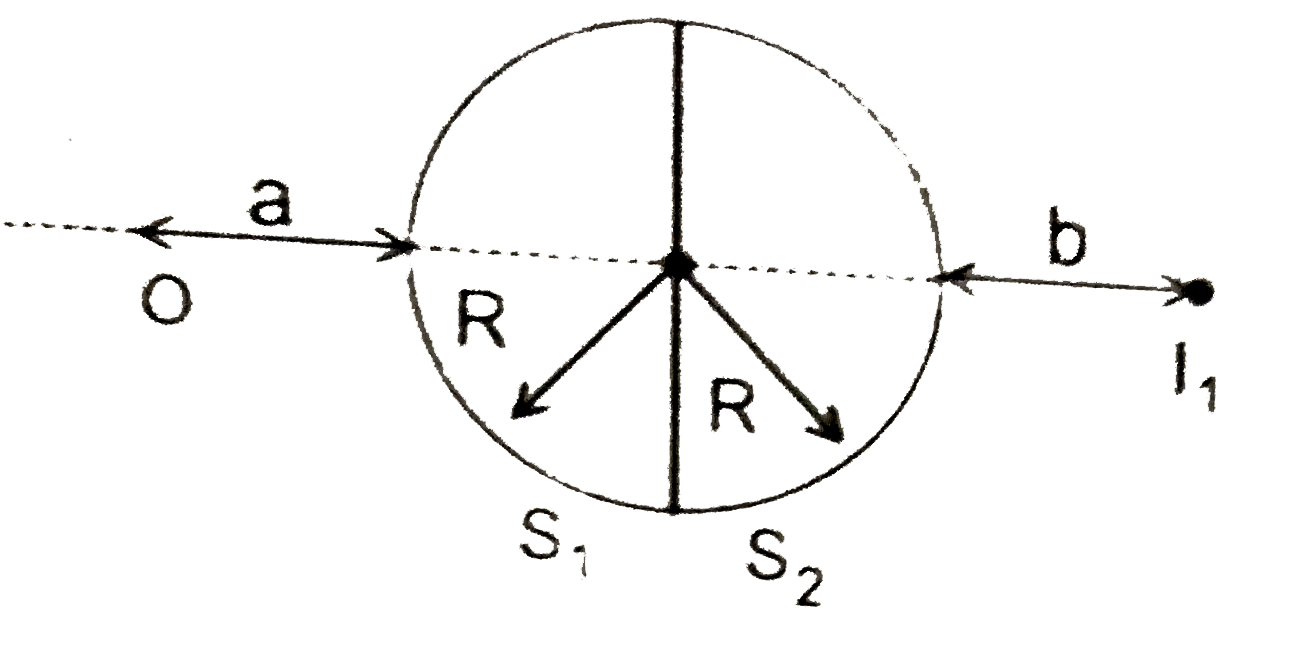
An object O kept a distance a=10cm from the surface of a glass hemisphere S1 of radius R. S2 is another glass hemisphere kept side by side of same radius R=5cm as shown in figure l1 is the image formed due to object O (b=6cm). Now if S2 is removed and S1 is kept at the same place and its flat surface is silvered then find te distance l1l2 (where l1 si image formed by silvered S1 only) (μ glass =3/2)A10 cmB12 cmC11cmD22 cm
Find the total surface area of a hemisphere of radius 10 cm
View SolutionFind the total surface area of a hemisphere of radius 10 cm
View SolutionA small object of height 0.5 cm is placed in front of a convex surface of glass (μ−1.5) of radius of curvature 10cm. Find the height of the image formed in glass.
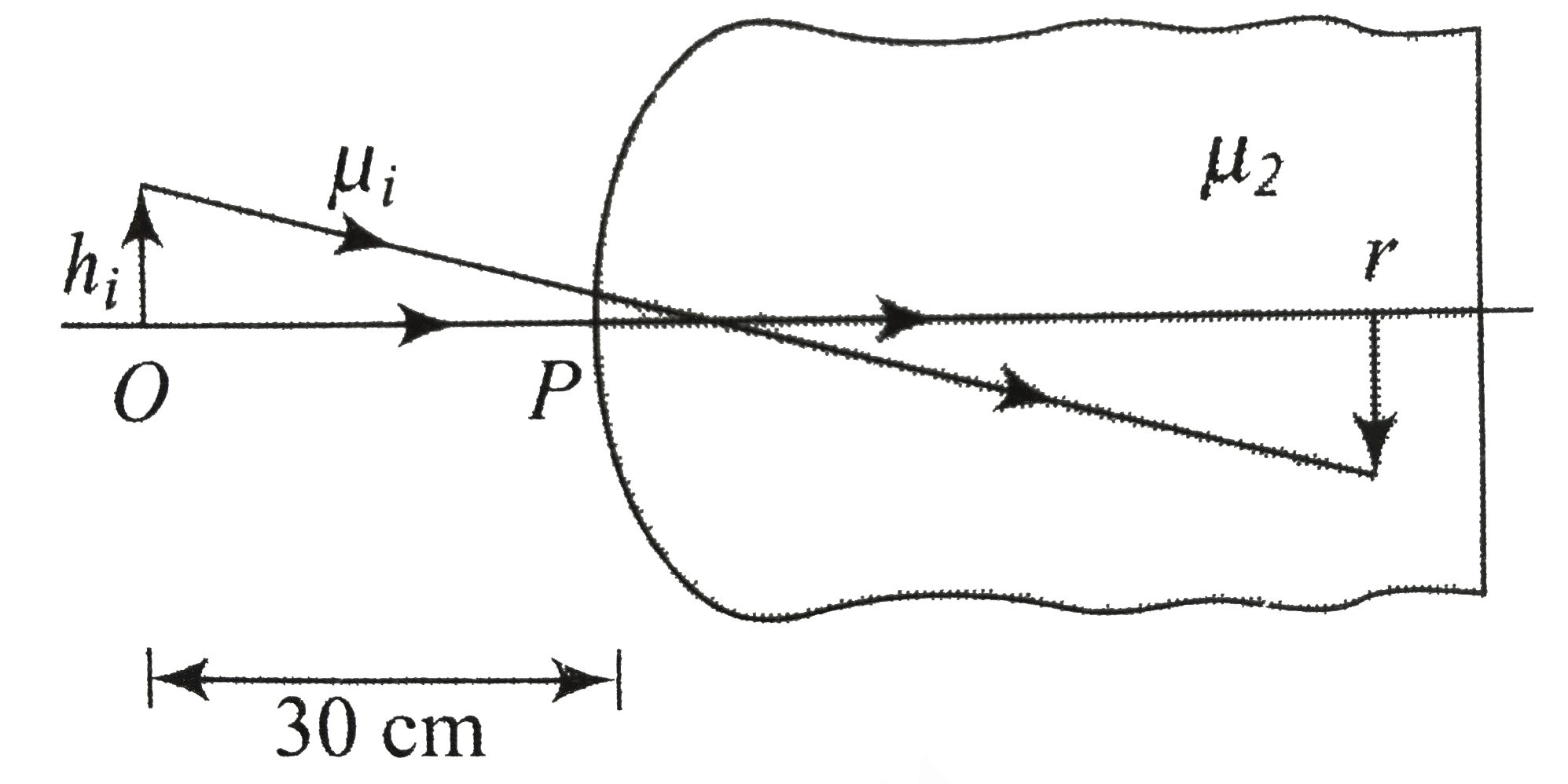
View SolutionA small object of height 0.5 cm is placed in front of a convex surface of glass (μ−1.5) of radius of curvature 10cm. Find the height of the image formed in glass.

View SolutionA 2.0 cm tall object is placed 40 cm from a diverging lens of focal length 15 cm. Find the position and size of the image.
View Solution
An object O kept a distance a=10cm from the surface of a glass hemisphere S1 of radius R. S2 is another glass hemisphere kept side by side of same radius R=5cm as shown in figure l1 is the image formed due to object O (b=6cm). Now if S2 is removed and S1 is kept at the same place and its flat surface is silvered then find te distance l1l2 (where l1 si image formed by silvered S1 only) (μ glass =3/2)
View SolutionA 0.5 cm high object is placed at 30 cm from a convex mirror whose focal length is 20 cm. Find the position and size of the image.
View Solution
