The focal length of a thin convex lens for red and blue colours is 100.5 cm and 99.5 cm .The dispersive power of the lens is
0.01
0.02
1.005
0.995
The correct Answer is:A
To find the dispersive power of the lens, we will follow these steps:
Step 1: Understand the formula for dispersive power
The dispersive power (Ω) of a lens is given by the formula:
Ω=fred−fbluefyellow
where:
- fred is the focal length for red light,
- fblue is the focal length for blue light,
- fyellow is the focal length for yellow light.
Step 2: Calculate the focal length for yellow light
The focal length for yellow light can be calculated using the formula:
fyellow=√fred⋅fblue
Given:
- fred=100.5cm
- fblue=99.5cm
Substituting the values:
fyellow=√100.5×99.5
Step 3: Perform the calculation for fyellow
Calculating fyellow:
fyellow=√100.5×99.5=√10000.25≈100.00125cm
Step 4: Substitute the values into the dispersive power formula
Now we can substitute the values into the dispersive power formula:
Ω=100.5−99.5100.00125
Step 5: Perform the subtraction and division
Calculating the numerator:
100.5−99.5=1.0
Now substituting this back into the dispersive power formula:
Ω=1.0100.00125≈0.009999875
Step 6: Round the answer
Rounding to two decimal places, we get:
Ω≈0.01
Final Answer
The dispersive power of the lens is approximately 0.01.
---
The dispersive power (Ω) of a lens is given by the formula:
Ω=fred−fbluefyellow
where:
- fred is the focal length for red light,
- fblue is the focal length for blue light,
- fyellow is the focal length for yellow light.
The focal length for yellow light can be calculated using the formula:
fyellow=√fred⋅fblue
Given:
- fred=100.5cm
- fblue=99.5cm
fyellow=√100.5×99.5
Calculating fyellow:
fyellow=√100.5×99.5=√10000.25≈100.00125cm
Now we can substitute the values into the dispersive power formula:
Ω=100.5−99.5100.00125
Calculating the numerator:
100.5−99.5=1.0
Ω=1.0100.00125≈0.009999875
Rounding to two decimal places, we get:
Ω≈0.01
The dispersive power of the lens is approximately 0.01.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - medical entrance special format question23videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Medical entrance gallary76videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.710videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY46videos
NUCLEI
- BOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective9videos
REFLECTION OF LIGHT
Similar Questions
The focal lengths of a thin lens for red and violet light are 90.0 cm and 86.4cm respectively. Find the dispersive power of the material of the lens. Make appropriate assumptions.
View SolutionThe focal lengths of a convex lens for red, yellow and violet rays are 100 cm, 98 cm and 96 cm respectively. Find the dispersive power of the material of the lens.
View SolutionAn achromatic lens-doublet is formed by placing in contact a convex lens of focal length 20cm and a concave lens of focal length 30cm. The dispersive power of the material of the convex lens is 0.8. Determine the dispersive power of the material of the concave lens. Calculate the focal length of the lens-doublet.
View SolutionCalculate the focal length of a convex lens whose radii of curvature of two surfaces is 10cm and 15cm respectively and its refractive index is 1.5.
View SolutionThe focal length of objective and eye lens of a microscope are 4cm and 8cm respectively. If the least distance of distinct vision is 24cm and object distance is 4.5cm from the objective lens, then the magnifying power of the microscope will be
View SolutionThe focal length of objective and eye lens of a microscope are 4 cm and 8 cm respectively. If the least distance of distinct vision is 24 cm and object distance is 4.5 cm from the objective lens, then the magnifying power of the microscope will be
View SolutionAn achromatic doublet of focal length 90 cm is to be made of two lenses, the material one having 1.5 times the dispersive power of the other. The doublet is converging type. Find the focal length of each of lens.
View SolutionThe focal length of a thin convex-lens is 30cm. At a distance of 10 cm from the lens there is a plane refracting surface fo refractive inidex 3/2 Where will parallel rays incident on lens converge?
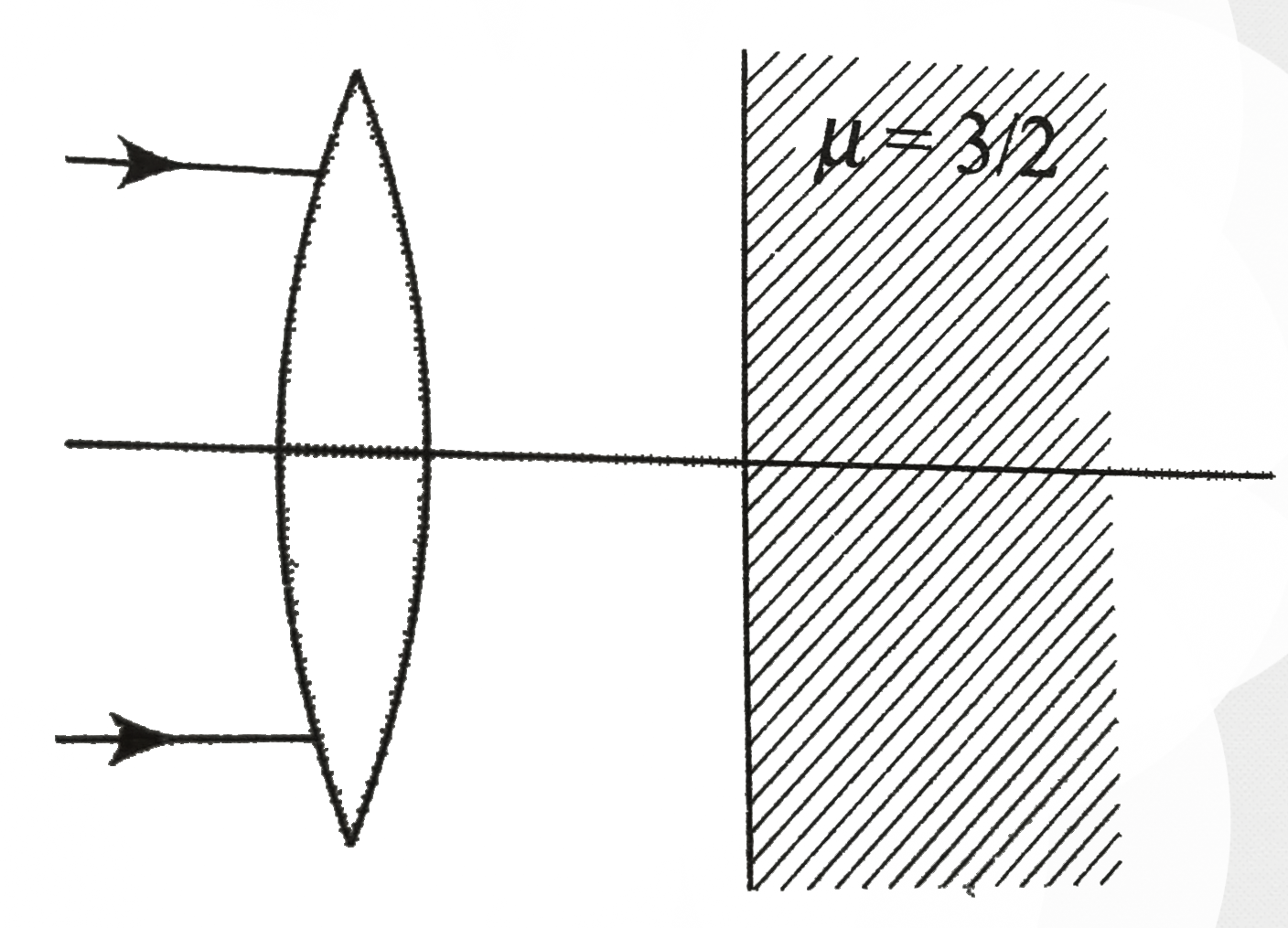 View Solution
View SolutionAssertion:A convex lens and a concave lens are kept in contact. They will behave as a diverging lens if focal length of convex lens is more.
Reason: Power of a concave lens is always less than the power of a convex lens, as power of concave lens is negative whereas power of convex lens is positive.View SolutionThe focal lengths of the objective and eye− lens of a microscope are 1cm and 5cm respectively. If the magnifying power for the relaxed eye is 45, then the length of the tube is
View Solution

