If light travels a distance x in t1 sec in air and 10x distance in t2 sec in a medium, the critical angle of the medium will be
The correct Answer is:C
To find the critical angle of the medium given the distances and times light travels in air and in the medium, we can follow these steps:
Step 1: Calculate the velocities of light in both media
The velocity of light in air (v1) can be calculated using the formula:
v1=xt1
The velocity of light in the medium (v2) can be calculated as:
v2=10xt2
Step 2: Use Snell's Law to relate the refractive indices
According to Snell's Law:
μ2μ1=v1v2
Where:
- μ1 is the refractive index of air, which is approximately 1.
- μ2 is the refractive index of the medium.
Step 3: Substitute the velocities into Snell's Law
Substituting the expressions for v1 and v2 into Snell's Law gives:
μ21=xt110xt2
This simplifies to:
μ2=t210t1
Step 4: Relate the critical angle to the refractive indices
The critical angle (C) can be found using the formula:
sinC=μ1μ2
Since μ1=1 (for air), we have:
sinC=1μ2
Substituting the expression for μ2:
sinC=1t210t1=10t1t2
Step 5: Find the critical angle
To find the critical angle, we take the inverse sine:
C=sin−1(10t1t2)
Final Answer
Thus, the critical angle of the medium is:
C=sin−1(10t1t2)
---
The velocity of light in air (v1) can be calculated using the formula:
v1=xt1
The velocity of light in the medium (v2) can be calculated as:
v2=10xt2
According to Snell's Law:
μ2μ1=v1v2
Where:
- μ1 is the refractive index of air, which is approximately 1.
- μ2 is the refractive index of the medium.
Substituting the expressions for v1 and v2 into Snell's Law gives:
μ21=xt110xt2
This simplifies to:
μ2=t210t1
The critical angle (C) can be found using the formula:
sinC=μ1μ2
Since μ1=1 (for air), we have:
sinC=1μ2
Substituting the expression for μ2:
sinC=1t210t1=10t1t2
To find the critical angle, we take the inverse sine:
C=sin−1(10t1t2)
Thus, the critical angle of the medium is:
C=sin−1(10t1t2)
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - medical entrance special format question
23 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Medical entrance gallary
76 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.7
10 Videos
- NUCLEIBOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
Similar Questions
Light takes t1 second to travel a distance x cm in vacuum and the same light takes t2 second to travel 10x cm in medium. The critical angle for the corresponding medium is
View SolutionIn vacum, to travel distance d, light takes time t and in medium to travel distance 5d, it takes time T. The critical angle of the medium is
View SolutionCalculate the speed of light in a medium whose critical angle is 30∘.
View SolutionWavelength of given light waves in air and in a medium are 6000 Å and 4000 Å respectively . The critical angle for the medium is given by
View SolutionWhen light is incident on a medium at angle i and refracted into a second medium at an angle r, the graph of sinr versus sini is as shown. From this one can conclude that

(i) the velocity of light in second medium is √3 times the velocity of light in the first medium
(ii) the velocity of light in the first medium is √3 times the velocity of ligth in second medium
(iii) the critical angle of the two media is given by siniC=1√3
(iv) the critical anlge of the two media is given by siniC=1√2
View SolutionA ray of light travels from an optically denser to rarer medium. The critical angle of the two media is C. The maximum possible deviation of the ray will be
View SolutionLight propagates 2 cm distance in glass of refractive index 1.5 in time t0. In the same time t0, light propagates a distance of 2.25 cm in medium. The refractive index of the medium is
View SolutionIf light travels a distance of 5 m in a medium of refractive index μ, then equivalent path in vaccum it would travel in same time is
View SolutionIn the given v-t graph, the distance travelled by the body in 5 sec will be :
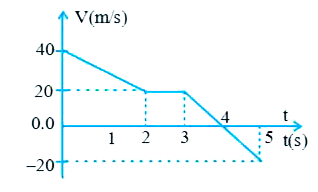
View SolutionWavelength of given light waves in air and in a medium are 6000 Å and 3000 Å, respectively. The critical angle is
View Solution
