The correctness of equation can be checked using the principle of homogeneity in dimensions.Using this principle, check whether the equation f=2π√lg is dimensionally correct, where f - frequency, I - length and g - acceleration due to gravity.
[f]=[M0L0T−1],[L]=[M0LT0],[g]=[M0LT−2] since [f]≠[√lg], the equation is wrong.
Similar Questions
Using dimensional analysis check the correctness of the equation T=2π√lg, where T is the period of the simple pendulum of length l and g is the acceleration due to gravity.
View Solution(A) The correctness of an equation is verified using the principle of homogeneity
(B) All unit less quantities are dimensional less.View Solution
Knowledge Check
- Question 1 - Select One
Using the principle of homogeneity of dimensions, which of the following is correct?
AT2=4π2r3GMBT2=4π2r2CT2=4π2r3GDT=4π2r3G - Question 2 - Select One
Using the principle of homogeneity of dimensions, which of the following is correct?
AT2=4π2r3GMBT2=4π2r2CT2=4π2r3GDT=4π2r3G - Question 3 - Select One
Whether a given relation / formula is correct or not can be checked on the basis of the principle of homogeneity of dimensions. According to this principle, only that formula is correct, in which the dimensions of the various terms on one side of the relation are equal to the respective dimensions of these terms on the other side of the relation. With the help of the comprehension given above, choose the most appropriate alternative for each of the following questions :
In the same equation, the dimensional formula of a isA[M−1L0T2]B[ML0T2]C[ML−1T−2]D[M−1L1T−2]
Consider the following arrangement and choose the correct options
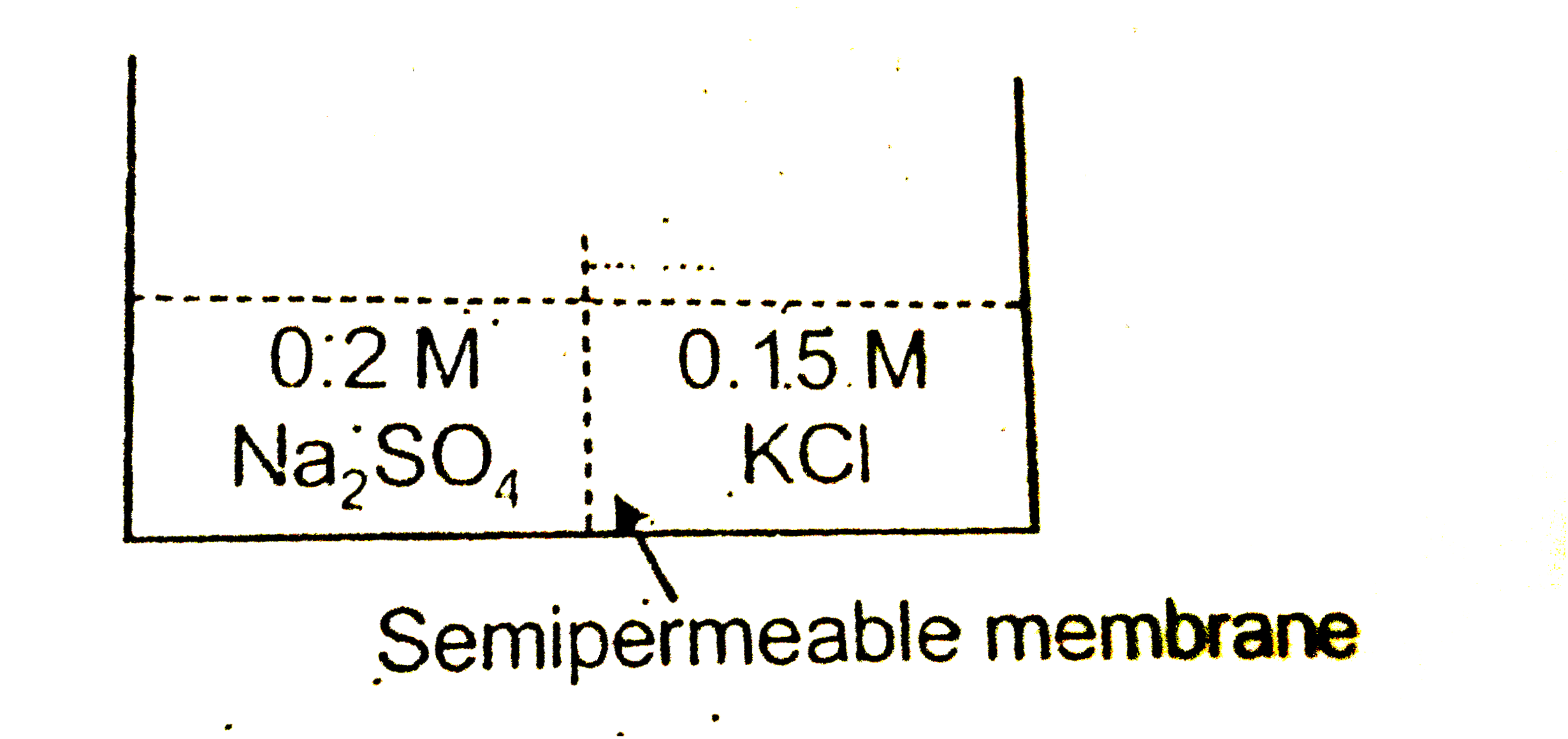 View Solution
View SolutionState the principle of homogeneity of dimensions.Test the dimensional homogeneity of the following equations:- h=h0+v0⋅t+12g⋅t2
View SolutionConsider the equation T=2π√lg and check whether it is correct or not.
View SolutionUsing the dimensional analysis, check whether the following equation is correct or not: T=2π√R3/GM.
View SolutionWhether a given relation / formula is correct or not can be checked on the basis of the principle of homogeneity of dimensions. According to this principle, only that formula is correct, in which the dimensions of the various terms on one side of the relation are equal to the respective dimensions of these terms on the other side of the relation. With the help of the compreshension given above, choose the most appropriate alternative for each of the following questions :
The distance travelledl by a body in nth second is given by Snth=u+a2(2n−1) where u is initial velocity and a is acceleration. The dimensions of Snth areView Solution
