If a random variable X has a binomial distribution B (8,12) then find X for which the outcome is the most likely.Solution in Odia
Given binomial distribution is B (8.12)
Thus n=8,p=12,q=12
We shall find X which is most likely i.e.for which p(x-r) is maximum where r=0,1,2,.......,8
As p=q, the probability is maximum when ^8Cr is maximum
But ^8Cr,r=0,1,2,....... 8 is maximum when r=4.
Thus the most likely outcome is x=4.
Topper's Solved these Questions
Similar Questions
Prove the following by induction.
1 + 2+ 3+….+ n = n(n+1)/2
View SolutionIf A-{a,b,c,d) mention the type of relations on A given below, which of them are equivalence relations?
{(a, a),(b,b)}
View SolutionThe probability distribution of the random variables X is given by
X1234P(X=x)1/81/21/81/4
Then the value of V(X) is equal to
View SolutionA random variable X has the distribution.
Then , variance of the distribution, is
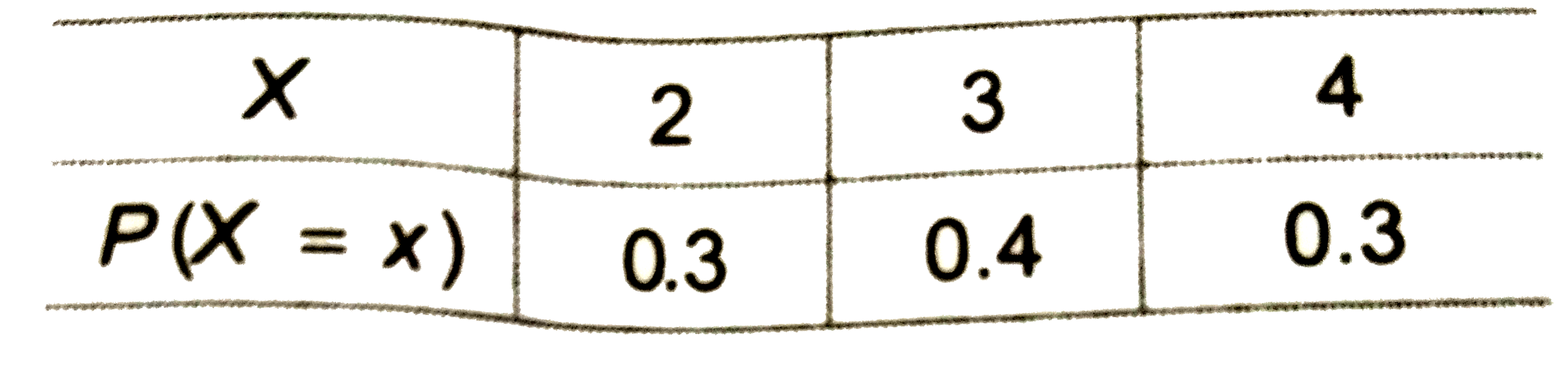
View Solutionकिसी यदृच्छया चर x का माध्य तथा विचलन जिसका द्वोपद वितरण है , क्रमश: 4 तथा 2 है तब P(X=1) होगा :
View Solutionयदि यादृच्छिक चर X के संगत प्रायिकता बंटन B(5,12) द्विपद बंटन है। किस यादृच्छिक चर के संगत न्यूनतम और अधिकतम प्रायिकता है।
View Solutionsuppose X has a binomial distribution B(6,12) . Show that X = 3 is the most likely outcome.
View SolutionSuppose X has binomial distribution B(6,12). Show that X = 3 is the most likely outcome.
View SolutionIf a random variable X has a binomial distribution B(8,12), then find X for which the outcome is most likely.
View SolutionA random variable X has the following probability distribution find mean of x:
View SolutionSuppose X has a binomial distribution B(6,1/2) show that X=3 is the most likely outcome.
View SolutionA random variable X has the distribution.
Then , variance of the distribution, is
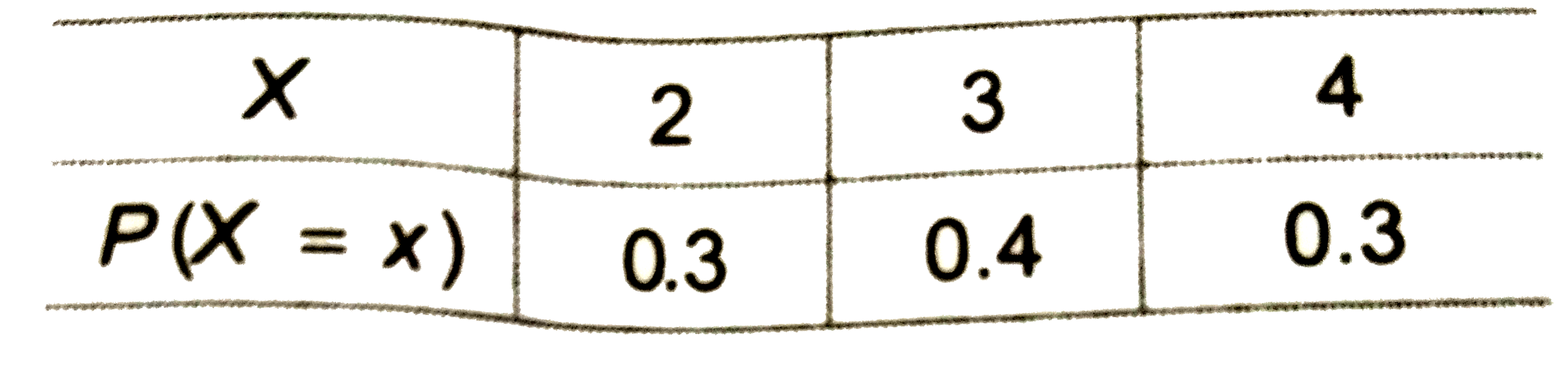
View SolutionSuppose x has a binomial distribution B(6,12) show that x =3 is the most likely outcome
View SolutionSuppose x has a binomial distribution B(6,12) show that x =3 is the most likely outcome
View SolutionIf a random variable X has a binomial distribution B (8,12) then find X for which the outcome is the most likely.
View Solution
