If ∠BAC=120∘ then measure of ∠RPQ will be
60∘
90∘
120∘
150∘
The correct Answer is:B
Topper's Solved these Questions
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL II) (PRACTICE SHEET (ADVANCED) Straight Objective Type Questions)
10 Videos
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL II) (More than one correct answer Type Questions)
7 Videos
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL II) (LECTURE SHEET (ADVANCED)) (More than one correct answer Type Questions)
19 Videos
- PLANESBOOK - AAKASH SERIESCHAPTER - PLANESEXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS
30 Videos
- PROPERTIES OF VECTORSBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF VECTORSEXERCISE - PRACTICE EXERCISES
55 Videos
Similar Questions
In ΔABC,P,Q,R are the feet of angle bisectors from the vertices to their opposite sides as shown in the figure. ΔPQR is constructed

If ∠BAC=120∘, then measusred of ∠RPQ will be
View SolutionIn the figure, □ABCD is a cyclic quadrilateral. seg AB is a diameter. If ∠ADC=120∘, find the measure of ∠BAC.
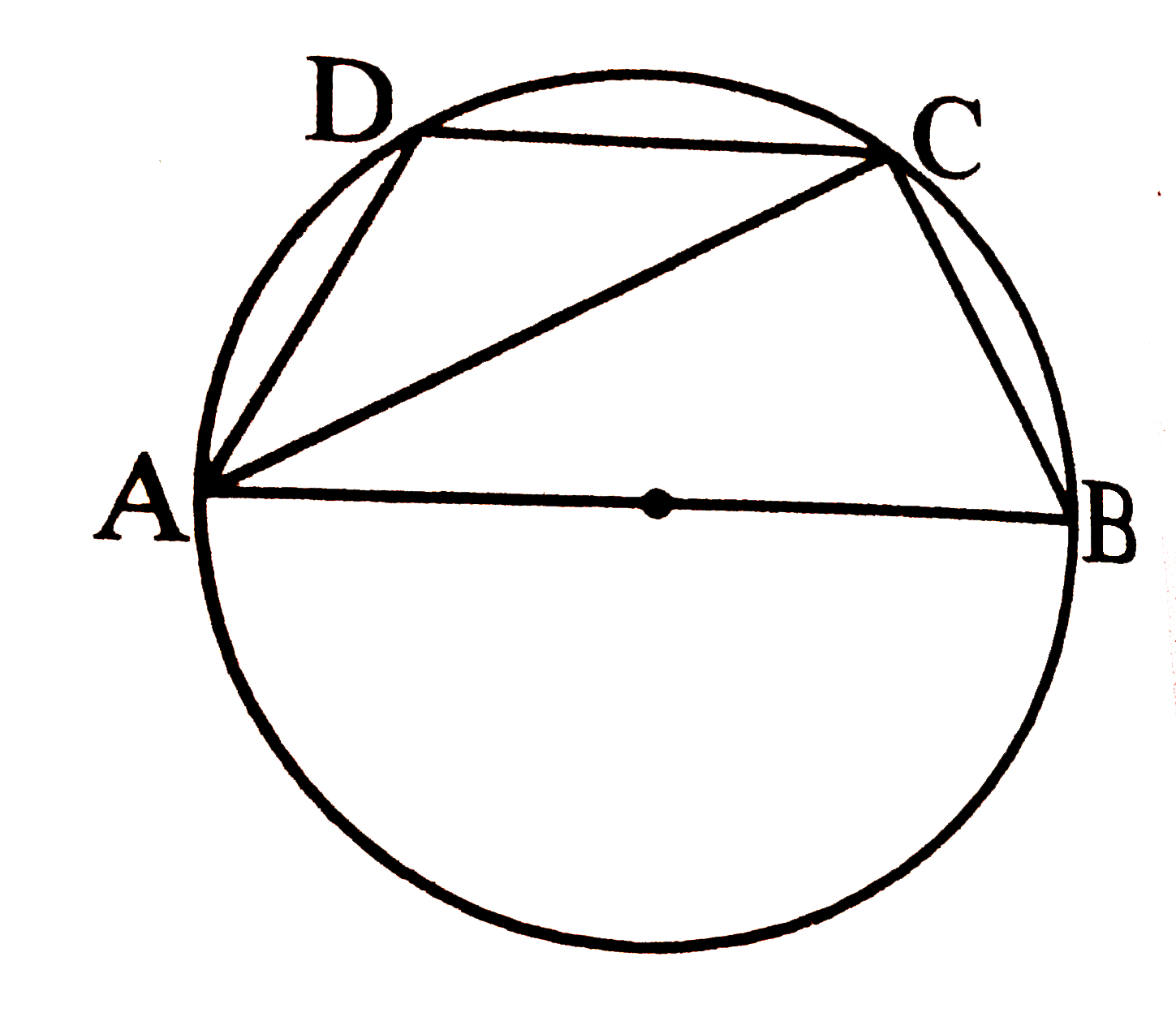
View Solution
Knowledge Check
In ΔABC,P,Q,R are the feet of angle bisectors from the vertices to their opposite sides as shown in the figure. ΔPQR is constructed

If ∠BAC=120∘, then measusred of ∠RPQ will beA60∘B90∘C120∘D150∘ABCD is a cyclic quadrilateral whose vertices are equidistant from the point O (centre of the circle). If ∠COD=120∘ and ∠BAC=30∘, then the measure of ∠BCD is :
A180∘B150∘C60∘D90∘In the triangle ABC,BC=CDand(∠ABC−∠BAC)=30∘. The measure of ∠ABD is :
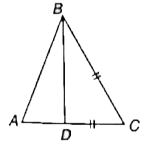 A30∘B45∘C15∘Dcan't be determined
A30∘B45∘C15∘Dcan't be determined
If O is the orthocentre of a triangle ABC and ∠BOC=100∘, the measure of ∠BAC is
View Solution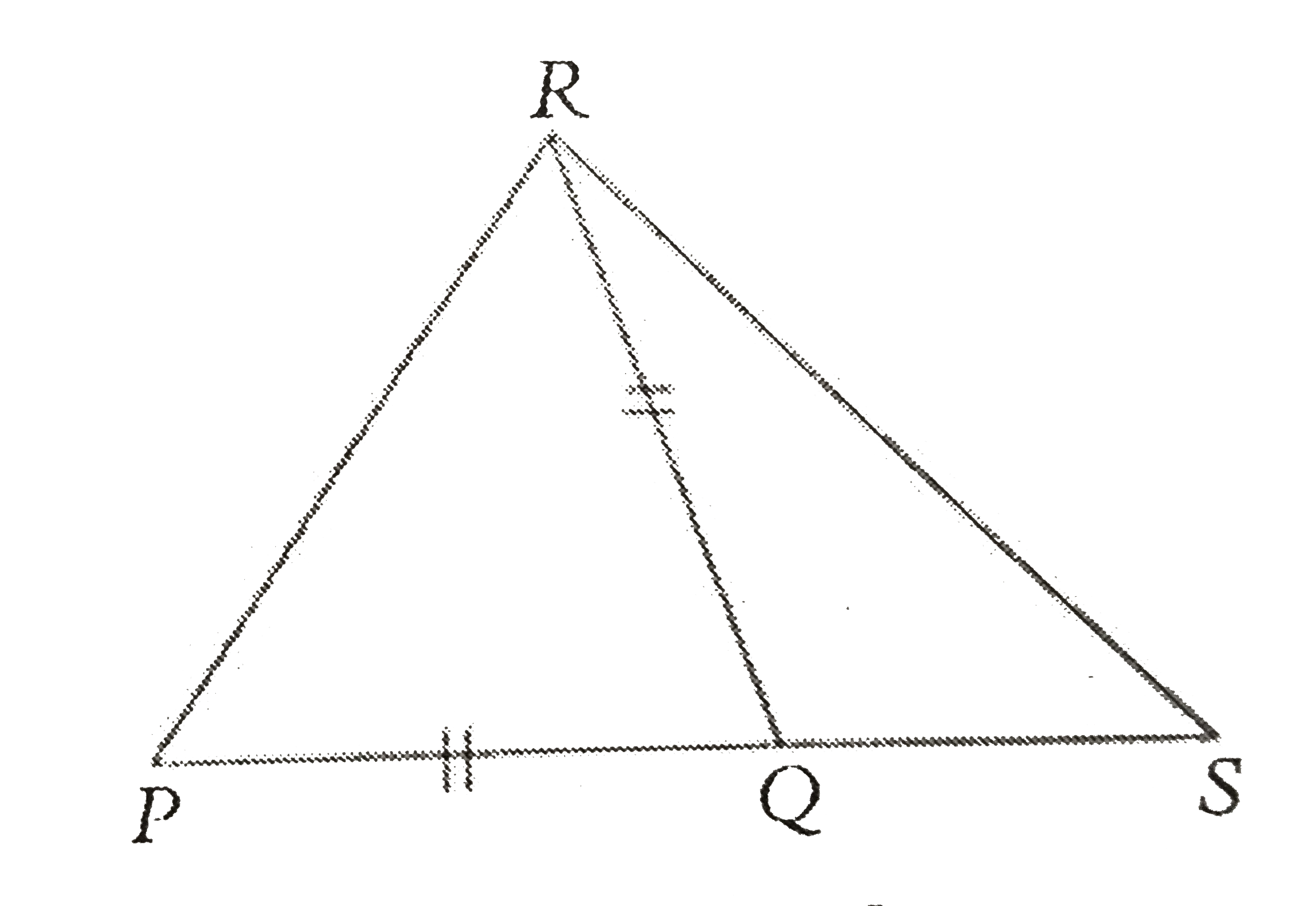
In the figure, PQ=QR,∠RPQ=60∘,and∠QRS=20∘. Find the measure of ∠QSR.
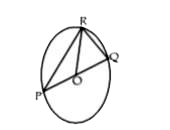
View SolutionIn the given figure, 0 is the centre of the circle and ∠QOR=50∘, then what is the value of ∠RPQ (in degrees)?
View SolutionThe circumentre of a triangle ABC is O. If <BAC−70∘and∠BAC−80∘, then the measure of ∠OAC is equal to :
View SolutionABCD is a cyclic quadrilateral whose vertices are equidistant from the point O (centre of the circle). If ∠COD=120∘ and ∠BAC=30∘, then the measure of ∠BCD is
View Solution
