यदि f(x)+f(1−x)=2 तथा g(x)=f(x)−1∀x∈R हो तो g(x) सम्मित है -
मूल बिन्दु के सापेक्ष
रेखा x=12 के सापेक्ष
बिन्दु (1,0) के सापेक्ष
बिन्दु (12,0) के सापेक्ष
The correct Answer is:D
Step by step video, text & image solution for यदि f(x)+f(1-x)=2 तथा g(x)=f(x)-1 AA x in R हो तो g(x) सम्मित है - by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
माना कि g:R→R,g(x)=3+4x द्वारा दिया जाता है यदि gn(x)=gogo…….og(x), तब g−n(x) बराबर है, जहाँ g−n(x),gn(x) का प्रतिलोम है-
02:42View Solutionफलन f1(x)=⎧⎪⎨⎪⎩1यदिx≤0x2+1यदि0<x<25यदिx≥2,f2(x)=log1/2(x−3),f3(x)=23−x तथा f4(x)=e{x} जहाँ {.} भिन्नात्मक भागफलन को प्रदर्शित करता है, के आरेख निम्न (क्रम में नहीं) है-
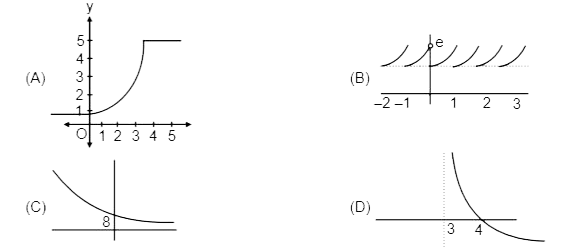
फलन f1(x),f2(x),f3(x) एवं f4(x) के आरेखों का सही क्रम होगा-06:52View SolutionLet f and g be differentiable functions such that: xg(f(x))f'(g(x))g'(x)=f(g(x))g'(f(x))f'(x)∀x∈R Also, f(x)>0 and g(x)>0∀x∈R∫x0f(g(t))dt=1−e−2x2,∀x∈R and g(f(0))=1,h(x)=g(f(x))f(g(x))∀x∈RNow answer the question:f(g(0))+g(f(0))= (A) 1 (B) 2 (C) 3 (D) 4
06:32View SolutionSuppose f and g are differentiabel functions such that xg(f(x))f'(g(x))g'(x)=(g(x))g'(f(x))f'(x)∀x∈Randf is positive ∀n∈R. Also ∫x0f(g(t))dt=12(1−e−2x)∀x∈R,g(f(0))=1andh(x)=g(f(x))f(g(x))∀x∈R.
The graph of y=h(x) is symmetric with respect to line:04:24View SolutionSuppose f and g are differentiabel functions such that xg(f(x))f'(g(x))g'(x)=(g(x))g'(f(x))f'(x)∀x∈Randf is positive ∀n∈R. Also ∫x0f(g(t))dt=12(1−e−2x)∀x∈R,g(f(0))=1andh(x)=g(f(x))f(g(x))∀x∈R.
The largest possible value of h(x)∀x∈R is:01:56View Solutionयदि f(x)=x1+|x|,∀x∈R तथा g(x)=x1−|x|,∀x∈R जहाँ −1<x<1 तो gof तथा fog ज्ञात कीजिये दिखाइए कि fog=gof
01:27View Solutionयदि f:R→R तथा g:R→R निम्न प्रकार परिभाषित हैं कि f(x) = 2x - 3 तथा g(x)=x2+5 , तो g(g(x)) ज्ञात कीजिए |
02:07View Solutionयदि f:x→3x−2 तथा g:x→x5∀x∈R , तो ज्ञात कीजिए : f(g(x) + 3)
03:13View Solutionयदि f:x→3x−2 तथा g:x→x5∀x∈R , तो ज्ञात कीजिए : f(g(x) - f(x) )
01:52View Solutionयदि फलन f तथा g निम्न प्रकार परिभाषित हो, तो fog तथा gof ज्ञात कीजिए:
f(x)=logx,g(x)=ex02:00View Solutionयदि फलन f तथा g निम्न प्रकार परिभाषित हो, तो fog तथा gof ज्ञात कीजिए:
f(x)=x3,g(x)=sinx01:58View Solutionयदि फलन f तथा g निम्न प्रकार परिभाषित हो, तो fog तथा gof ज्ञात कीजिए:
f(x)=cosx,g(x)=|sinx|04:51View Solutionयदि फलन f तथा g निम्न प्रकार परिभाषित हो, तो fog तथा gof ज्ञात कीजिए:
f(x)=ex,g(x)=x+2View Solutionयदि f(x)=xयदिx परिमेय है 0यदिx अपरिमेय है तथा g(x)=0यदिx परिमेय हैx यदिx अपरिमेय है तबf−g है
03:38View SolutionLet f(x)=|x−2|∀x∈R and g(x)=f(f(f(x))), then the number of solutions of g(x)=32 is
04:51View SolutionIf f:R→R and g:R→R are defined by f(x)=x−[x] and g(x)=[x]∀x∈R,f(g(x))=
02:57View Solution

