If x1,x2,x3,x4,x5 and x6 are the 6 terms between 3 and 24, find the common difference of the corresponding A.P.Solution in Malayalam
3
Topper's Solved these Questions
Similar Questions
If (x3+1,y−23)=(53,13), find the values
of x and y.
View SolutionConsider the set {x : x=2n ,n is a natural number, n≤5} Write this set in roster form.
View SolutionConsider the system of equations x1+x22+x33+x44+x55=5 and x1+2x2+3x3+4x4+5x5=15 where x1,x2,x3,x4,x5 are positive real numbers. Then numbers of (x1,x2,x3,x4,x5) is ___________.
View SolutionIf x1,x2,x3,x4,x5,x6 all are independent then the maximum and minimum values of [sin−1x1]+[cos−1x2]+[tan−1x3]+[cot−1x4]+[sec−1x5]+[cosec−1x6], where [] represents greatest integer function, respectively are
View SolutionIF A=[−1342],B=[213−5],X=[x1x2x3x4] and A+B=X, the find the values of x1,x2,x3,x4.
View SolutionA=[−1342],B=[213−5] మరియు X=[x1x2x3x4],A+B=X అయితే x1,x2,x3,x4 ల విలువలు కనుక్కోండి.
View SolutionIf mean and standard deviation of 5 observations x1,x2,x3,x4 are 10 and 3 respectively , then the variance of 6 observation x1,x2,…..x5and−50 is equal to:
View SolutionConsider the system of equations x1+x22+x33+x44+x55=5 and x1+2x2+3x3+4x4+5x5=15 where x1,x2,x3,x4,x5 are positive real numbers. Then numbers of (x1,x2,x3,x4,x5) is ___________.
View SolutionIf the terms of the arithmetic sequence 29,39,49,59.. are represented as x1,x2,x3.. then x4+x5+x6=.....
View SolutionThe following functions are defined for the set of variables x1,x2,x3,.......,xn
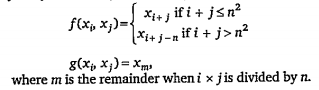
Find the value of f(f(x2,x3),f(x5,x6)) if n=3
View SolutionMean of the data x_1 , x_2 , x_3 , x_4 , x_5 is 15. If each observation is multiplied 3, then the mean becomes
View SolutionThe number of solutions to the system of equations x1+x2+x3+x4+x5=20 and x1+x2=15 wher xk≥0
View SolutionIf x1,x2,x3,x4,x5,x6 all are independent then the maximum and minimum values of [sin−1x1]+[cos−1x2]+[tan−1x3]+[cot−1x4]+[sec−1x5]+[cosec−1x6], where [] represents greatest integer function, respectively are
View SolutionConsider the system of equations x_1+x_(2)^(2)+x_(3)^(3)+x_(4)^(4)+x_(5)^(5)=5 and x_1+2x_2+3x_3+4x_4+5x_5=15 where x_1,x_2,x_3,x_4,x_5 are positive real numbers. Then numbers of (x_1,x_2,x_3,x_4,x_5) is ___________.
View SolutionThe number of solutions to the system of equations x1+x2+x3+x4+x5=20 and x1+x2=15 wher xk≥0
View Solution
