Suppose ABC is a triangle and D and E are the mid points of AB and AC respectively.
Choose the correct answer from the bracket and fill in the blank
−−→BA+−−→AC=……
(oversetrarr(CB),oversetrarr(BC),1/2 oversetrarrr(BC),2oversetrarr(BC))
−−→BC
Topper's Solved these Questions
Similar Questions
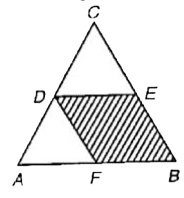
ABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
View SolutionIn the adjoining figure D, E and F are the mid - points of the sides BC, AC and AB respectively to triangle. Show that ∠EDF=∠A, ∠DEF=∠B and ∠DFE=∠C:

View Solution
Knowledge Check
Area of ΔABC=30cm2. D and E are the mid - points of BC and AB respectively. Find A(ΔBDE) :
A10cm2B7.5cm2C15cm2Dnone of theseGiven an equilateral triangle ABC, D, E and F are the mid-points of the sides AB, BC and AC respectively, then the quadrilateral BEFD is exactly a
AsquareBrectangleCparallelogramDrhombus
In ΔABC,D,E and F are mid-point of sides AB ,BC and AC respectively , Prove that AE and DF bisect each other.
View SolutionIn the adjoining figure D, E and F are the mid-points of the sides BC, AC and AB respectively. △DEF is congruent to triangle :

View SolutionABC is a triangle and D is the mid-point of BC . The perpendiculars from D to AB and AC are equal. Prove that the triangle is isosceles.
View SolutionIf DandE are the mid-points of sides ABandAC of a triangle ABC respectively, show that →BE+→DC=32→BC.
View SolutionIn triangle ABC, D and E are mid-points of sides AB and BC respectively. Also, F is a point in side AC so that DF is parallel to BC
(i) Prove that DBEF is a parallelogram.
View SolutionIf D and E are the mid-points of sides AB and AC of a triangle ABC respectively, show that −−→BE+−−→DC=32−−→BC.
View Solution
