Find the shortest distance between the lines ¯r=i+2j+3k+λ(i+j+k) and
¯r=i+j+k+μ(i+j+k)Solution in Malayalam
Step by step video & image solution for Find the shortest distance between the lines barr=i+2j+3k+lambda(i+j+k) and barr=i+j+k+mu(i+j+k) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Consider the following information regarding the purchase of pens and penciles by the three students Ravi, Twinkle and Lal from a shop.
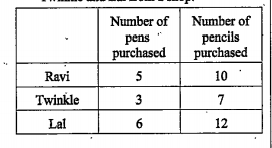 Represent the above data in the form of a 3×2 matrix. What does the entry in the 2nd row and in the first column represent?
Represent the above data in the form of a 3×2 matrix. What does the entry in the 2nd row and in the first column represent?
View SolutionLet A be the set of all 50 students of class X in a school. Let f:A→N
be function defined by f(x) = roll numbers of the student x. Show that
f is one-one but not onto
View SolutionFind the shortest distance and the vector equation of the line of shortest distance between the lines given by r=(3ˆi+8ˆj+3ˆk)+λ(3ˆi−ˆj+ˆk)andr=(−3ˆi−7ˆj+6ˆk)+μ(−3ˆi+2ˆj+4ˆk).
View SolutionIf the lines ¯r=(2i)+λ(i+2j+mk) and ¯r=(i+j)+μ(2i+j+2k) are mutually perpendicular, them: m=
View SolutionIf the lines ¯r=(i−j+k)+λ(π+j) and ¯r=(2i−j)+μ(i−j+4k) are coplanar then: p=
View SolutionThe shortest distance between the line r = 3i + 5j + 7k + λ (I + 2j + k) and r = I - j - k + μ (7i - 6j + k) is
View SolutionThe shortest distance between the lines r=3i+5j+7k+λ(i+2j+k)andr=−i−j−k+μ(7i−6j+k) is
View SolutionFind an equation of the line of shortest distance between the lines
r=λ(i−j+k)andr=(i−j)+μ(−2j+k)
In the vectorial notation and Cartesian notation. Also find the shortest distance between them.
View SolutionEquation of the plane containing the lines.
r=i+2j−k+λ(i+2j−k)
and r=i+2j−k+μ(i+j−3k) is
View SolutionShow that the line ¯r=i+j+λ(2i+j+4k) is parallel to the plane ¯r.(−2i+k)=5 .
View SolutionFind the distance between the line ¯r=i+j+λ(2i+j+4k) and the plane ¯r.(−2i+k)=5 .
View SolutionConsider the lines ¯r=(i+2j−2k)+λ(i+2j)
and ¯r=(i+2j−2k)+μ(2j−k)
Find the angles between the lines.
View SolutionConsider the line ¯r=(2i−j+k)+λ(i+2j+3k). Find the Cartesian equation of the line.
View SolutionConsider the line ¯r=(2i−j+k)+λ(i+2j+3k)
Find the vector equation of the line passing through A (1,0,2) and parallel to the line.
View SolutionConsider the pair of lines
¯r=3i+4j−2k+λ(−i+2j+k)….L1,
¯r=i−7j−2k+μ(i+3j+2k)….L2
Find the shortest distance between L1 and L2
View SolutionFind the shortest distance between the lines ¯r=(2i−j−k)+λ(3i−5j+2k) and ¯r=(i+2j+k)+μ(i−j+k)
View Solution
