The solution set of 6≤−3(2x−4)<12,x∈R is
(0,1]
[1,0)
(0,1)
[0,1]
The correct Answer is:A
To solve the inequality 6≤−3(2x−4)<12, we will break it down into two parts and solve step by step.
Step 1: Write the inequality
We start with the given inequality:
6≤−3(2x−4)<12
Step 2: Distribute the -3
Next, we distribute −3 to the terms inside the parentheses:
−3(2x−4)=−6x+12
So, we can rewrite the inequality as:
6≤−6x+12<12
Step 3: Split the inequality
Now we will split the compound inequality into two separate inequalities:
1. 6≤−6x+12
2. −6x+12<12
Step 4: Solve the first inequality
Let's solve the first inequality 6≤−6x+12:
1. Subtract 12 from both sides:
6−12≤−6x
This simplifies to:
−6≤−6x
2. Divide by -6 (remember to reverse the inequality sign):
1≥xorx≤1
Step 5: Solve the second inequality
Now, let's solve the second inequality −6x+12<12:
1. Subtract 12 from both sides:
−6x<12−12
This simplifies to:
−6x<0
2. Divide by -6 (again, reverse the inequality sign):
x>0
Step 6: Combine the results
From the two inequalities we found:
1. x≤1
2. x>0
Combining these results, we have:
0<x≤1
Step 7: Write the solution set
The solution set can be expressed in interval notation as:
x∈(0,1)
We start with the given inequality:
6≤−3(2x−4)<12
Next, we distribute −3 to the terms inside the parentheses:
−3(2x−4)=−6x+12
So, we can rewrite the inequality as:
6≤−6x+12<12
Now we will split the compound inequality into two separate inequalities:
1. 6≤−6x+12
2. −6x+12<12
Let's solve the first inequality 6≤−6x+12:
1. Subtract 12 from both sides:
6−12≤−6x
This simplifies to:
−6≤−6x
2. Divide by -6 (remember to reverse the inequality sign):
1≥xorx≤1
Now, let's solve the second inequality −6x+12<12:
1. Subtract 12 from both sides:
−6x<12−12
This simplifies to:
−6x<0
2. Divide by -6 (again, reverse the inequality sign):
x>0
From the two inequalities we found:
1. x≤1
2. x>0
0<x≤1
The solution set can be expressed in interval notation as:
x∈(0,1)
Topper's Solved these Questions
- LINEAR INEQUALITIES BOOK - ICSECHAPTER - LINEAR INEQUALITIES EXERCISE - MULTIPLE CHOICE QUESTION
16 Videos
- LIMITS AND DERIVATIVES BOOK - ICSECHAPTER - LIMITS AND DERIVATIVES EXERCISE - Multiple Choice Questions
31 Videos
- MATHEMATICAL INDUCTIONBOOK - ICSECHAPTER - MATHEMATICAL INDUCTIONEXERCISE - Exercise (a)
1 Videos
Similar Questions
Knowledge Check
The solution set of −12<4−3x−5≤2,x∈R is
A(103,803]B[−803,103)C(−803,−103]D(803,−103)The solution set of −1≤3+4x<23,x∈W is
A{0, 1, 2, 3, 4}B{−1,0,1,2,3,4}C{0,1,2,3,4,5}D{−1,0,1,2,3,4,5}Assertion: The solution set of −1<3+4x≤23,x∈R on the number line is
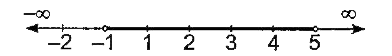
Reason: In the solution set, the number -1 is not included and the number 5 is included.ABoth assertion and reason are correct and reason is the correct explanation of assertionBBoth assertion and reason are correct but reason is not the correct explanation of assertionCAssertion is correct but reason is incorrectDAssertion is incorrect but reason is correct
Solve and graph the solution set on a number line :
2x5+1<−3,x∈R
View SolutionAssertion: The solution set of 2x−3≤x+1≤4x+7,x∈N is {1, 2, 3,4} .
Reason: If the solution of a linear inequation is x>2,x∈R then on the number line, it is represented as
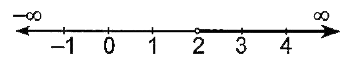
View SolutionThe solution set of −2+10x≤13x+10<24+10x,x∈Z is
View SolutionThe solution set of 2x+13<3x−25x∈R on the number line is
View SolutionThe solution set of 11x−4<15x+4≤13x+14,x∈I, on the number line is
View Solution
