If for a distribution and and the total number of observations is 50, then variance is
(a) 20
(b) 21
(c) 22
(d) 23
The correct Answer is:D
To find the variance of the given distribution, we will use the formula for variance:
σ2=∑x2iN−(∑xiN)2
Where:
- σ2 is the variance,
- ∑x2i is the sum of the squares of the observations,
- ∑xi is the sum of the observations,
- N is the total number of observations.
Given:
- ∑x2i=2400
- ∑xi=250
- N=50
Now, let's calculate the variance step by step:
Step 1: Calculate ∑x2iN
∑x2iN=240050
Calculating this gives:
240050=48
Step 2: Calculate ∑xiN
∑xiN=25050
Calculating this gives:
25050=5
Step 3: Calculate (∑xiN)2
Now, we square the result from Step 2:
(∑xiN)2=52=25
Step 4: Substitute values into the variance formula
Now we substitute the values we calculated into the variance formula:
σ2=48−25
Calculating this gives:
σ2=23
Conclusion
Thus, the variance of the distribution is:
Variance=23
Final Answer
The correct answer is option D: 23.
---
- σ2 is the variance,
- ∑x2i is the sum of the squares of the observations,
- ∑xi is the sum of the observations,
- N is the total number of observations.
- ∑x2i=2400
- ∑xi=250
- N=50
Topper's Solved these Questions
- BOOK - ICSECHAPTER - STATISTICSEXERCISE - EXERCISE 28 (A)5videos
STATISTICS
- BOOK - ICSECHAPTER - STATISTICSEXERCISE - EXERCISE 28 (B)11videos
STATISTICS
- BOOK - ICSECHAPTER - STATISTICSEXERCISE - CHAPTER TEST4videos
STATISTICS
- BOOK - ICSECHAPTER - SETSEXERCISE - CHAPTER TEST46videos
SETS
- BOOK - ICSECHAPTER - STRAIGHT LINES EXERCISE - Multiple Choice Questions46videos
STRAIGHT LINES
Similar Questions
Knowledge Check
- Question 1 - Select One
If for a distribution ∑(x−5)=3,∑(x−5)2=43 and the total number of terms is 18 then mean and variance are
A¯x=9318,σ2=8536B¯x=9518,σ2=8536C¯x=316,σ2=8536D¯x=9518,σ2=8336
The mean of the following frequency distribution is 57.6 and the sum of the observations is 50. Find the missing frquencies f1andf2 :
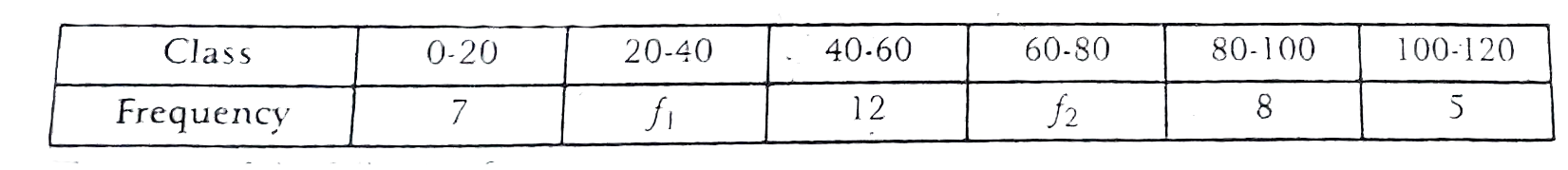 View Solution
View SolutionIf for a sample of size 60, we have the following information ∑x2i=18000 and ∑xi=960 , then the variance is (a) 6.63 (b) 16 (c) 22 (d) 44
View SolutionIf for distribution of 18 observations ∑(xi−5)=3and∑(xi−5)2=43, find the mean and standard deviation.
View SolutionIf for distribution of 18 observations ∑(xi−5)=3and∑(xi−5)2=43, find the mean and standard deviation.
View SolutionA binomial distribution has mean 5 and variance 4. Find the number of trials.
View SolutionIn a binomial distribution, mean is 5 and the variance is 4 . The number of trials is
View SolutionThe standard deviation (σ) of variate x is the square root of the A.M. of the squares of all deviations of x from the A.M. observations.
If xi/fi, i=1,2,… n is a frequency distribution then σ=√1NΣni=1fi(xi−¯x)2,N=Σni=1fi and variance is the square of standard deviation. Coefficient of dispersion is σx and coefficient of variation is σx×100
For a given distribution of marks mean is 35.16 and its standard deviation is 19.76 then coefficient of variation isView Solution

