The natural vibration frequency of a hydrogen molecule is 1.26×1014 Hz. Find the zero-point energy of the vibrations of the molecule. Can the vibrational degrees of freedom in the molecule be excited at 600 K?
लिखित उत्तर
The correct Answer is:0.26 eV. They can.
The zero-point energy is ε0=1/2hv. The energy of the first excited state is ε1=32hv, so the excitation energy is Δε=ε1−ε0=hv. The vibrational degrees of freedom will no longer be excited when the energy of thermal motion becomes less than the excitation energy. The usual criterion is 32kT≤hv, which yields the minimum temperature
Tvib=2hv/3k=4×103K
This does not agree with experiment, since the lines of the vibrational spectrum of hydrogen molecules are observed at lower temperatures.
This is because of the Maxwellian molecular speed distribution (see 25.2), which shows that a gas contains molecules whose speeds are far in excess of the average. For instance, about 2% of the molecules have speeds three times greater than the average. The energy of such molecules is more than 9 times the average kinetic energy.
|
समान प्रश्न
Given, Avogadro's number N=6.02×1023 and Boltzmann's constant k=1.38×10−23J/K.
(a) Calculate the average kinetic energy of translation of the molecules of an ideal gas at 0∘Candat100∘C.
(b) Also calculate the corresponding energies per mole of the gas.
View SolutionA mock− up of a CO2 molecule consists of three balls interconnected by identical light springs and placed along a straight line in the state of equilibrium. Such a system can freely perform oscillation of two types, as shown by the arrows in figure. Knowing the masses of the atomes, find the ration of frequencies of these oscillations
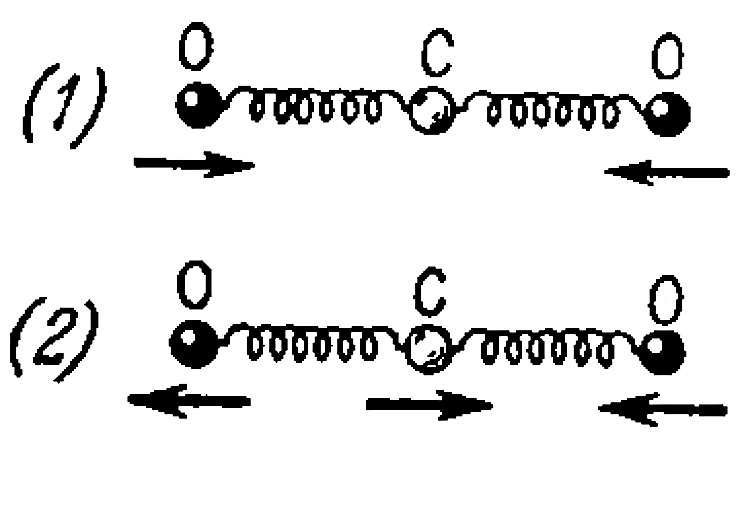
View Solution
ज्ञान जांच
A helium atom is two times heavier than a hydrogen molecule. At 298K, the average kinetic energy of a helium atom is
Atwo times that of a hydrogen moleculeBsame as that of a hydrogen moleculeCfour times that of a hydrogen moleculeDhalf that of a hydrogen moleculeMention the degree of freedom for diatomic gas molecules without vibration.
AStatement-1 is true, statement-2 is true, statement-2 is a correct explanation for statement-1BStatement-1 is true, statement-2 is true, statement-2 is not a correct explanation for statement-1Cstatement-1 is true, statement-2 is falseDstatement-1 is false, statement-2 is trueAn aluminium rod having a length 100 cm is clamped at its middle point and set into longitudinal vibrations. Let the rod vibrate in its fundamental mode. The density of aluminium is 2600 kg/m3 and its Young's modulus is 7.8×1010N/m2. The frequency of the sound produced is :-
A1250 HzB2740 HzC2350 HzD1685Hz
