Determine the Miller indices of the shaded plane. Coordinates of the corners of the plane are shown in the figure below.

Text Solution
A plane parallel to the plane between (0, 0, 0), (1,1, 0) and (0,1,1) will intersect the axes at x = a, y = - b and z = c.

Thus the Miller indices are (1,¯1,1) or (¯1,1,¯1)
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RC MUKHERJEECHAPTER - SOLID AND LIQUID STATES EXERCISE - PROBLMES (ANSWERS BRACKTED WITH QUESTIONS)38videos
SOLID AND LIQUID STATES
- BOOK - RC MUKHERJEECHAPTER - SOLID AND LIQUID STATES EXERCISE - OBJECTIVE PROBLEMS51videos
SOLID AND LIQUID STATES
- BOOK - RC MUKHERJEECHAPTER - PROPERTIES OF GASESEXERCISE - OBJECTIVE PROBLEMS5videos
PROPERTIES OF GASES
- BOOK - RC MUKHERJEECHAPTER - VOLUMETRIC CALCULATIONS EXERCISE - OBJECTIVE PROBLEMS37videos
VOLUMETRIC CALCULATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
The area of the shaded region in the figure given below is
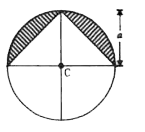 Aa22(π2−1) sq. unitsBa2(π−1) sq . UnitsCa2(π2−1) sq . UnitsDa22−(π−1) sq . Units
Aa22(π2−1) sq. unitsBa2(π−1) sq . UnitsCa2(π2−1) sq . UnitsDa22−(π−1) sq . Units - Question 2 - Select One

Determine the fraction of the shaded parts in the figure shown.A25B1125C1225D1225 - Question 3 - Select One
The Miller indices of a plane having intercepts 2a,2b,∞are
A110B220C100D10
The area of the shaded region shown in the figure is
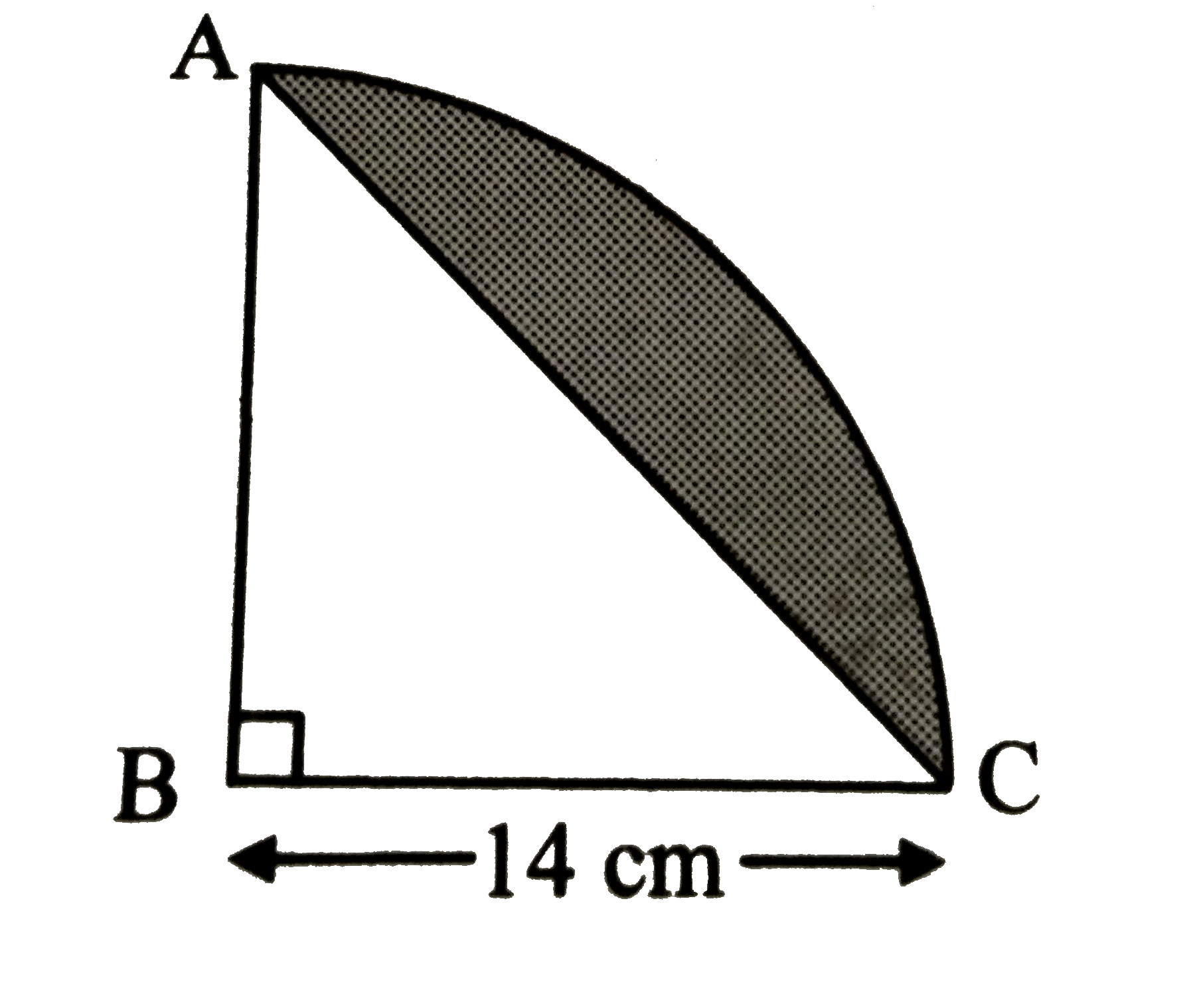 View Solution
View SolutionCalculate the area of the shaded region in the figure given below.
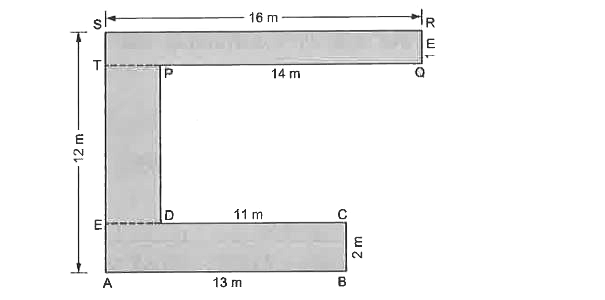 View Solution
View SolutionFind the area of the shaded part of the figure given below.
View SolutionThe Miller indices of a plane having intercepts 2a,2b,∞are
View SolutionThe corner points of the feasible region, shown as shaded in the graph below, are :
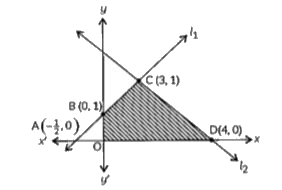 View Solution
View Solution

