Question

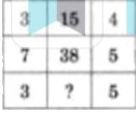
$18!+19!$

Answer

`=4`

Explanation

To solve the expression $$18!+19!$$, we can follow these steps:

Step 1: Rewrite \( 19! \) in terms of \( 18! \)
We know that \( 19! \) can be expressed as:
\( 19! = 19 \times 18! \)

Step 2: Substitute \( 19! \) in the original expression
Now, we can substitute this back into the original expression:
\( 18! + 19! = 18! + 19 \times 18! \)

Step 3: Factor out \( 18! \)
Next, we can factor out \( 18! \) from both terms:
\( 18! + 19 \times 18! = 18!(1 + 19) \)

Step 4: Simplify the expression inside the parentheses
Now, simplify the expression inside the parentheses:
\( 1 + 19 = 20 \)

Step 5: Write the final expression
Now we can write the final expression:
\( 18! + 19! = 18! \times 20 \)

Final Answer
Thus, the value of \( 18! + 19! \) is:
\( 18! \times 20 \)

---