Question

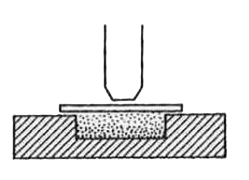
In 1908-1910 Perrin determined the Avogadro number. He did it by observing the distribution of tiny gumboge gum balls in water with the aid of a short-focus microscope (Fig.). By adjusting the focus of the microscope to observe a definite layer he was able to count the number of particles in each layer. In one of the experiments the following data were obtained:
${\displaystyle \begin{array}{lllll}\text{Height of the layer above the}& & & & \\ \text{tray's bottom,}\mu & 5& 35& 65& 95\\ \text{Number of particles in the layer}& 100& 47& 23& 12\end{array}}$
Knowing the ball's radius to be 0.212 um, the density of gumboge gum to be ${\displaystyle 1.252\times {10}^{3}kg/m^3}$ the density of water at ${\displaystyle {27}^{\circ }C}$ to be ${\displaystyle 0.997\times {10}^{3}kg/m^3}$ find the Avogadro number. 

Answer

`N_(A) = (5.9 pm 0.3) xx10^(26)" kmole"^(-1)` . [Hint The Brownian particles behave like gigantic molecules , and the barometric distribution is valid for them]

Explanation

Small gumboge gum balls take part in random thermal motion, behaving like gigantic molecules. Therefore their distribution over height corresponds to the barometric distribution
`(N_1)/(N_2) = e^((mg(h_2 -h_1))/(kt))”log” (N_1)/(N_2) = (0.434mg(h_2 - h_1))/(kT)`
One should take into account that in addition to the force of gravity, the particles are acted upon by the Archimedean force. Expressing the Boltzmann constant in terms of the gas constant and the Avogadro number, we obtain for the latter
`N_A = (3RT “log” (N_1//N_2))/(0.434 xx 4 pi r^3 g(rho - rho_0) (h_2 - h_1))`
Substituting the known data, we obtain the working formula
`N = 5.79 xx 10^(22) (“log” (N_1//N_2))/(h_2 - h_1)`
 The values obtained for the Avogadro number are: `6.32 xx 10^26, 5.98 xx 10^26` and `5.45 xx 10^26` The average value is 5.92 X 1026, the maximum error is `0.3 xx 10^26`. Hence from data obtained in this experiment
`N_A = (5.9 pm 0.3) xx 10^(26) “k mol”^(-1)`.