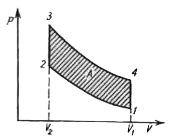
Figure depicts an idealized cycle of a petrol internal combustion engine. The segment 1-2 corresponds to the adiabatic compression of the combustible mixture, segment 2-3, to the isochoric combustion of fuel in the course of which the working fluid receives an amount of heat Q, segment 3-4 corresponds to the adiabatic expansion of the working fluid, segment 4-1, to the isochoric exhaust of spent gases. Express the engine's efficiency in terms of the gas compression ratio x=V2/V1. 
Figure depicts an idealized cycle of a petrol internal combustion engine. The segment 1-2 corresponds to the adiabatic compression of the combustible mixture, segment 2-3, to the isochoric combustion of fuel in the course of which the working fluid receives an amount of heat Q, segment 3-4 corresponds to the adiabatic expansion of the working fluid, segment 4-1, to the isochoric exhaust of spent gases. Express the engine's efficiency in terms of the gas compression ratio x = V2//V1. 

Text Solution
Verified by Experts

The correct Answer is:η=1−xγ−1

The efficiency of the cycle is η=W/Q. Since the work in isochoric sections is zero, the useful work is equal to the difference between the work of adiabatic expansion and that of adiabatic compression :
W=mMCmV(T3−T4)-
−mMCmV(T2−T1)=
=mMCmV(T3−T2+T1−T4)
The working medium receives heat in the process of isochoric combustion of fuel :
Q=mMCmV(T3−T2)
Hence η=1−T4−T1T3−T2
Making use of the result, express the temperatures in terms of the volumes. We have Vγ2T2=Vγ−11T1andVγ−12T3=Vγ−11T4. Dividing the first equality by the second, we obtain T2/T3=T1/T4. Transform the expression for the efficiency and reduce it to the following form:
η=1−T4T3⋅1−(T1/T4)1−(T2/T3)
But the second fraction is , evidently , unity and the first fraction T4/T3=(V2/V1)γ−1=xγ−1. Hence η=1−xγ−1.
ocr_image

Doubtnut Promotions Banner
|