If you sling a thin wire loop around a block of ice and attach to it a weight of several kilograms then after some time the wire will pass through the block of ice, but the block remains intact (Fig. 23.2). Explain this phenomenon.
Ice melts under the pressure of the wire, and the wire sinks: the water formed above the wire immediately freozes again.
Similar Questions
In the arrangement shown in the fig. a monkey of mass M keeps itself as well as block A at rest by firmly holding the rope. Rope is massless and the pulley is ideal. Height of the monkey and block A from the floor is h and 2h respectively [h = 2.5 m]
(a) The monkey loosens its grip on the rope and slides down to the floor. At what height from the ground is block A at the instant the money hits the ground?
(b) Another block of mass equal to that of A is stuck to the block A and the system is released. The monkey decides to keep itself at height h above the ground and it allows the rope to slide through its hand. With what speed will the block strike the ground?
(c) In the situation described in (b), the monkey decides to prevent the block from striking the floor. The monkey remains at height h till the block crosses it. At the instant the block is crossing the monkey it begins climbing up the rope. Find the minimum acceleration of the monkey relative to the rope, so that the block is not able to hit the floor. Do you think that a monkey can climb with such an acceleration? (g=10ms−2)

View SolutionThere exists a uniform magnetic field in horizontal direction perpendicular to the plane of the paper as shown in Fig. A wire of length 1 and mass m is attached with a block of equal mass with the help of an ideal string. The block is kept on floor and the wire on two conducting support as shown. After closing the switch, a charge q passes though the wire for a small interval of time. To what height the block will rise? (takeqBlm=20)
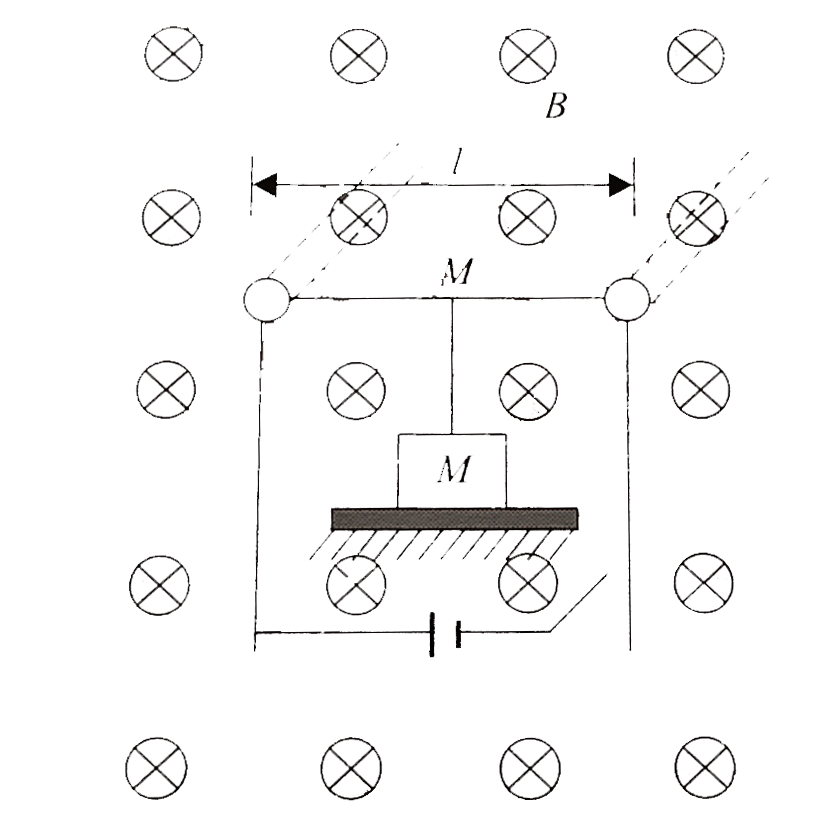
View Solution
Knowledge Check
There exists a uniform magnetic field in horizontal direction perpendicular to the plane of the paper as shown in Fig. A wire of length 1 and mass m is attached with a block of equal mass with the help of an ideal string. The block is kept on floor and the wire on two conducting support as shown. After closing the switch, a charge q passes though the wire for a small interval of time. To what height the block will rise? (takeqBlm=20)
 A10 mB5 mC2.5 mD1 m
A10 mB5 mC2.5 mD1 mA block of ice of mass 50kg is sliding on a horizontal plane. It starts with speed 5ms−1 and stop 3 after moving through some distance. The mass of ice that has melted due to friction between the block and the suface is (assuming that no energy is lost nad the surface is (assuming that no energy is lost and latent heat of fustion of ice is 80 calg−1)
A2.86 gB3.86 gC0.86 gD1.86 gPQRS is a square region of side 2a in the plane of paper. A uniform magnetic field B, directed perpendicular to the plane of paper and into its plane is confined within this square region. A square loop of side 'a' and made of a conducting wire of resistance R is moved at a constant velocity →v from left to right in the plane of paper as shown. Obviously, the square loop will enter the magnetic field at some time and then leave it after some time. During the motion of loop, whenever magnetic flux through it changes, emf will be induced resulting in induced current. Let the motion of the square loop be along x-axis and let us measure x coordinate of the centre of square loop from the centre of the square magnetic field region (taken as origin). Thus, x coordinate will be positive if the centre of square loop is to the right of the origin O (centre of magnetic field) and negative if centre is to the left.

For x=a/4
(i) magnetic flux through the loop,
(ii) induced current in the loop and
(iii) external force required to maintain constant velocity of the loop, will beA(i) Ba2 (ii) Bav2R (iii) B2a2v24R2B(i) Ba2 (ii) zero (iii) zeroC(i) Ba2 (ii) Bav2R (iii) zeroD(i) zero (ii) zero (iii) zero
Figure shows water in a container having 2.0−mm thick walls made of a material of thermal conductivity 0.50Wm−1C−1 . The container is kept in a melting-ice bath at 0∘C . The total surface area in contact with water is0.05m2 . A wheel is clamped inside the water and is coupled to a block of mass M as shown in the figure. As the goes down, the wheel rotates. It is found that after some time a steady state is reached in which the block goes down with a constant speed of 10cms−1 and the temperature of the water remains constant at 1.0∘C .Find the mass M of the block. Assume that the heat flow out of the water only through the walls in contact. Take g=10ms−2 .

View SolutionFigure shows water in a container having 2.0−mm thick walls made of a material of thermal conductivity 0.50Wm−1^(@)C^(-1).Theconta∈eriskept∈ame<∈g−icebathat0^(@)C.The→talsurfacearea∈contactwithwateris0.05m^(2).Awheelisclamped∈sthewaterandiscoup≤→ablockofmassMasshowm∈thefigure.Asthegoesdown,thewheelrotates.Itisfoundtˆafterso±etimeasteadystateisreached∈whichtheblockgoesdonwwithacontantspeedof10cm^(-1)andthetemperatureofthewaaterrema∈scontantat1.0^(@)C.F∈dthemassMoftheblock.As∑etˆtheheatflowoutofthewateronlythroughthewalls∈contact.Takeg=10ms^(-2) .

View SolutionIn this section you have few short passages. After each passage, you will find some items based on the passage. First, read a passage and answer the items based on it. You are required to select your answers based on the contents of the passage and opinion of the author only.
Passage
For many years, ship captains navigating the waters of Antarctica have been intrigued by sightings of emerald icebergs. Scientists have now explained their mystery: There icebergs are turned upside down. Icebergs are blocks of ice that have broken off huge slabs of frozen snow called ice shelves. Their green appearance results from sea water that has frozen at the bottom over hundreds of years. The frozen sea water has dissolved organic matter which gives it a yellow tone and the fresh water 'ice shelf' above has a blue tinge. When the iceberg turns upside down, it appears green through the visual mix of yellow with the blue from below.
What is the meaning of 'intrigued' ?
View SolutionPQRS is a square region of side 2a in the plane of paper. A uniform magnetic field B, directed perpendicular to the plane of paper and into its plane is confined within this square region. A square loop of side 'a' and made of a conducting wire of resistance R is moved at a constant velocity →v from left to right in the plane of paper as shown. Obviously, the square loop will enter the magnetic field at some time and then leave it after some time. During the motion of loop, whenever magnetic flux through it changes, emf will be induced resulting in induced current. Let the motion of the square loop be along x-axis and let us measure x coordinate of the centre of square loop from the centre of the square magnetic field region (taken as origin). Thus, x coordinate will be positive if the centre of square loop is to the right of the origin O (centre of magnetic field) and negative if centre is to the left.

For x=a/4
(i) magnetic flux through the loop,
(ii) induced current in the loop and
(iii) external force required to maintain constant velocity of the loop, will be
View SolutionTake a small slab of ice, a thin wire, two identical weights. Activity :
(1) Put a slab of ice on a stand as shown in figure.
(2) Hang two equal weights to the two ends of a metal wire and put the wire on the slab as shown in the figure.
What do you observe ?
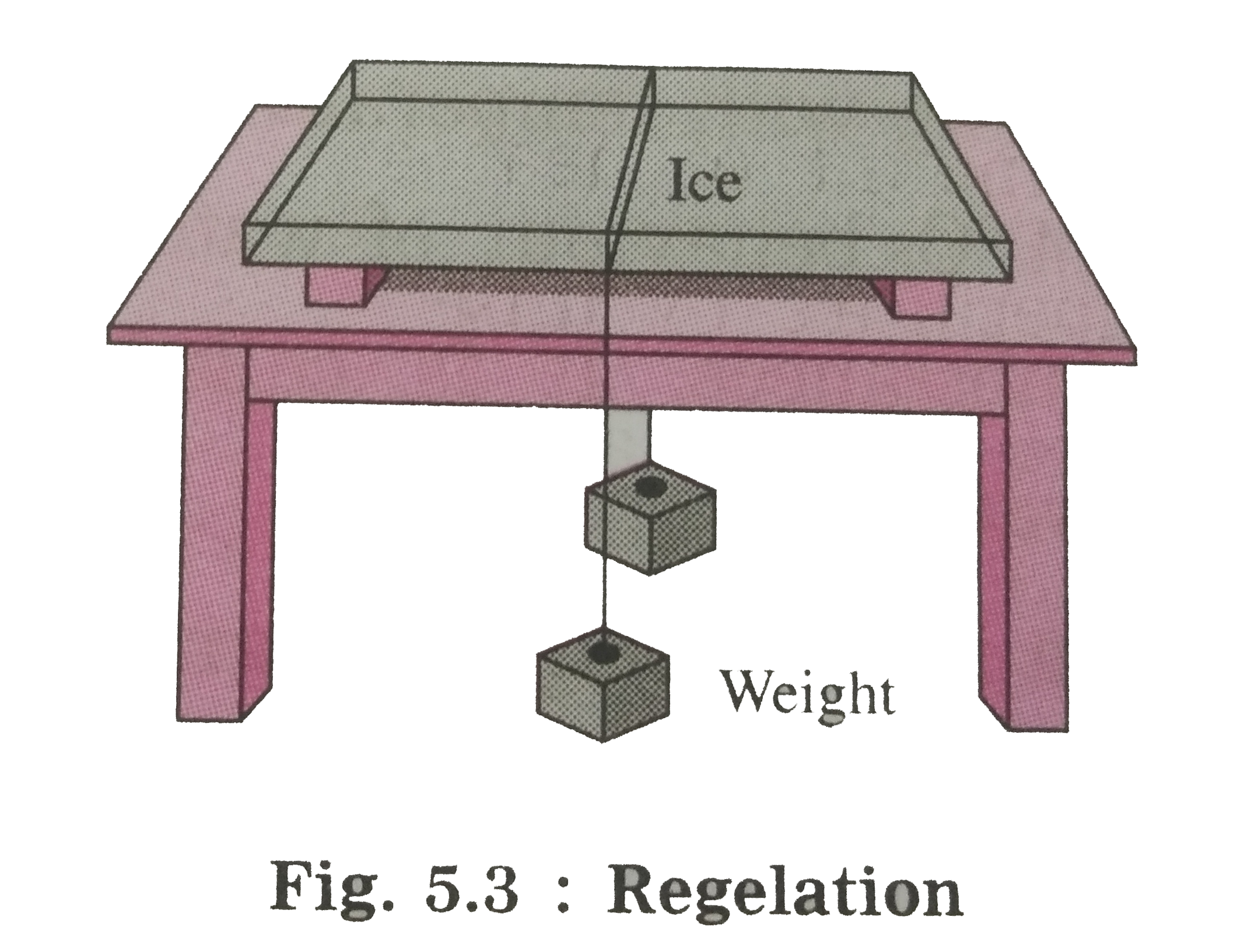
View Solution
