Compare the electrical conductivity of pure germanium at . The activation energy for germanium is 0.72 eV
The correct Answer is:
The mean free path of the electron is much less dependent on the temperature than the exponential term, therefore the temperature dependence of the factor B can be neglected in the first approximation. We have
Similar Questions
Knowledge Check
Compare Ea (activation energy) for a, b, c and d.
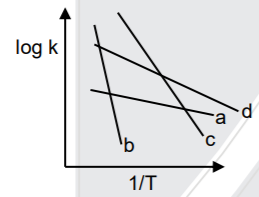 AEb>Ec>Ed>EaBEa>Ed>Ec>EbCEc>Eb>Ea>EdDEd>Ea>Eb>Ec
AEb>Ec>Ed>EaBEa>Ed>Ec>EbCEc>Eb>Ea>EdDEd>Ea>Eb>EcThe maximum wavelength of electromagnetic radiation, which can create a hole-electron pair in germanium. (Given that forbidden energy gap in germanium is 0.72 eV)
A1.7×10−6 mB1.5×10−5 mC1.3×10−4 mD1.9×10−5 m
Determine the conductivity of pure germanium at 27∘C. The concentration of the carriers at this temperature is 2.2×1019m−3. The mobility of electrons = 0.36 and that of holes = 0.17
View SolutionCompare Ea (activation energy) for a, b, c and d.

View SolutionDetermine the conductivity and resistivity of pure germanium at 300K assuming that at this temperature the concentration of germanium is 2.5×1013cm−3. The electron and hole mobilities are 3600cm2V−1s−1 and 1700cm2V−1s−1 respectively.
View SolutionThe intrinsic conductivity of germanium at 27∘C is 2.13ohm−1⋅m−1, the mobilities of electrons and holes are 0.38 and 0.18m2/(V⋅s), respectively. Compute the carrier densities and the Hall coefficient.
View SolutionThe intrinsic conductivity of germanium at 27∘C is 2.13ohm−1⋅m−1, the mobilities of electrons and holes are 0.38 and 0.18m2/(V⋅s), respectively. Compute the carrier densities and the Hall coefficient.
View SolutionThe energy gap in germanium is 0.75eV. Compare the intrinsic conductivities of germanium at 300K and 330K. Take kB=8.6×10−5eVK−1
View Solution
