Emery powder particles are stirred up in a beaker of water 0.1 m deep. Assuming the particles to be spherical and of all sizes, calculate the radius of the largest particle remaining in suspension after 24 hours. Given that density of emery is4000kgm−3 and coefficient of Viscosity water is 0.001 decapoise.
To solve the problem of finding the radius of the largest emery powder particle that can remain suspended in water for 24 hours, we will use the concept of terminal velocity. Here are the steps to arrive at the solution:
1. Understand the concept of terminal velocity:
The terminal velocity V of a spherical particle falling through a fluid can be expressed using the formula:
V=29⋅r2⋅g⋅(ρp−ρf)η
where:
- r = radius of the particle (in meters)
- g = acceleration due to gravity (approximately 9.8m/s2)
- ρp = density of the particle (emery powder, 4000kg/m3)
- ρf = density of the fluid (water, 1000kg/m3)
- η = coefficient of viscosity of the fluid (water, 0.001Pa.s or 0.001kg/(m.s))
2. Calculate the terminal velocity:
The terminal velocity must be equal to the average speed of the particle over the time period of 24 hours. The average speed V can be calculated as:
V=displacementtime=0.1m24hours=0.1m24×3600s=0.186400m/s
3. Convert time to seconds:
24hours=24×60×60=86400s
Therefore,
V=0.186400≈1.157×10−6m/s
4. Rearrange the terminal velocity formula to solve for r2:
r2=V⋅9⋅η2⋅g⋅(ρp−ρf)
5. Substitute the known values into the equation:
- V≈1.157×10−6m/s
- η=0.001kg/(m.s)
- g=9.8m/s2
- ρp=4000kg/m3
- ρf=1000kg/m3
Plugging in these values:
r2=(1.157×10−6)⋅9⋅0.0012⋅9.8⋅(4000−1000)
r2=(1.157×10−6)⋅9⋅0.0012⋅9.8⋅3000
6. Calculate r2:
r2=1.157×10−6⋅9⋅0.0012⋅9.8⋅3000=1.157×10−6⋅0.00958800
r2≈1.0413×10−858800≈1.77×10−13m2
7. Find r:
r≈√1.77×10−13≈4.20×10−7m=4.20μm
Final Answer:
The radius of the largest particle remaining in suspension after 24 hours is approximately 4.20μm.
The terminal velocity V of a spherical particle falling through a fluid can be expressed using the formula:
V=29⋅r2⋅g⋅(ρp−ρf)η
where:
- r = radius of the particle (in meters)
- g = acceleration due to gravity (approximately 9.8m/s2)
- ρp = density of the particle (emery powder, 4000kg/m3)
- ρf = density of the fluid (water, 1000kg/m3)
- η = coefficient of viscosity of the fluid (water, 0.001Pa.s or 0.001kg/(m.s))
The terminal velocity must be equal to the average speed of the particle over the time period of 24 hours. The average speed V can be calculated as:
V=displacementtime=0.1m24hours=0.1m24×3600s=0.186400m/s
24hours=24×60×60=86400s
Therefore,
V=0.186400≈1.157×10−6m/s
r2=V⋅9⋅η2⋅g⋅(ρp−ρf)
- V≈1.157×10−6m/s
- η=0.001kg/(m.s)
- g=9.8m/s2
- ρp=4000kg/m3
- ρf=1000kg/m3
r2=(1.157×10−6)⋅9⋅0.0012⋅9.8⋅(4000−1000)
r2=(1.157×10−6)⋅9⋅0.0012⋅9.8⋅3000
r2=1.157×10−6⋅9⋅0.0012⋅9.8⋅3000=1.157×10−6⋅0.00958800
r2≈1.0413×10−858800≈1.77×10−13m2
r≈√1.77×10−13≈4.20×10−7m=4.20μm
The radius of the largest particle remaining in suspension after 24 hours is approximately 4.20μm.
Topper's Solved these Questions
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)
19 Videos
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS ( FROM PRESSURE, ARCHIMEDES. PRINCIPLE)
7 Videos
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - SELECTED PROBLEMS (FROM HEAT ENGINES)
21 Videos
- OSCILLATIONS BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (OSCILLATION IN A TUNNEL BORED THROUGH THE EARTH)
2 Videos
Similar Questions
A powder comprising particle of various sizes is stirred up in a vessel filled to a height of 10cm with water. Assuming the particle to be spherical, find the size of the largest particle that will remain in suspension after 1h (density of powder =4g/cm3, viscosity of water =0.01poise).
View SolutionSpherical particles of pollen are shaken up in water and allowed to settle. The depth of water is 2×10−2m. What is the diameter of the largest particles remaining in suspension one hour later? Density of pollen =1.8×103kgm−3 viscosity of water =1×10−2 poise and density of water =1×10−3kgm−3
View SolutionThe rate of water gushing out of a pipe of radius 5 cm is 100Lmin−1. The Reynolds number for the flow is of the orderof [density of water = 1000kgm−3 ,coefficient of viscosity of water = 1m Pa s ]
View SolutionWater from a pipe is coming at a rate of 100 litres per minute. If the radius of the pipe is 5cm, the Reynolds number for the flow is of the order of : (density of water =1000kg/m3, coefficient of viscosity of water =1mPas)
View SolutionA drop of water of radius 10−5m is falling through a medium whose density is1.21kgm−3 and coefficient of Viscosity 1.8×10−4 poise. Find the terminal velocity of the drop.
View SolutionAn air bubble of radius 2.0mm is formed at the bottom of a 3.3m deep river. Calculate the radius of the bubble as it comes to the surface. Atmospheric pressure =1.0×105Pa and desnity of water=1000kg m^(-3).
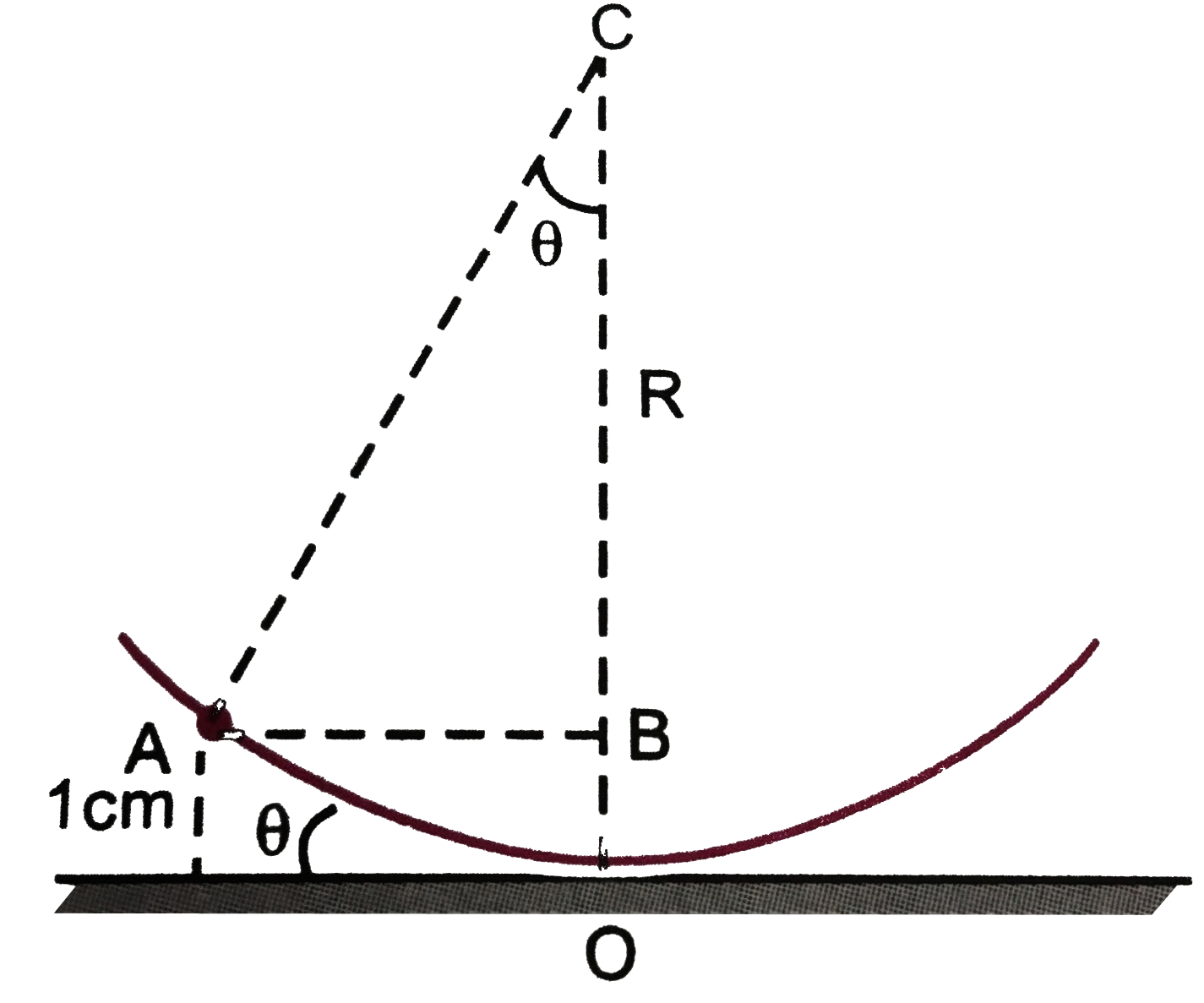
View SolutionA particle of mass 1 g executes an oscillatory motion on the concave surface of a spherical dish of radius 2m placed on a horizontal plane, Figure . If the motion of the particle begins from a point on the disc at a height of 1 cm. from the horizontal plane and coefficient of friction is 0.01 , find the total distance covered by the particle before coming to rest.
View SolutionA spherical mercury drop of 10−3m radius is sprayed into million drops of the same size. Calculate the energy used in doing so. Given surface tension of mercury =0.55Nm−1
View SolutionAn air bubble of radius 1 mm is located at a depth of 20 cm below water level. The excess pressure inside the bubble above the atmospheric pressure is [Given, the surface tension of water is 0.075 Nm−1 and density is 1000 kg m−3]
View SolutionThe wire of a Young's modules appartus is elongated by 2 mm when a brick is suspended from .it When the brick is immersed in water the wire contracts by 0.6mm Calculate the density of the brick given that the density of water is 1000kgm−3
View Solution
