Case OF Loop Entering Field and Categories OF EMI||Introduction to Variable B Case
Step by step video solution for Case OF Loop Entering Field and Categories OF EMI||Introduction to Variable B Case by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Oblique Collision || Variable Mass Case
View SolutionA square loop of edge a has N turns and a total resistance R . The loop moves with constant velocity v through a region of constant magnetic field B . The loop enters in magnetic field at t=0. Discuss the vartion of (a) Flux passing through the loop (b) the induced emf and (c) the external force acting on the loop a function of position of the loop in the field.

View Solution
Knowledge Check
In case of a uniform magnetic field, the lines of magnetic induction are
Anon-parallelBcurvedCequidistant and parallelDall of the aboveIn which of the following cases the emf is induced due to time varying magnetic feld (induced field emf)? Case I A magnet is moving along the axis of a conducting coil
Case II A loop having varying area (due to moving jumper) is placed in a magnetic field
case III The resistance of the coil is changing, which is connected to an ideal battery. case IV a current carrying wire is approaching a conducting ring.AI,II and III onlyBI,III and IV onlyCI,II and IV onlyDAll the fourIn case of infinite long wire electric field is proportional to
A1rB1r2C1r3Dr0
In how many categories, can the court cases be divided ?
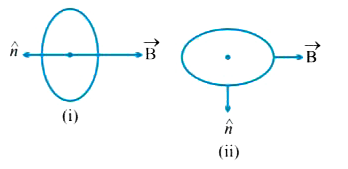
View SolutionA current loop is placed in a uniform magnetic field in the following orientations (1) and (2). Calculate the magnetic moment in each case.
View SolutionWhere is the gravitational field zero and where is the gravitational potential zero, in case of Earth?
View Solutiony = In 1−x21+x2 find d2y if :
(a)x is an independent variable,
(b) x is a function of another variable. Consider the particular case when x = tan t
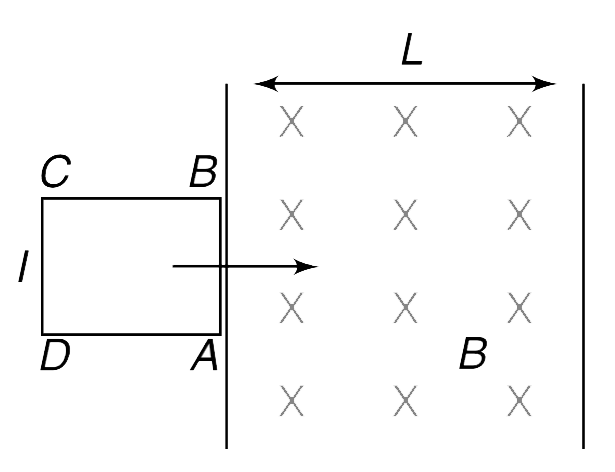
View SolutionA region of width L contains a uniform magnetic field B directed into the plane of the figure. A square conducing loop of side length l(<L) is kept with its side AB at the boundary of the field region (see Figure). The loop is pushed into the field region with a speed such that it just manages to exit the field region. Calculate the time needed for the entire loop to enter the field region after it is pushed. Mass and resistance of the loop is M and R respectively.
View Solution
