A force couple is the term used for a system of two equal antiparallel forces, the arm of the couple is the shortest distance between the forces. Prove that the torque is equal to the product of the magnitude of the force and the arm no matter what is the position of the point with respect to which the torque is determined.
Text Solution
The correct Answer is:Hint . Draw the axis of rotation perpendicular to the plane through an arbitrary point in the plane of the forces. Compute the moment of each force about this axis and find their sum.
From an arbitrary point o draw an axis perpendicular tothe plane in which the forces lie (Fig). The moment of the force couple
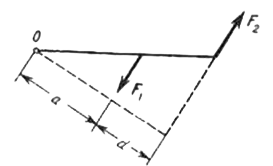
(the torque) is equal to the algebraic sum of the moments of each force about the axis. We have
M=F1a+F2(a+d)=F1a+F2a+F2d
However, F1=FandF2=−F , so M =-Fd. The minus sign shows that this couple makes a left-hand screw about the axis.
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
Square of the resultant of two forces of equal magnitude is equal to three times the product of their magnitude. The angle between them is
A0∘B45∘C60∘D90∘ - Question 2 - Select One
Square of the resultant of two forces of equal magnitude is equal to three times the product of their magnitude. The angle between them is
A0∘B45∘C60∘D90∘ - Question 3 - Select One
Two equal forces are acting at a point with an angle of 60∘ between them. If the resultant force is equal to 40√3 N, the magnitude of each force is
A40 NB20 NC80 ND30 N
Square of the resultant of two forces of equal magnitude is equal to three times the product of their magnitude. The angle between them is
View SolutionSquare of the resultant of two forces of equal magnitude is equal to three times the product of their magnitude. The angle between them is :
View SolutionTwo equal forces are acting at a point with an angle of 60° between them. If the resultant force is equal to 30√3 N, the magnitude of each force is
View SolutionSquare of the resultant of two forces of equal magnitude is equal to three times the product of their magnitude. The angle between them is :
View SolutionTwo equal forces are acting at a point with an angle 60∘ between them. If the resultant force is equal to 4√3N, the magnitude of each force is
View Solution

