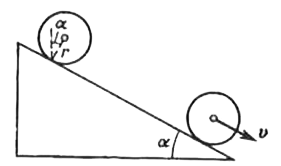
A solid cylinder with base radius jis placed on top of an inclined plane of length land slope angle α (Fig). The cylinder rolls down without slipping. Find the speed of the centre of mass of the cylinder at the bottom of the plane, if the coefficient of rolling friction is k. Can rolling friction be neglected? Do the calculation for the following conditions: l=1m,α=30∘,r=10cm,k=5×10−4m . What would be the speed if, in the absence of friction, the cylinder slides down?
The correct Answer is:2.7m/s;3.4m/s
From the law of conservation of energy
U−Mtr=K, or mg(lsinα+rcosα)−Troll=mv22+Iω22
The force of rolling friction is Trol=kmgcosα/r , the moment of inertia of a solid cylinder is I=1/2mr2 , and the angular velocity is ω=v/r . We have
mg(lsinα+rcosα)−lkmgcosαr=mv22+mv24
from which
v=√4g2(lsinα+rcosα−lkcosαr)
In principle the friction may not be neglected since in the absence of friction the disk will not roll down, but will slide down, and in this cage the kinetic energy of rolling should not be taken into account. But if the friction is small enough, the work of the force of friction may be neglected. The necessary condition for this is
lkr<<ltanα+r
For the numerical example contained in the problem we have
lkr=1×5×10−410−1=5×10−3,ltanα+r√33+0.1=0.68
i.e. the work of the force of rolling friction may be neglected in the calculation
When the cylinder slides down
mg(lsinα+rcosα)=12mv2,v=√2g(lsinα+rcosα)
Similar Questions
A solid cylinder of mass M and radius R rolls without slipping down an inclined plane of length L and height h. What is the speed of its center of mass when the cylinder reaches its bottom
View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane without slipping. THE speed of its centre of mass when it reaches the bottom is
View Solution
Knowledge Check
A hollow sphere rolls down a 30∘ incline of length 6m without slipping. The speed of centre of mass at the bottom of plane is
A6ms−1B3ms−1C6√2ms−1D3√3ms−1A solid cylinder rolls down from an inclined plane of height h. What is the velocity of the cylinder when it reaches at the bottom of the plane ?
A√2gh3B√2ghC√4gh3D√3gh2The least coefficient of friction for an inclined plane inclined at angle α with horizontal in order that a solid cylinder will roll down without slipping is
A23tanαB27tanαCtanαD57tanα
A solid cylinder rolls without slipping down an inclined plane of height h. The velocity of the cylinder when it reaches the bouom is
View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane of height h without slipping. The speed of its centre when it reaches the bottom is.
View SolutionA solid cylinder of mass M and radius R rolls without slipping down an inclined plane of length L and height h. What is the speed of its centre of mass when the cylinder reaches its bottom ?
View SolutionA solid cylinder of mass M and radius R rolls without slipping down on inclined plane of length L and height h what is the speed of its centre of mass when the cylinder reaches its bottom?
View SolutionA solid cylinder of mass M and radius R rolls without slipping down on inclined plane of length L and height h what is the speed of its centre of mass when the cylinder reaches its bottom?
View Solution
