Find the reflex angle corresponding to each of the following angles:
53∘
The correct Answer is:307∘
To find the reflex angle corresponding to the angle of 53∘, we can follow these steps:
1. Understand the Definition of Reflex Angle:
A reflex angle is an angle that is greater than 180∘ but less than 360∘. It is the larger angle formed when two lines intersect.
2. Use the Formula for Reflex Angle:
The reflex angle can be calculated using the formula:
Reflex Angle=360∘−Given Angle
3. Substitute the Given Angle:
In this case, the given angle is 53∘. Substitute 53∘ into the formula:
Reflex Angle=360∘−53∘
4. Perform the Calculation:
Now, subtract 53 from 360:
360∘−53∘=307∘
5. State the Final Answer:
Therefore, the reflex angle corresponding to 53∘ is:
307∘
Final Answer:
The reflex angle corresponding to 53∘ is 307∘.
---
A reflex angle is an angle that is greater than 180∘ but less than 360∘. It is the larger angle formed when two lines intersect.
The reflex angle can be calculated using the formula:
Reflex Angle=360∘−Given Angle
In this case, the given angle is 53∘. Substitute 53∘ into the formula:
Reflex Angle=360∘−53∘
Now, subtract 53 from 360:
360∘−53∘=307∘
Therefore, the reflex angle corresponding to 53∘ is:
307∘
The reflex angle corresponding to 53∘ is 307∘.
Similar Questions
Knowledge Check
- Question 1 - Select One
Name each of the following angles
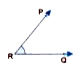 A∠QPRB∠RQPC∠RPQD∠PRQ
A∠QPRB∠RQPC∠RPQD∠PRQ - Question 2 - Select One
Name each of the following angles
 A∠YXZB∠XYZC∠YZXD∠ZYX
A∠YXZB∠XYZC∠YZXD∠ZYX - Question 3 - Select One
Name each of the following angles
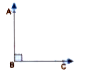 A∠BACB∠ABCC∠BCAD∠CAB
A∠BACB∠ABCC∠BCAD∠CAB
Find the reflex angle corresponding to each of the following angles:
90∘View SolutionFind the reference angles corresponding to each of the following angles. It may help if you sketch θ in standard position. θ=−2300 (ii) 31π9 (iii) θ=6400
View SolutionFind the reference angles corresponding to each of the following angles. It may help if you sketch θ in standard position. θ=−230∘ (ii)31π9 (iii)θ=640∘
View SolutionState the kind of each of the following angles:
View SolutionFind the complement of each of the following angles:
View Solution

