Find the complementary angle of each of the following angles:
70∘
The correct Answer is:20∘
To find the complementary angle of 70∘, we can follow these steps:
Step 1: Understand the concept of complementary angles
Complementary angles are two angles whose sum is 90∘. If we have an angle A and its complementary angle B, then:
A+B=90∘
Step 2: Set up the equation
In this case, we have:
70∘+(complementary angle)=90∘
Step 3: Isolate the complementary angle
To find the complementary angle, we can rearrange the equation:
(complementary angle)=90∘−70∘
Step 4: Perform the subtraction
Now, we calculate:
(complementary angle)=90∘−70∘=20∘
Step 5: State the final answer
Thus, the complementary angle of 70∘ is:
Complementary angle=20∘
---
Complementary angles are two angles whose sum is 90∘. If we have an angle A and its complementary angle B, then:
A+B=90∘
In this case, we have:
70∘+(complementary angle)=90∘
To find the complementary angle, we can rearrange the equation:
(complementary angle)=90∘−70∘
Now, we calculate:
(complementary angle)=90∘−70∘=20∘
Thus, the complementary angle of 70∘ is:
Complementary angle=20∘
Similar Questions
Knowledge Check
Name each of the following angles
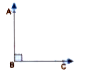 A∠BACB∠ABCC∠BCAD∠CAB
A∠BACB∠ABCC∠BCAD∠CABName each of the following angles
 A∠YXZB∠XYZC∠YZXD∠ZYX
A∠YXZB∠XYZC∠YZXD∠ZYXName each of the following angles
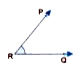 A∠QPRB∠RQPC∠RPQD∠PRQ
A∠QPRB∠RQPC∠RPQD∠PRQ
Find the supplementary angle of each of the following angles:
27∘
View SolutionFind the complementary angle of each of the following angles:
13 right angle
View SolutionFind the complementary angle of each of the following angles:
13 of 60∘
View SolutionFind the complementary angle of each of the following angles:
25 of 175∘
View SolutionFind the complementary angle of each of the following angles:
14 of 124∘
View Solution
