Add : 3ax+5by−cz,by−6ax−3czand5cz−ax−2by.
The correct Answer is:−4ax+4by+cz
To solve the problem of adding the algebraic expressions 3ax+5by−cz, by−6ax−3cz, and 5cz−ax−2by, we will follow these steps:
Step 1: Write down the expressions
We have three expressions to add:
1. 3ax+5by−cz
2. by−6ax−3cz
3. 5cz−ax−2by
Step 2: Combine like terms
To add these expressions, we will group the like terms together. The like terms are those that have the same variable part.
- For ax terms: 3ax, −6ax, and −ax
- For by terms: 5by, by, and −2by
- For cz terms: −cz, −3cz, and 5cz
Step 3: Add the ax terms
3ax−6ax−ax=(3−6−1)ax=−4ax
Step 4: Add the by terms
5by+by−2by=(5+1−2)by=4by
Step 5: Add the cz terms
−cz−3cz+5cz=(−1−3+5)cz=1cz=cz
Step 6: Combine all results
Now, we combine the results from each step:
−4ax+4by+cz
Final Answer
The sum of the given expressions is:
−4ax+4by+cz
We have three expressions to add:
1. 3ax+5by−cz
2. by−6ax−3cz
3. 5cz−ax−2by
To add these expressions, we will group the like terms together. The like terms are those that have the same variable part.
- For by terms: 5by, by, and −2by
- For cz terms: −cz, −3cz, and 5cz
3ax−6ax−ax=(3−6−1)ax=−4ax
5by+by−2by=(5+1−2)by=4by
−cz−3cz+5cz=(−1−3+5)cz=1cz=cz
Now, we combine the results from each step:
−4ax+4by+cz
The sum of the given expressions is:
−4ax+4by+cz
Topper's Solved these Questions
Similar Questions
if a>b>c and the system of equations ax+by+cz=0,bx+cy+az0andcx+ay+bz=0 has a non-trivial solution, then the quadratic equation ax2+bx+c=0 has
View SolutionConsider the linear equations ax+by+cz=0,bx+cy+az=0andcx+ay+bz=0.
Match the conditions/expressions in Column I with statements in Column II.
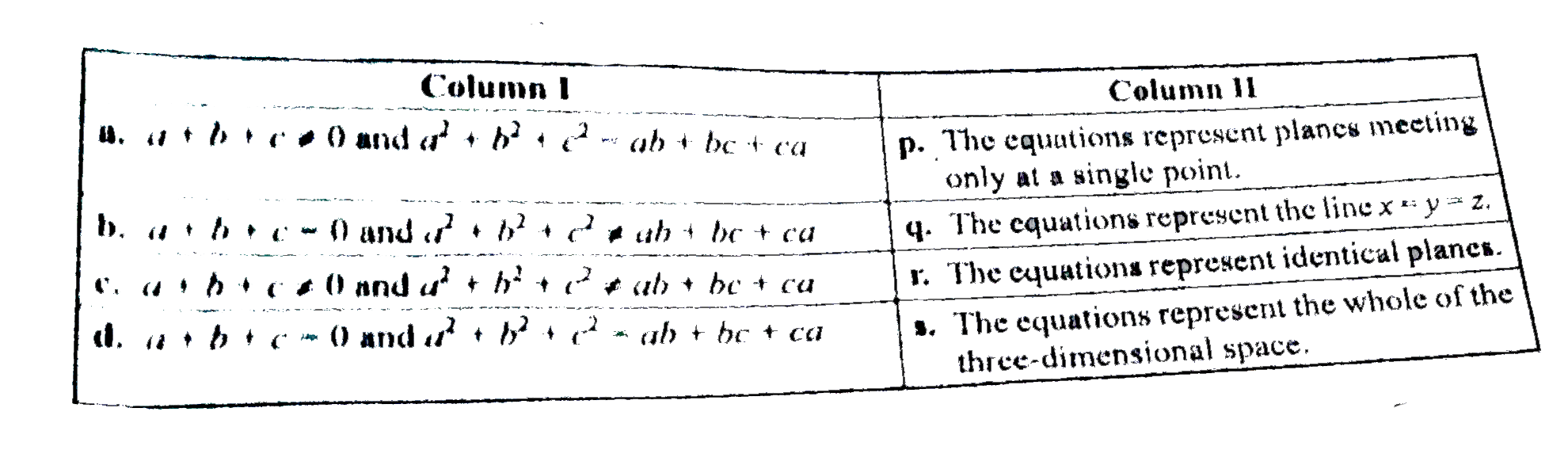 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Add −34 and 56
A14B13C112D712 - Question 2 - Select One
If a, b and c are three different numbers and ax:by:cz=1:2:−3, then ax+by+cz=
A0B1/2C3D6 - Question 3 - Select One
distance between the parallel planes ax+by+cz+d=0andax+by+cz+d'=0 is
A|d+d|√a2+b2+c2B|d−d'|√a2+b2+c2Cd√a2+b2+c2Dnone of these
STATEMENT-1 : If a,b,c are distinct and x,y,z are not all zero and ax+by+cz=0,bx+cy+az=0,cx+ay+bz=0, then a+b+c=0 and STATEMENT-2 : a2+b2+c2>ab+bc+ca, if a,b,c are distinct.
View SolutionIf a+b+c=0anda2+b2+c2−ab−bc−ca≠0,∀a,b,c∈R then the system of equations
ax+by+cz=0,bx+cy+az=0 and cx+ay+bz=0 hasView SolutionIf x,y,z are not all zero & if ax+by+cz=0,bx+cy+az=0&cx+ay+bz=0, then prove that x:y:z=1:1:1 OR 1:ω:ω2OR1:ω2:ω , where ω is one ofthe complex cube root of unity.
View SolutionIf a>b>c and the system of equtions ax+by+cz=0, bx+cy+az=0, cx+ay+bz=0 has a non-trivial solution then both the roots of the quadratic equation at2+bt+c are
View SolutionIf x,y and z are in A.P ax,by and cz in G.P and a, b, c in H.P then prove that xz+zx=ac+ca
View Solution

