Prove that the loss in weight of a body when immersed wholly or partially in a liquid is equal to the buoyant force (or upthrust) and this loss is because of the difference in pressure exerted by liquid on the upper and lower surfaces of the submerged part of the body.
To prove that the loss in weight of a body when immersed wholly or partially in a liquid is equal to the buoyant force (or upthrust), we can follow these steps:
Step 1: Understanding Weight and Buoyant Force
- The weight of a body is the force due to gravity acting on it, which is directed downwards.
- When a body is immersed in a fluid, it experiences an upward force known as the buoyant force or upthrust.
Step 2: Archimedes' Principle
- According to Archimedes' Principle, when a body is immersed in a fluid, it experiences an upward buoyant force equal to the weight of the fluid displaced by the body.
- This principle applies whether the body is fully or partially submerged.
Step 3: Analyzing Pressure Differences
- The pressure exerted by a fluid increases with depth. Therefore, the pressure on the lower surface of the submerged part of the body is greater than the pressure on the upper surface.
- If we denote the depth of the upper surface as h1 and the depth of the lower surface as h2, the pressure difference can be expressed as:
ΔP=Plower−Pupper=ρgh2−ρgh1=ρg(h2−h1)
where ρ is the density of the fluid and g is the acceleration due to gravity.
Step 4: Calculating the Buoyant Force
- The buoyant force Fb can be calculated by multiplying the pressure difference by the area A of the submerged surface:
Fb=ΔP⋅A=ρg(h2−h1)⋅A
- The volume V of the submerged part of the body is given by V=A⋅(h2−h1). Thus, we can rewrite the buoyant force as:
Fb=ρgV
Step 5: Relating Buoyant Force to Loss of Weight
- The weight of the fluid displaced (which is equal to the buoyant force) can also be expressed as:
Wdisplaced=Volume of fluid displaced×Density of fluid×g=V⋅ρ⋅g
- According to Archimedes' principle, the loss in weight of the body when immersed in the fluid is equal to the weight of the fluid displaced:
Loss in weight=Wdisplaced=Fb
Conclusion
- Therefore, we have proved that the loss in weight of a body when immersed wholly or partially in a liquid is equal to the buoyant force (or upthrust), and this loss is due to the difference in pressure exerted by the liquid on the upper and lower surfaces of the submerged part of the body.
- The weight of a body is the force due to gravity acting on it, which is directed downwards.
- When a body is immersed in a fluid, it experiences an upward force known as the buoyant force or upthrust.
- According to Archimedes' Principle, when a body is immersed in a fluid, it experiences an upward buoyant force equal to the weight of the fluid displaced by the body.
- This principle applies whether the body is fully or partially submerged.
- The pressure exerted by a fluid increases with depth. Therefore, the pressure on the lower surface of the submerged part of the body is greater than the pressure on the upper surface.
- If we denote the depth of the upper surface as h1 and the depth of the lower surface as h2, the pressure difference can be expressed as:
ΔP=Plower−Pupper=ρgh2−ρgh1=ρg(h2−h1)
where ρ is the density of the fluid and g is the acceleration due to gravity.
- The buoyant force Fb can be calculated by multiplying the pressure difference by the area A of the submerged surface:
Fb=ΔP⋅A=ρg(h2−h1)⋅A
- The volume V of the submerged part of the body is given by V=A⋅(h2−h1). Thus, we can rewrite the buoyant force as:
Fb=ρgV
- The weight of the fluid displaced (which is equal to the buoyant force) can also be expressed as:
Wdisplaced=Volume of fluid displaced×Density of fluid×g=V⋅ρ⋅g
- According to Archimedes' principle, the loss in weight of the body when immersed in the fluid is equal to the weight of the fluid displaced:
Loss in weight=Wdisplaced=Fb
- Therefore, we have proved that the loss in weight of a body when immersed wholly or partially in a liquid is equal to the buoyant force (or upthrust), and this loss is due to the difference in pressure exerted by the liquid on the upper and lower surfaces of the submerged part of the body.
Topper's Solved these Questions
- UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONBOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(A) (Multiple Choice Question)
3 Videos
- UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONBOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(A) (Numerical)
10 Videos
- UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONBOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(c) (Numerical)
9 Videos
- SOUNDBOOK - ICSECHAPTER - SOUNDEXERCISE - TOPIC 2 Infrasonic and Ultrasonic Waves ( 3 Marks Questions)
3 Videos
Similar Questions
For a body immersed in a liquid, when the weight of the body is less than the upthrust then the body will
View SolutionThe loss of weight of body is 10 N when body is completely immersed in water, then the amount of water displaced by that body is
View SolutionA : The apparent weight of a body floating on the surface of a liquid is zero.
R : The net force on a body floating on the surface of a liquid is zero.
View SolutionThe loss of weight of a solid when immersed in a liquid at 0∘C is W0 and at t∘C is 'W'. If cubical coefficient of expansion of the solid and the liquid are γs and γ1 then W=
View SolutionThe loss of weight of a solid when immersed in a liquid at 0∘C is W0 and at t∘C is 'W'. If cubical coefficient of expansion of the solid and the liquid are γs and γ1 then W=
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.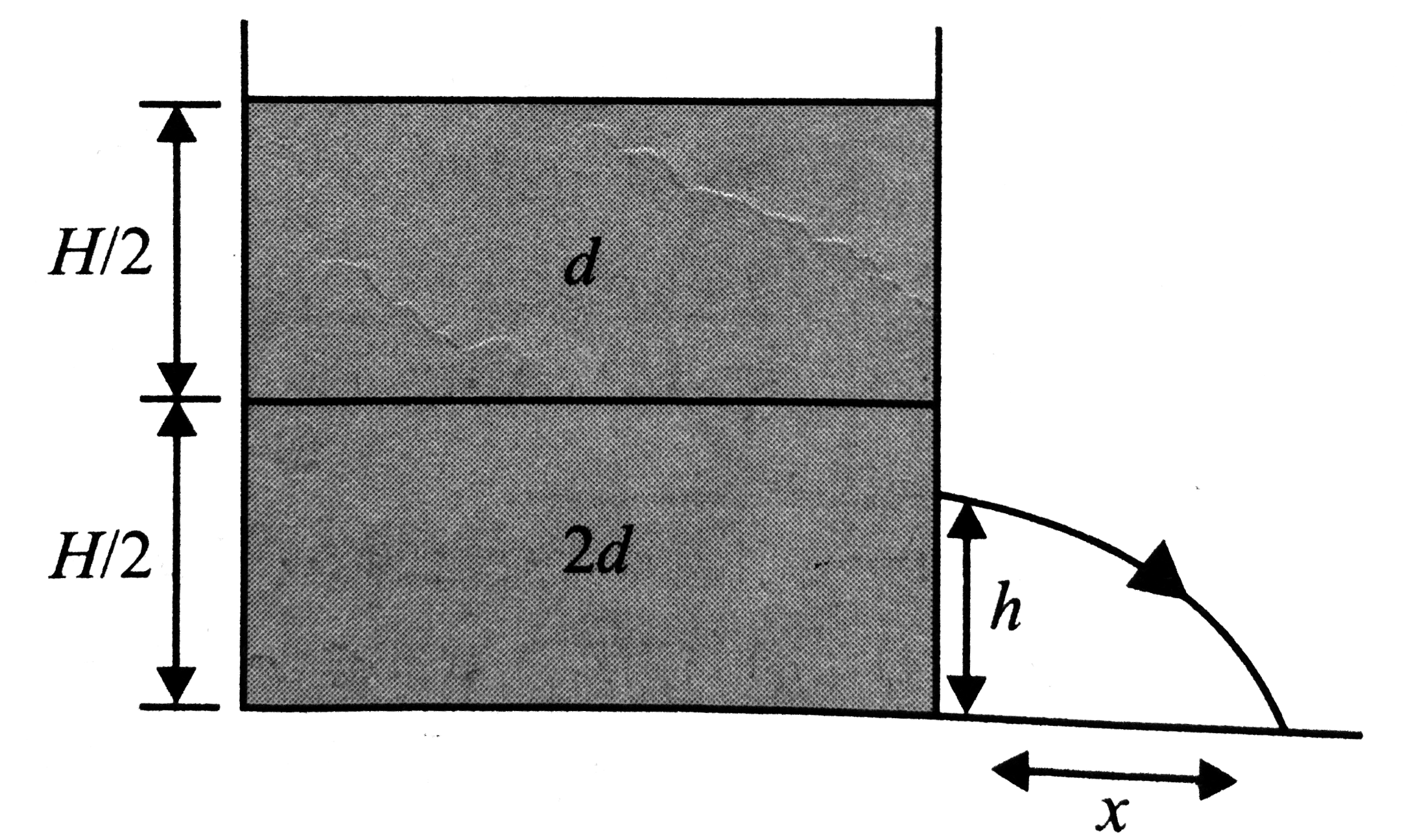
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The total pressure at the bottom of the container is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the Body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.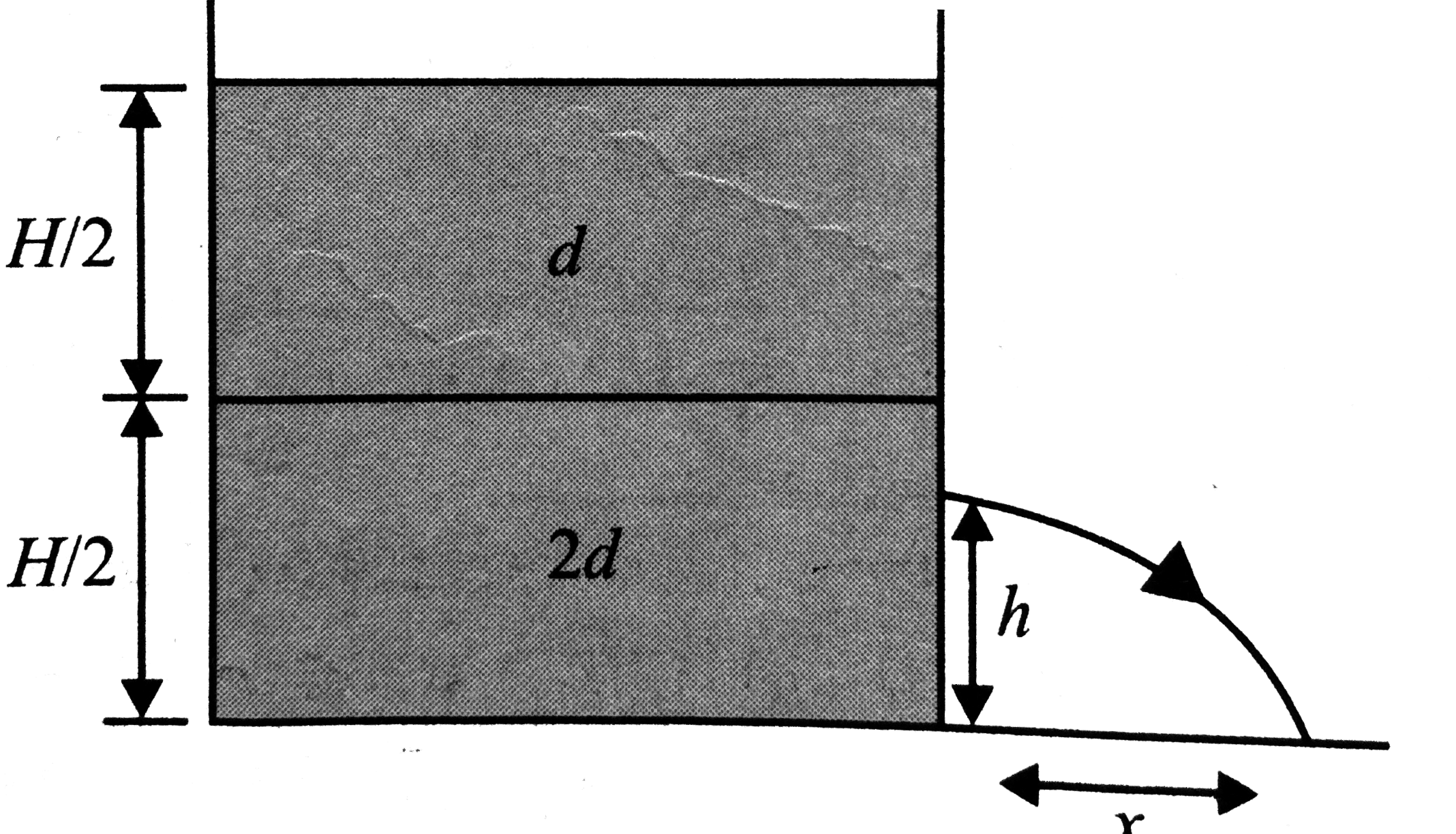
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The density of the solid is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform cross sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.
Situation II:
A cyliner is removed and the original arrangement is restored.A tiny hole of area s(s<<A) is punched on the veritical sideof the containier at a height h(h<H/2)
The initial speed of efflux of the liquid at the hole is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.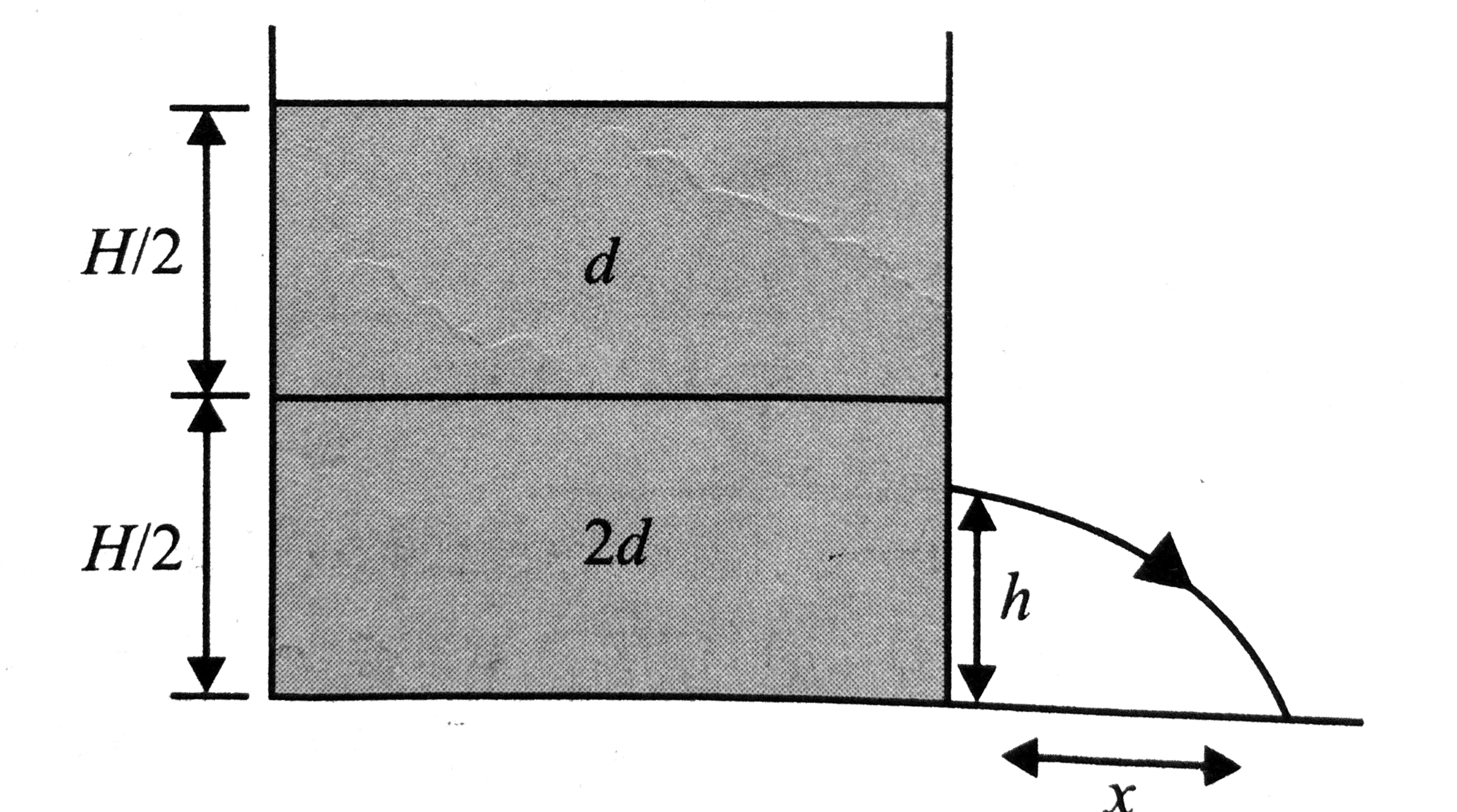
Situation II:
A cyliner is removed and the original arrangement is restoreed.A tiny hole of area s(s<<A) is punched on the veritical sideof the containier at a height h(h<H/2)
The horizontal distance x travelled by the liquid is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.
Situation II:
A cyliner is removed and the original arrangement is restoreed.A tiny hole of area s(s<<A) is punched on the veritical side of the containier at a height h(h<H/2)
The height hm at which the hole should be punched so that the liquid travels the maximum distance is
View Solution
