If 0∘<θ<90∘ and cosθ=45 find the values of
cos(90∘+θ)
The correct Answer is:−35
To solve the problem, we need to find the value of cos(90∘+θ) given that cosθ=45 and 0∘<θ<90∘.
1. Use the Cosine Addition Formula:
We know that:
cos(90∘+θ)=cos90∘cosθ−sin90∘sinθ
2. Substitute Known Values:
We know that:
cos90∘=0andsin90∘=1
Substituting these values into the formula gives:
cos(90∘+θ)=0⋅cosθ−1⋅sinθ
This simplifies to:
cos(90∘+θ)=−sinθ
3. Find sinθ:
We can use the Pythagorean identity:
sin2θ+cos2θ=1
Substituting cosθ=45:
sin2θ+(45)2=1
This simplifies to:
sin2θ+1625=1
Rearranging gives:
sin2θ=1−1625
Finding a common denominator:
sin2θ=2525−1625=925
4. Calculate sinθ:
Taking the square root:
sinθ=√925=35
Since θ is in the first quadrant, sinθ is positive.
5. Substitute Back to Find cos(90∘+θ):
Now substituting sinθ back into the equation for cos(90∘+θ):
cos(90∘+θ)=−sinθ=−35
Final Answer:
cos(90∘+θ)=−35
We know that:
cos(90∘+θ)=cos90∘cosθ−sin90∘sinθ
We know that:
cos90∘=0andsin90∘=1
Substituting these values into the formula gives:
cos(90∘+θ)=0⋅cosθ−1⋅sinθ
This simplifies to:
cos(90∘+θ)=−sinθ
We can use the Pythagorean identity:
sin2θ+cos2θ=1
Substituting cosθ=45:
sin2θ+(45)2=1
This simplifies to:
sin2θ+1625=1
Rearranging gives:
sin2θ=1−1625
Finding a common denominator:
sin2θ=2525−1625=925
Taking the square root:
sinθ=√925=35
Since θ is in the first quadrant, sinθ is positive.
Now substituting sinθ back into the equation for cos(90∘+θ):
cos(90∘+θ)=−sinθ=−35
cos(90∘+θ)=−35
Topper's Solved these Questions
- TRIGONOMETRICAL FUNCTIONS BOOK - ICSECHAPTER - TRIGONOMETRICAL FUNCTIONS EXERCISE - Chapter test
8 Videos
- TRIGONOMETRICAL FUNCTIONS BOOK - ICSECHAPTER - TRIGONOMETRICAL FUNCTIONS EXERCISE - Exercise 4(c)
12 Videos
- TRIGONOMETRIC EQUATIONS BOOK - ICSECHAPTER - TRIGONOMETRIC EQUATIONS EXERCISE - CHAPTER TEST
6 Videos
Similar Questions
Knowledge Check
If 90∘<θ<180∘ and sinθ=2029, then cosθ = ?
A2920B2021C−2129D−2921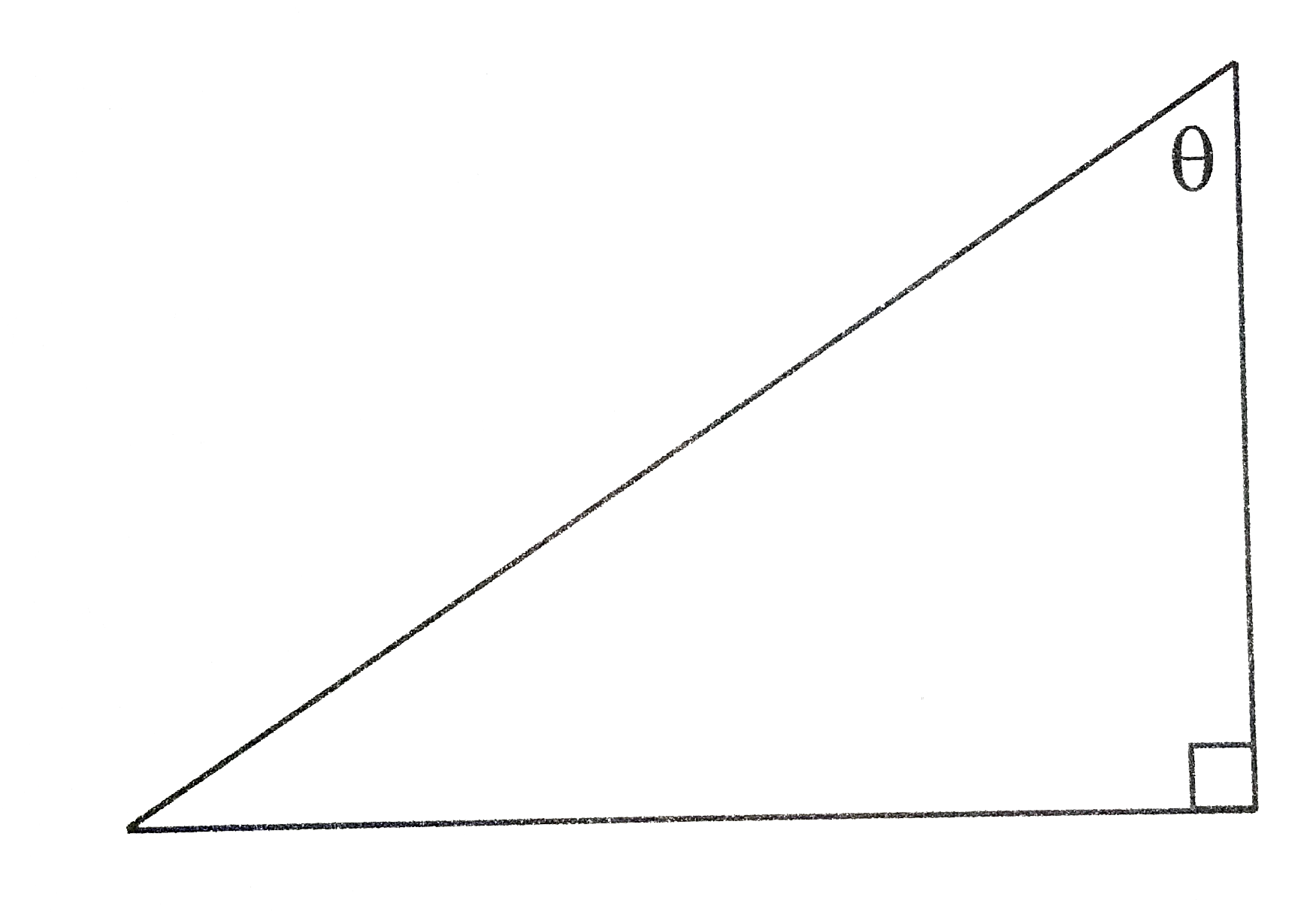
In the figure above, IF cosθ=35, what is the value of cos(90−θ)?A35B34C45D54
If 0∘<θ<90∘ and cosθ=45 find the values of
tan(360∘−θ)
View SolutionGiven : 4sinθ=3cosθ , find the value of:
cosθ
View SolutionIf 3sinθ+4cosθ=5, then find the value of 4sinθ−3cosθ.
View SolutionSolve for θ(0∘<θ<90∘)
2cos3θ=1
View SolutionIf 4sin2θ−1=0 and angle θ is less than 90∘, find the value of θ and hence the value of cos2θ+tan2θ.
View SolutionIf 4sin2θ−1=0 and angle θ is less than 90∘, find the value of θ and hence the value of cos2θ+tan2θ.
View Solution
