How many diagonals are there in an n- sided polygen (n>3)?
To find the number of diagonals in an n-sided polygon (where n > 3), we can follow these steps:
Step 1: Understand the Problem
A polygon with n sides has n vertices. We need to find the number of diagonals that can be formed using these vertices.
Step 2: Calculate the Total Number of Line Segments
To determine the total number of line segments that can be formed by connecting any two vertices, we can use the combination formula. The number of ways to choose 2 vertices from n vertices is given by:
Total line segments=(n2)=n(n−1)2
Step 3: Identify the Sides of the Polygon
In an n-sided polygon, there are exactly n sides. These sides are also counted in the total line segments calculated in Step 2.
Step 4: Calculate the Number of Diagonals
To find the number of diagonals, we need to subtract the number of sides from the total number of line segments:
Number of diagonals=Total line segments−Number of sides
Substituting the values we found:
Number of diagonals=n(n−1)2−n
Step 5: Simplify the Expression
Now, we simplify the expression:
Number of diagonals=n(n−1)2−2n2=n(n−1)−2n2=n2−n−2n2=n2−3n2
Final Result
Thus, the number of diagonals in an n-sided polygon is:
Number of diagonals=n(n−3)2
A polygon with n sides has n vertices. We need to find the number of diagonals that can be formed using these vertices.
To determine the total number of line segments that can be formed by connecting any two vertices, we can use the combination formula. The number of ways to choose 2 vertices from n vertices is given by:
In an n-sided polygon, there are exactly n sides. These sides are also counted in the total line segments calculated in Step 2.
To find the number of diagonals, we need to subtract the number of sides from the total number of line segments:
Now, we simplify the expression:
Thus, the number of diagonals in an n-sided polygon is:
Similar Questions
Knowledge Check
How many different triangles are there for which the lengths of the sides are 3, 8, and n, where n is an integer and 3<n<8 ?
ATwoBThreeCFourDFive
Write the number of diagonals of an n-sided polygon.
View SolutionHow many σ−bonds are present in N2O3 ?
View SolutionHow many rings are present in [MEDTA]+n ?
View SolutionHow many N atom are present in final product.
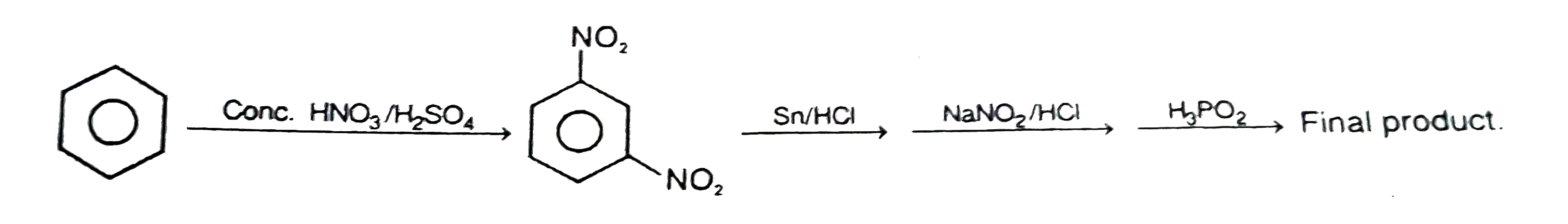
View SolutionHow many orbitals are possible for n = 3, l=2 ?
View SolutionHow many triangles can be formed by joining the vertices of an n- sided polygen?
View SolutionHow many different diagonal matrices of order n can be formed which are involuntary ?
View Solution
