Find the value of :
50C47
The correct Answer is:19600
To find the value of (5047), we will use the formula for combinations, which is given by:
nCr=n!r!(n−r)!
1. Identify the values of n and r:
Here, n=50 and r=47.
2. Apply the combination formula:
Using the formula, we have:
(5047)=50!47!(50−47)!=50!47!⋅3!
3. Simplify the factorials:
We can express 50! as:
50!=50×49×48×47!
Therefore, substituting this back into our equation gives:
(5047)=50×49×48×47!47!⋅3!
4. Cancel out the 47!:
The 47! in the numerator and denominator cancels out:
(5047)=50×49×483!
5. Calculate 3!:
We know that:
3!=3×2×1=6
6. Substitute 3! back into the equation:
Thus, we have:
(5047)=50×49×486
7. Perform the multiplication:
First, calculate 50×49×48:
50×49=2450
2450×48=117600
8. Divide by 6:
Now, divide 117600 by 6:
1176006=19600
Final Answer:
Thus, the value of (5047) is 19600.
Here, n=50 and r=47.
Using the formula, we have:
(5047)=50!47!(50−47)!=50!47!⋅3!
We can express 50! as:
50!=50×49×48×47!
Therefore, substituting this back into our equation gives:
(5047)=50×49×48×47!47!⋅3!
The 47! in the numerator and denominator cancels out:
(5047)=50×49×483!
We know that:
3!=3×2×1=6
Thus, we have:
(5047)=50×49×486
First, calculate 50×49×48:
50×49=2450
2450×48=117600
Now, divide 117600 by 6:
1176006=19600
Thus, the value of (5047) is 19600.
Similar Questions
Find the value of :
5C2
View SolutionFind the value of :
10C4
View SolutionFind the values of A,B and C
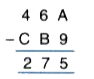
View SolutionFind the value of 47C4+5∑r=152−rC3
View SolutionFind the value of :50C4+6∑r=1.56−rC3.
View SolutionFind the value of ?
3,2=7
5,4=23
7,6=47
9,8=79
10,9=?
View SolutionIn previous, Find the value of γ=CP/Cv:
View SolutionFind the value of: 50−(−48)−(−2)−110
View SolutionFind the value of x, if: (i) 4x=(52)2−(48)2 (ii) 14x=(47)2−(33)2 (iii) 5x=(50)2−(40)2
View SolutionFind the highest power of 3 in 50C10and50P10
View Solution
