A car starting from rest accelerates uniformly to acquire a speed 20 km h−1 in 30 min. The distance travelled by car in this time interval will be :
The correct Answer is:600 km
To solve the problem step by step, we will follow the physics concepts related to uniformly accelerated motion.
Step 1: Identify the given data
- Initial speed (u) = 0 km/h (since the car starts from rest)
- Final speed (v) = 20 km/h
- Time (t) = 30 minutes = 0.5 hours (since we need to convert minutes to hours)
Step 2: Convert final speed to consistent units
Since we are working with time in hours, we can keep the speed in km/h. Thus, we do not need to convert the final speed.
Step 3: Use the formula to find acceleration
We will use the formula for final velocity in uniformly accelerated motion:
v=u+at
Where:
- v = final velocity
- u = initial velocity
- a = acceleration
- t = time
Substituting the known values:
20=0+a×0.5
Now, solving for acceleration (a):
20=0.5a
a=200.5=40 km/h2
Step 4: Calculate the distance traveled using the distance formula
We will use the formula for distance (s) in uniformly accelerated motion:
s=ut+12at2
Substituting the known values:
- u = 0 km/h
- a = 40 km/h²
- t = 0.5 hours
Now, substituting into the formula:
s=0×0.5+12×40×(0.5)2
s=0+12×40×0.25
s=0+408
s=5 km
Conclusion
The distance traveled by the car in the time interval of 30 minutes is 5 kilometers.
- Initial speed (u) = 0 km/h (since the car starts from rest)
- Final speed (v) = 20 km/h
- Time (t) = 30 minutes = 0.5 hours (since we need to convert minutes to hours)
Since we are working with time in hours, we can keep the speed in km/h. Thus, we do not need to convert the final speed.
We will use the formula for final velocity in uniformly accelerated motion:
v=u+at
Where:
- v = final velocity
- u = initial velocity
- a = acceleration
- t = time
20=0+a×0.5
20=0.5a
a=200.5=40 km/h2
We will use the formula for distance (s) in uniformly accelerated motion:
s=ut+12at2
Substituting the known values:
- u = 0 km/h
- a = 40 km/h²
- t = 0.5 hours
s=0×0.5+12×40×(0.5)2
s=0+12×40×0.25
s=0+408
s=5 km
The distance traveled by the car in the time interval of 30 minutes is 5 kilometers.
Topper's Solved these Questions
- MOTION IN ONE DIMENSION BOOK - ICSECHAPTER - MOTION IN ONE DIMENSION EXERCISE - EXERCISE -2 (C)
3 Videos
- MEASUREMENTS AND EXPERIMENTATION BOOK - ICSECHAPTER - MEASUREMENTS AND EXPERIMENTATION EXERCISE - TOPIC 2 SIMPLE PENDULUM (4 Marks Questions )
1 Videos
- PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREBOOK - ICSECHAPTER - PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREEXERCISE - Exercise -4(B)Numericals
5 Videos
Similar Questions
A car starts from rest and accelerates uniformly to a speed of 180kmh−1 in 10 s. The distance covered by the car in the time interval is
View SolutionIf a car, initially at rest, accelerates uniformly to a speed of 50ms−1 in 25s, distance travelled by it is
View SolutionIf a car at rest, accelerates uniformly to a speed of 144km/h in 20s, it covers a distance of
View SolutionA car travels for 20 min with a constant speed of 54 km h−1 Find the distance travelled by the car.
View SolutionA car starting from rest acquires a velocity 180 m s−1 in 0.05 h. Find the acceleration.
View SolutionA car starts form rest and accelerates uniformly for 10s to a velocity of 8ms−1. It then runs at a constant velocity an dis finally brought to rest in 64m with a constant retardation. The totla distance covered by the car is 584m Find the value of acceleration, retardation, and totl time taken.
View SolutionA car starting from rest acquires a velocity of 54kmh−1 min 20 s.
Calculate the acceleration of the car in S.I. unit.
View SolutionA car acquires a velocity of 72 km h−1 In 10 s starting from rest. Calculate :
(i) the acceleration,
(ii) the average velocity, and
(iii) the distance travelled in this time.
View SolutionA car, starting from rest, has a constant acceleration for a time interval t1 during which it covers a distance In the next time interval the car has a constant retardation and comes to rest after covering a distance in time Which of the following relations is correct?
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
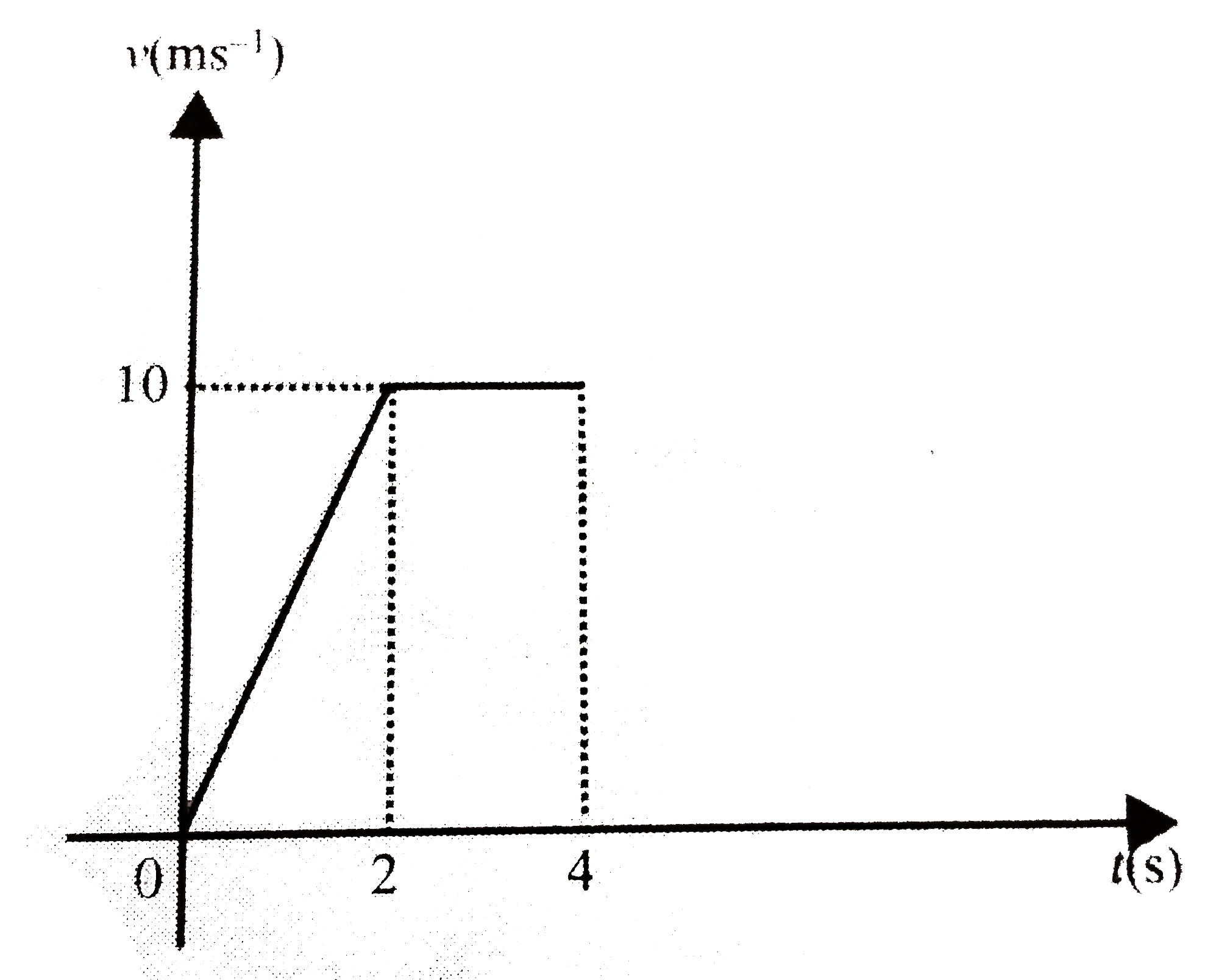
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View Solution
