In a bundle of 50 shirts, 44 are good, 4 have minor defects and 2 have major defects. What is the probability that :
(ii) it is acceptable to a trader who rejects only a shirt with major defects ?
To find the probability that a shirt is acceptable to a trader who rejects only shirts with major defects, we can follow these steps:
1. Identify Total Shirts:
- We have a total of 50 shirts in the bundle.
2. Classify the Shirts:
- Out of these shirts:
- Good shirts: 44
- Shirts with minor defects: 4
- Shirts with major defects: 2
3. Determine Acceptable Shirts:
- The trader accepts shirts that are either good or have minor defects.
- Therefore, the number of acceptable shirts = Good shirts + Minor defect shirts = 44 + 4 = 48.
4. Calculate Total Outcomes:
- The total number of shirts is 50.
5. Calculate Probability:
- The probability (P) that a shirt is acceptable to the trader is given by the formula:
P(acceptable)=Number of acceptable shirtsTotal number of shirts=4850
6. Simplify the Probability:
- Simplifying 4850:
P(acceptable)=48÷250÷2=2425
7. Final Answer:
- The probability that a shirt is acceptable to the trader is 2425.
- We have a total of 50 shirts in the bundle.
- Out of these shirts:
- Good shirts: 44
- Shirts with minor defects: 4
- Shirts with major defects: 2
- The trader accepts shirts that are either good or have minor defects.
- Therefore, the number of acceptable shirts = Good shirts + Minor defect shirts = 44 + 4 = 48.
- The total number of shirts is 50.
- The probability (P) that a shirt is acceptable to the trader is given by the formula:
P(acceptable)=Number of acceptable shirtsTotal number of shirts=4850
- Simplifying 4850:
P(acceptable)=48÷250÷2=2425
- The probability that a shirt is acceptable to the trader is 2425.
Topper's Solved these Questions
Similar Questions
In a bundle of 50 shirts, 44 are good, 4 have minor defects and 2 have major defects. What is the probability that a shirt is acceptable to a trader who accepts only a good shirt ?
View SolutionIn a bundle of 50 shirts, 44 are good, 4 have minor defects and 2 have major defects. What is the probability that :
(i) it is acceptable to a trader who accepts only a good shirt .
(ii) it is acceptable to a trader who reject only a shirt with major defects ?
View Solution
Knowledge Check
Minor defects are found on 7 to 10 new cars. If 3 of the 10 cars are selectred at random , what is the probability that 2 have minor defects ?
A0.143B0.333C0.525D0.667
A lot consists of 48 mobile phones of which 42 are good, 3 have only minor defected and 3 have major defects. Varnika will buy a phone if its is good but the trader will only buy a mobile, if it has no major defect. One phone is selected at random from the lot. What is the probability that t is
(i) accetable to Varnika?
(ii) acceptable to the trader.
View SolutionA carton consists of 100 shirts of which 88 are good, 8 have minor defects and 4 have major defects. Jimmy, a trader, will only accept the shirts which are good, but Sujatha, another trader, will only reject the shirts which have major defects. One shirt is drawn at random from the carton. What is the probability that it is acceptable to (i) Jimmy? (ii) Sujatha?
View SolutionA carton consists of 100 shirts of which 88 are good. 8 have minor defects and 4 have major defects. Jimmy, a trader, will only accept the shirts which are good, but Sujatha, another trader, will only reject the shirts which have major defects. One shirt is drawn at random from the carton. What is the probability that(i) it is acceptable to Jimmy?(ii) it is acceptable to Sujatha?
View SolutionA lot consists of 12 good pencils , 6 with minor defects and 2 with major defects .A pencil is chosen at random .Find the probability that this pencil is not defective.
View Solution4 Defective Pens Are Accidentally Mixed with 16 Good Ones. It is Not Possible to Just Look at a Pen and Tell Whether Or Not It is Defective. One Pen is Drawn at Random from the Lot
Suppose the pen drawn is defective and is not replaced. Now one more pen is drawn at random from the rest. What is the probability that this pen is : (a) defective ? (b) not defective ?
View Solution(i) A lot of 20 bulbs contain 4 defective ones. One bulb is drawn at random from the lot. What is the probability that this bulb is defective?(ii) Suppose the bulb drawn in (i) is not defective and is not replaced. Now one bulb is drawn at random from the rest. What is the probability that this bulb is not defective ?
View SolutionBulbs are packed in cartons each containing 40 bulbs, seven hundred cartons were examined for defective bulbs and the results are given in the following table.
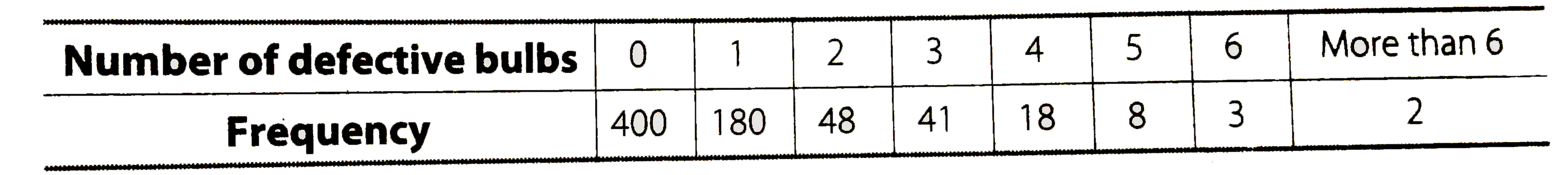
One carton was selected at random. What is the probability that it has
i) no defective bulb?
ii) defective bulbs from 2-6?
iii) defective bulbs less than 4?
View Solution
