State True or False.
If the product of two whole numbers is 1, then at least one of them is one.
The correct Answer is:T
To determine whether the statement "If the product of two whole numbers is 1, then at least one of them is one" is true or false, we can analyze the situation step by step.
Step 1: Understand Whole Numbers
Whole numbers are defined as the set of non-negative integers, which includes 0, 1, 2, 3, and so on.
Hint: Remember that whole numbers start from 0 and include all positive integers.
Step 2: Analyze the Product of Whole Numbers
When we multiply two whole numbers, we obtain a product. We need to check under what conditions this product can equal 1.
Hint: Consider the multiplication table of whole numbers to see what products you can get.
Step 3: Consider Examples
1. Example 1:
- Let’s take the whole numbers 1 and 1.
- The product is: 1×1=1.
- Here, at least one of the numbers is 1.
2. Example 2:
- Now, let’s take another pair, 0 and 1.
- The product is: 0×1=0.
- Here, the product is not 1, so this does not apply.
3. Example 3:
- Let’s take the whole numbers 2 and 3.
- The product is: 2×3=6.
- Again, the product is not 1.
Hint: Check if there are any pairs of whole numbers that can give a product of 1 without one of them being 1.
Step 4: Conclusion
From the examples above, the only way to achieve a product of 1 using whole numbers is when both numbers are 1. If we have two whole numbers and their product is 1, it must be that at least one of them is 1.
Therefore, the statement is True.
Final Answer: True
Whole numbers are defined as the set of non-negative integers, which includes 0, 1, 2, 3, and so on.
When we multiply two whole numbers, we obtain a product. We need to check under what conditions this product can equal 1.
1. Example 1:
- Let’s take the whole numbers 1 and 1.
- The product is: 1×1=1.
- Here, at least one of the numbers is 1.
- Now, let’s take another pair, 0 and 1.
- The product is: 0×1=0.
- Here, the product is not 1, so this does not apply.
- Let’s take the whole numbers 2 and 3.
- The product is: 2×3=6.
- Again, the product is not 1.
From the examples above, the only way to achieve a product of 1 using whole numbers is when both numbers are 1. If we have two whole numbers and their product is 1, it must be that at least one of them is 1.
Similar Questions
State True or False.
If the product of two numbers is zero, then at least one of them is zero.View SolutionThe product of two whole numbers is zero. What do you conclude?
View SolutionThe product of two rational numbers is 25. If one of them is −825 , find the other.
View Solution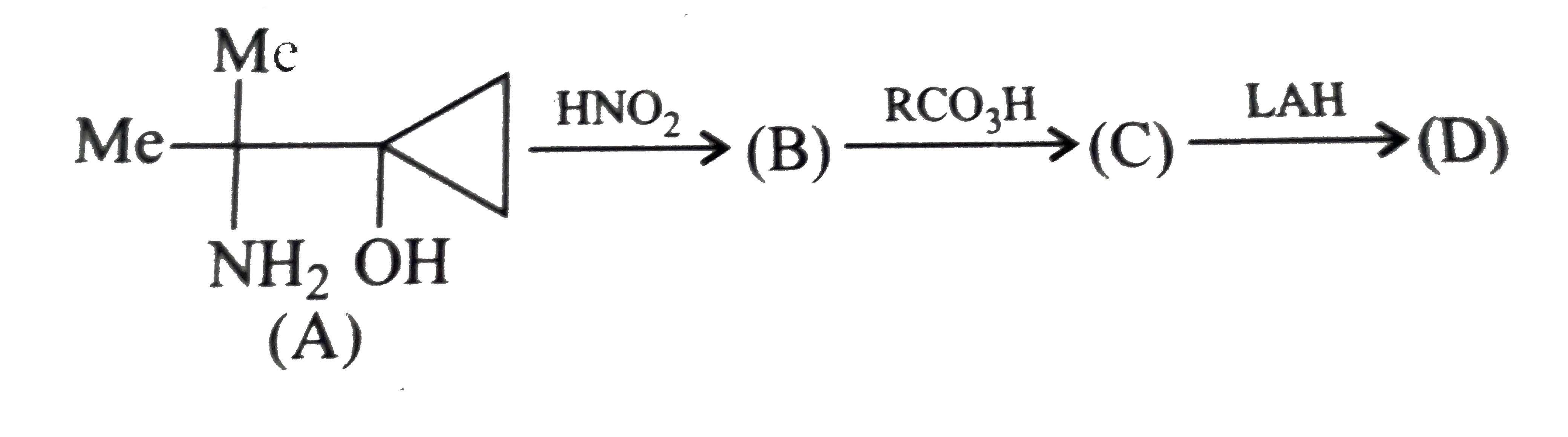
State True or False: Product B is Alcohol.View SolutionThe product of two rational numbers is 4445 If one of them is −119 find the other.
View SolutionThe product of two rational numbers is −2. If one of them is 47, find the other.
View SolutionThe product of two numbers is 52.8 . If one of them is 8.25, find the other .
View SolutionIf the product of two whole numbers is 1, can we say that one or both of them will be 1? Justify through examples.
View SolutionState True or False.
All whole number are natural numbers.View SolutionThe product of two rational numbers is −23. If one of them is 1639, find the other
View Solution

