From the given algbraic expression (2ab)(3ac)−(6c2)(ca)+(2ab)(−ac) complete the following table filling in the required coefficients . Write NA for the reaction that do not apply.
To solve the algebraic expression (2ab)(3ac)−(6c2)(ca)+(2ab)(−ac) and fill in the required coefficients in the table, we will break down each term step by step.
Step 1: Calculate (2ab)(3ac)
1. Multiply the coefficients: 2×3=6
2. Multiply the variables:
- a×a=a2
- b remains as b
- c remains as c
So, (2ab)(3ac)=6a2bc.
Step 2: Calculate −(6c2)(ca)
1. Multiply the coefficients: −6 remains as −6
2. Multiply the variables:
- c2×c=c3
- a remains as a
So, −(6c2)(ca)=−6c3a.
Step 3: Calculate (2ab)(−ac)
1. Multiply the coefficients: 2×−1=−2
2. Multiply the variables:
- a×a=a2
- b remains as b
- c remains as c
So, (2ab)(−ac)=−2a2b.
Step 4: Combine all the terms
Now we combine all the results from the previous steps:
6a2bc−6c3a−2a2b
Step 5: Fill in the coefficients in the table
Now, we will identify the coefficients for each term in the expression:
1. For 6a2bc:
- Coefficient of a: NA (no a term)
- Coefficient of b: 6a2c
- Coefficient of c: 6a2b
- Coefficient of a2: 6bc
- Coefficient of c3: NA (no c3 term)
- Coefficient of bc: 6a2
2. For −6c3a:
- Coefficient of a: −6c3
- Coefficient of b: NA (no b term)
- Coefficient of c: NA (no c term)
- Coefficient of a2: NA (no a2 term)
- Coefficient of c3: −6a
- Coefficient of bc: NA (no bc term)
3. For −2a2b:
- Coefficient of a: NA (no a term)
- Coefficient of b: −2a2
- Coefficient of c: −2a2c
- Coefficient of a2: −2bc
- Coefficient of c3: NA (no c3 term)
- Coefficient of bc: −2a2
Summary of Coefficients
- For 6a2bc:
- Coefficient of a: NA
- Coefficient of b: 6a2c
- Coefficient of c: 6a2b
- Coefficient of a2: 6bc
- Coefficient of c3: NA
- Coefficient of bc: 6a2
- For −6c3a:
- Coefficient of a: −6c3
- Coefficient of b: NA
- Coefficient of c: NA
- Coefficient of a2: NA
- Coefficient of c3: −6a
- Coefficient of bc: NA
- For −2a2b:
- Coefficient of a: NA
- Coefficient of b: −2a2
- Coefficient of c: NA
- Coefficient of a2: −2bc
- Coefficient of c3: NA
- Coefficient of bc: −2a2
2. Multiply the variables:
- a×a=a2
- b remains as b
- c remains as c
2. Multiply the variables:
- c2×c=c3
- a remains as a
2. Multiply the variables:
- a×a=a2
- b remains as b
- c remains as c
- Coefficient of a: NA (no a term)
- Coefficient of b: 6a2c
- Coefficient of c: 6a2b
- Coefficient of a2: 6bc
- Coefficient of c3: NA (no c3 term)
- Coefficient of bc: 6a2
- Coefficient of a: −6c3
- Coefficient of b: NA (no b term)
- Coefficient of c: NA (no c term)
- Coefficient of a2: NA (no a2 term)
- Coefficient of c3: −6a
- Coefficient of bc: NA (no bc term)
- Coefficient of a: NA (no a term)
- Coefficient of b: −2a2
- Coefficient of c: −2a2c
- Coefficient of a2: −2bc
- Coefficient of c3: NA (no c3 term)
- Coefficient of bc: −2a2
- Coefficient of a: NA
- Coefficient of b: 6a2c
- Coefficient of c: 6a2b
- Coefficient of a2: 6bc
- Coefficient of c3: NA
- Coefficient of bc: 6a2
- Coefficient of a: −6c3
- Coefficient of b: NA
- Coefficient of c: NA
- Coefficient of a2: NA
- Coefficient of c3: −6a
- Coefficient of bc: NA
- Coefficient of a: NA
- Coefficient of b: −2a2
- Coefficient of c: NA
- Coefficient of a2: −2bc
- Coefficient of c3: NA
- Coefficient of bc: −2a2
Similar Questions
For the given algebraic expression 4a2bc−3a2bc2+2bc, complete the following table, filling in the required coefficients.
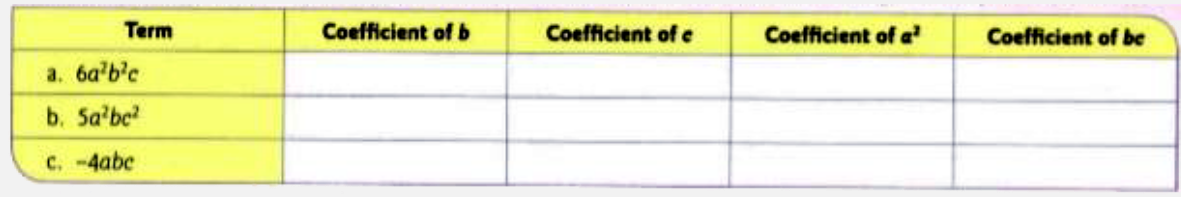
View SolutionFor the given algebraic expression 6a2b2c+5a2bc2−4abc complete the given table filling in the required coefficients.
View SolutionAdd the algebraic expressions.
3ab+6bc−4caand2ab−2bc+3ca
View SolutionAdd the algebraic expressions.
5ab2−5bc+8caand3ab2+6bc−4ca
View SolutionWrite the terms of the following algebraic expressions.
ac+b−2c
View SolutionAdd the following expressions:
2ab+3bc−5ca,4bc−3ab+7ca,2ca−ab−5bc
View SolutionIf the expression x2+2(a+b+c)x+3(bc+ca+ab) is a perfect square then
View SolutionWrite down the numerical coefficient and the literal coefficient of each of the following monomials :
−ab2c3d4
View SolutionLet A(2,−1,4) and B(0,2,−3) be the points and C be a point on AB produced such that 2AC=3AB, then the coordinates of C are
View SolutionWrite all the terms of each of the following algebraic expressions :
3ab−9ab3+5c2−3
View Solution
