Fill in the blanks:
4abc+2a2bc−3b2c−c2+5=abc−c2+5− _______
To solve the equation and fill in the blank, we start with the given expression:
Given:
4abc+2a2bc−3b2c−c2+5=abc−c2+5−_____
Step 1: Isolate the blank term.
To find the term that fills the blank, we can rearrange the equation. We can move the left-hand side (LHS) to the right-hand side (RHS) and set it equal to the blank term.
So we have:
_____=abc−c2+5−(4abc+2a2bc−3b2c−c2+5)
Step 2: Simplify the expression.
Now we simplify the expression on the right-hand side:
_____=abc−c2+5−4abc−2a2bc+3b2c+c2−5
Step 3: Combine like terms.
Now we combine the like terms:
- For abc: abc−4abc=−3abc
- For c2: −c2+c2=0
- For constants: 5−5=0
- The remaining terms: −2a2bc+3b2c
So we have:
_____=−3abc−2a2bc+3b2c
Final Result:
Thus, the term that fills the blank is:
−3abc−2a2bc+3b2c
---
4abc+2a2bc−3b2c−c2+5=abc−c2+5−_____
To find the term that fills the blank, we can rearrange the equation. We can move the left-hand side (LHS) to the right-hand side (RHS) and set it equal to the blank term.
_____=abc−c2+5−(4abc+2a2bc−3b2c−c2+5)
Now we simplify the expression on the right-hand side:
_____=abc−c2+5−4abc−2a2bc+3b2c+c2−5
Now we combine the like terms:
- For abc: abc−4abc=−3abc
- For c2: −c2+c2=0
- For constants: 5−5=0
- The remaining terms: −2a2bc+3b2c
_____=−3abc−2a2bc+3b2c
Thus, the term that fills the blank is:
−3abc−2a2bc+3b2c
Similar Questions
Fill in the blanks:
2abc2+3ab2c−4ab+3bc−2ca−2=2abc2+3bc−2ca−2− _________
View SolutionIf a= 2, b= 3 and c= 4, find the value of : ab+bc+ca−a2−b2−c23abc−a3−b3−c3, using suitable identity
View SolutionIdentify like terms in the following:
abc,ab2c,acb2,c2ab,b2ac,a2bc,cab2
View Solution∣∣ ∣ ∣ ∣∣a2+b2cccab2+c2aabba2+c2b∣∣ ∣ ∣ ∣∣ equal to : (A) 4abc (B) a2+b2+c2 (C) (a+b+c)2 (D) None of These
View SolutionThe value of the determinant ∣∣ ∣ ∣∣1aa2−bc1bb2−ca1cc2−ab∣∣ ∣ ∣∣ is (A) (a+b+c),(a2+b2+c2) (B) a3+b3+c3−3abc (C) (a−b)(b−c)(c−a) (D) 0
View SolutionFactorize the following ∣∣3a+b+ca3+b3+c3a+b+ca2+b2+c2a4+b4+c4a2+b2+c2a3+b3+c3a5+b5+c5∣∣
View SolutionFrom the sum of 4abc+3ab−bc+4and3abc−ab+2bc−2 subtract 2abc+ab−3bc+8
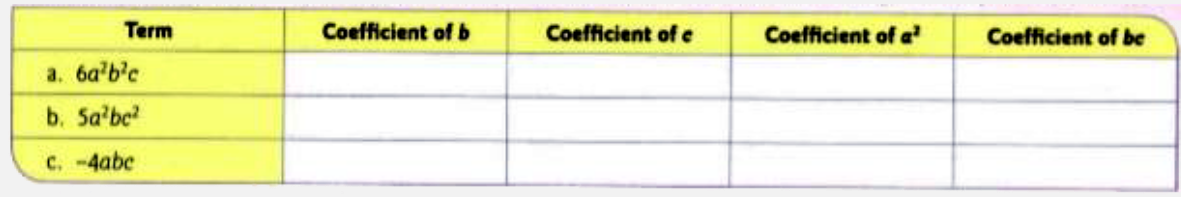
View SolutionFor the given algebraic expression 6a2b2c+5a2bc2−4abc complete the given table filling in the required coefficients.
View SolutionAdd 4a2b−2b2c+3c2a+4abc−5anda2b+b2−2c2+8ab
View SolutionFind the value of :
3abc−2a2+4b3+ac given that a=−2,b=3 and c=−1
View Solution
