Weight of a person on the Earth depends on the gravitational force exerted by the Earth.
To solve the question regarding the weight of a person on Earth and its dependence on gravitational force, we can break it down into the following steps:
Step 1: Understand the Concept of Weight
Weight is defined as the force exerted on an object due to gravity. It is calculated using the formula:
Weight(W)=mass(m)×acceleration due to gravity(g)
Step 2: Identify the Variables
In this formula:
- m is the mass of the person (measured in kilograms).
- g is the acceleration due to gravity on Earth, which is approximately 9.81m/s2.
Step 3: Apply the Formula
When a person stands on the surface of the Earth, their weight can be calculated by multiplying their mass by the acceleration due to gravity:
W=m×g
This means that the weight of the person is directly proportional to their mass and the gravitational acceleration.
Step 4: Conclusion on Dependence
The weight of a person on Earth indeed depends on the gravitational force exerted by the Earth. If the gravitational force changes (for example, if the person were on another planet), their weight would also change. Therefore, the statement in the question is true.
Final Statement
Thus, the weight of a person on Earth depends on the gravitational force exerted by the Earth.
---
Weight is defined as the force exerted on an object due to gravity. It is calculated using the formula:
Weight(W)=mass(m)×acceleration due to gravity(g)
In this formula:
- m is the mass of the person (measured in kilograms).
- g is the acceleration due to gravity on Earth, which is approximately 9.81m/s2.
When a person stands on the surface of the Earth, their weight can be calculated by multiplying their mass by the acceleration due to gravity:
W=m×g
This means that the weight of the person is directly proportional to their mass and the gravitational acceleration.
The weight of a person on Earth indeed depends on the gravitational force exerted by the Earth. If the gravitational force changes (for example, if the person were on another planet), their weight would also change. Therefore, the statement in the question is true.
Thus, the weight of a person on Earth depends on the gravitational force exerted by the Earth.
Topper's Solved these Questions
- FORCE AND PRESSURE : MOTION BOOK - ICSECHAPTER - FORCE AND PRESSURE : MOTION EXERCISE - EXERCISES (SECTION I) (Write T for True and F for False. Correct the false statements.)
1 Videos
- FORCE AND PRESSURE : MOTION BOOK - ICSECHAPTER - FORCE AND PRESSURE : MOTION EXERCISE - EXERCISES (SECTION I) (Choose the correct option to fill in the blank.)
7 Videos
- FORCE AND PRESSURE : MOTION BOOK - ICSECHAPTER - FORCE AND PRESSURE : MOTION EXERCISE - QUESTIONS (Write T for True and F for False. Correct the false statements.)
5 Videos
- ENERGYBOOK - ICSECHAPTER - ENERGYEXERCISE - EXERCISE (PICTURE STUDY)
3 Videos
- HEATBOOK - ICSECHAPTER - HEATEXERCISE - EXERCISE (PICTURE)
10 Videos
Similar Questions
A satellite revolves around earth under the gravitational force exerted upon it the earth. Does it perform any work ?
View SolutionName the following.
Gravitational force exerted by the Earth on an object .
View SolutionThe gravitational force exerted by the Sun on the Moon is about twice as great as the gravitational force exerted by the earth on the Moon, but still Moon is not escaping from the gravitational influence of the earth. Mark the option which correctly explains the above system.
View SolutionLet F1 be the magnitude of the gravitational force exerted on the Sun by Earth and F2 be the magnitude of the force exerted on Earth by the Sun. Then:
View SolutionWrite the formula to find the magnitude of the gravitational force between the Earth and an object on the surface of the Earth.
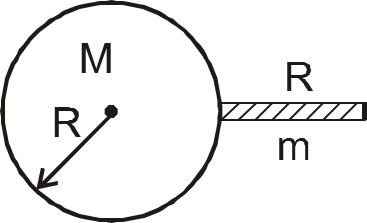
View SolutionA uniform thin rod of mass m and length R is placed normally on surface of earth as shown. The mass of earth is M and its radius R. Then the magnitude of gravitational force exerted by earth on the rod is

View SolutionThe centripetal force on a satellite orbiting round the earth and the gravitational force of earth acting on the satellite both equal F. The net force on the satellite is
View SolutionIt is known that the time of revolution T of a satellite around the earth depends on the universal gravitational constant G, the mass of the earth M, and the radius of the circular orbit R. Obtain an expression for T using dimensional analysis.
View SolutionA body of weight 72 N moves from the surface of earth at a height half of the radius of earth , then geavitational force exerted on it will be
View SolutionA uniform thin rod of mass m and length R is placed normally on surface of earth as shown. The mass of earth is M and its radius is R. If the magnitude of gravitational force exerted by earth on the rod is ηGMm12R2 then η is
View Solution
