A rock on a cliff has more energy than a rock resting on the ground.
To solve the question, "A rock on a cliff has more energy than a rock resting on the ground," we will analyze the concept of gravitational potential energy and how it applies to both rocks.
1. Understanding the Situation:
- We have two rocks: one resting on the ground and another on a cliff at a height h.
2. Defining Energy Types:
- The energy we are concerned with here is gravitational potential energy (GPE), which is the energy an object possesses due to its position in a gravitational field.
3. Formula for Gravitational Potential Energy:
- The formula for gravitational potential energy is given by:
Potential Energy (PE)=mgh
where:
- m = mass of the rock,
- g = acceleration due to gravity (approximately 9.81m/s2),
- h = height above the ground.
4. Calculating the Energy of Each Rock:
- For the rock resting on the ground, its height h=0, so its gravitational potential energy is:
PEground=mg⋅0=0Joules
- For the rock on the cliff, its height is h, so its gravitational potential energy is:
PEcliff=mgh
5. Comparing the Energies:
- The rock on the cliff has gravitational potential energy mgh, while the rock on the ground has 0Joules.
- Therefore, the rock on the cliff has more energy than the rock resting on the ground by an amount equal to its gravitational potential energy:
Energy difference=mgh−0=mgh
6. Conclusion:
- Thus, we conclude that a rock on a cliff has more energy than a rock resting on the ground due to its height above the ground, which contributes to its gravitational potential energy.
- We have two rocks: one resting on the ground and another on a cliff at a height h.
- The energy we are concerned with here is gravitational potential energy (GPE), which is the energy an object possesses due to its position in a gravitational field.
- The formula for gravitational potential energy is given by:
Potential Energy (PE)=mgh
where:
- m = mass of the rock,
- g = acceleration due to gravity (approximately 9.81m/s2),
- h = height above the ground.
- For the rock resting on the ground, its height h=0, so its gravitational potential energy is:
PEground=mg⋅0=0Joules
- For the rock on the cliff, its height is h, so its gravitational potential energy is:
PEcliff=mgh
- The rock on the cliff has gravitational potential energy mgh, while the rock on the ground has 0Joules.
- Therefore, the rock on the cliff has more energy than the rock resting on the ground by an amount equal to its gravitational potential energy:
Energy difference=mgh−0=mgh
- Thus, we conclude that a rock on a cliff has more energy than a rock resting on the ground due to its height above the ground, which contributes to its gravitational potential energy.
Topper's Solved these Questions
- WORK AND ENERGYBOOK - ICSECHAPTER - WORK AND ENERGYEXERCISE - Exercises (Section II) Distinguish between the following
4 Videos
- WORK AND ENERGYBOOK - ICSECHAPTER - WORK AND ENERGYEXERCISE - Exercises (Section II) Short answer questions
8 Videos
- WORK AND ENERGYBOOK - ICSECHAPTER - WORK AND ENERGYEXERCISE - Exercises (Choose the correct option to fill in the blank)
5 Videos
- SOUNDBOOK - ICSECHAPTER - SOUNDEXERCISE - Picture Study
4 Videos
Similar Questions
Knowledge Check
A rock is dropped off a cliff and strikes the ground with an impact velocity of 30m/s. How high was the cliff ?
A15mB20mC30mD45 m
A rock is dropped off a cliff that's 80 m high. If it strikes the ground with an impact velocity of 40m/s, what acceleration did it experience during its descent ?
View SolutionA rock is dropped from an 80- meter cliff. How long does it take to reach the ground ?
View SolutionA stone dropped from a cliff hits the ground in 4 sec. Height of the cliff is
View SolutionFor a certain reacation large fraction of molecules has energy more than energy more than the threshold enrgy,yet the rate of reaction is very slow. Why ?
View SolutionA stone is dropped from top of a cliff.It is seen to hit the ground below after 4.2 s. How high is the cliff?
View SolutionA rock of mass m is dropped to the ground from a height h. A second rock, with mass 2m, is dropped from the same height. When the second rock strikes the ground, what is its kinetic energy? (a) Twice that of the first rock, (b) four times that of the first rock, (c) same as that of the first rock, (d) half as much as that of the first rock, (e) impossible to determine.
View Solution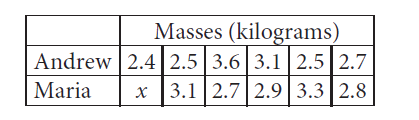
Andrew and Maria each collected six rocks, and the masses of the rocks are shown in the table above. The mean of the masses of the rocks Maria collected is 0.1 kilogram greater than the mean of the masses of the rocks Andrew collected. What is the value of x ?
View Solution
