An imaginary line passing through the pole and the centre of curvature of the spherical mirror
To solve the question regarding the imaginary line passing through the pole and the center of curvature of a spherical mirror, we can follow these steps:
1. Understand the Components of a Spherical Mirror:
- A spherical mirror has two main components: the pole and the center of curvature.
- The pole (P) is the central point of the mirror's surface.
- The center of curvature (C) is the center of the sphere from which the mirror is made.
2. Identify the Imaginary Line:
- The question asks for an imaginary line that connects the pole and the center of curvature.
- This line is not physically drawn on the mirror but is an important conceptual tool in optics.
3. Define the Imaginary Line:
- The imaginary line that connects the pole (P) and the center of curvature (C) is known as the principal axis.
- This line is crucial in understanding how light behaves when it interacts with the mirror.
4. Conclusion:
- Therefore, the answer to the question is that the imaginary line passing through the pole and the center of curvature of the spherical mirror is called the principal axis.
Final Answer:
The imaginary line passing through the pole and the center of curvature of the spherical mirror is called the principal axis.
---
- A spherical mirror has two main components: the pole and the center of curvature.
- The pole (P) is the central point of the mirror's surface.
- The center of curvature (C) is the center of the sphere from which the mirror is made.
- The question asks for an imaginary line that connects the pole and the center of curvature.
- This line is not physically drawn on the mirror but is an important conceptual tool in optics.
- The imaginary line that connects the pole (P) and the center of curvature (C) is known as the principal axis.
- This line is crucial in understanding how light behaves when it interacts with the mirror.
- Therefore, the answer to the question is that the imaginary line passing through the pole and the center of curvature of the spherical mirror is called the principal axis.
The imaginary line passing through the pole and the center of curvature of the spherical mirror is called the principal axis.
Similar Questions
An imaginary line passing through the pole and the centre of curvature of a spherical mirror is called the _____
View SolutionWhat is a spherical mirror?
View SolutionM-line passes through the centre of
View SolutionThe geometrical centre of a spherical mirror
View SolutionA pole of height 4 m is kept in front of a vertical plane mirror
of length 2m. The lower end of the mirror is at a height of 6m form the grown
.the horizontal distance betweem the mirror and the pole is 2m. Upto what
minimum and maximum heights a man can see the image of top of the pole at a
horizontal distance of 4m (from the mirror O standing on the same horizontal
line which is passing through the pole and the horizontal point below the
mirror?

View Solution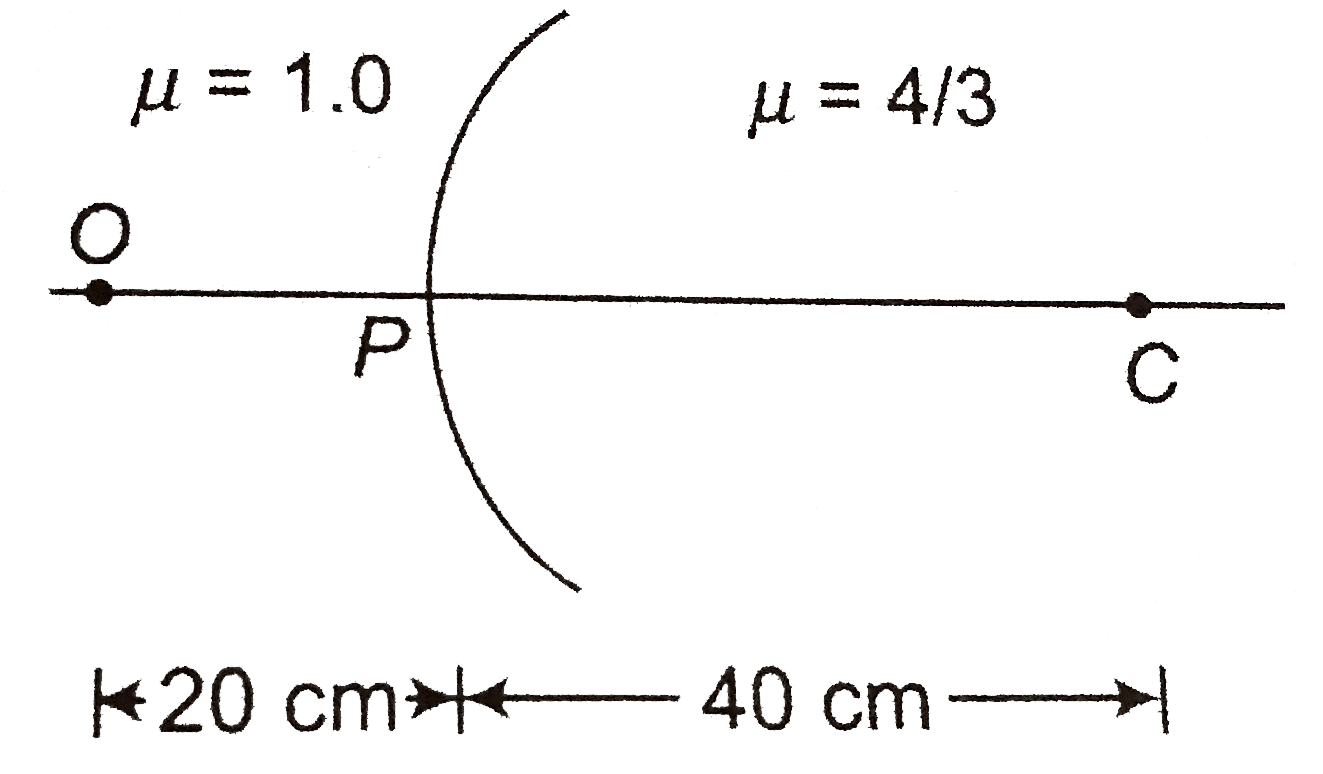
Locate the image of the point object O. The point C is centre of curvature of the spherical surface.
View SolutionDefine the terms pole, principal axis and centre of curvature with reference to a spherical mirror.
View SolutionThe geometric centre of a spherical mirror is called
View SolutionDefine principal axis of a spherical mirror.
View SolutionDefine: Radius of curvature and centre of curvature
View Solution
