Calculate the value of A, if :
(sinA−1)(2cosA−1)=0
The correct Answer is:90∘and60∘
To solve the equation (sinA−1)(2cosA−1)=0, we need to break it down into two separate equations based on the zero product property.
1. Set each factor to zero:
sinA−1=0or2cosA−1=0
2. Solve the first equation:
sinA−1=0⟹sinA=1
The sine function equals 1 at:
A=90∘
3. Solve the second equation:
2cosA−1=0⟹2cosA=1⟹cosA=12
The cosine function equals 12 at:
A=60∘andA=300∘(in the range of 0∘ to 360∘)
4. Final values of A:
The solutions to the original equation are:
A=90∘,60∘, and 300∘
Summary of Solutions:
The values of A that satisfy the equation (sinA−1)(2cosA−1)=0 are:
A=90∘,60∘,300∘
sinA−1=0or2cosA−1=0
sinA−1=0⟹sinA=1
The sine function equals 1 at:
A=90∘
2cosA−1=0⟹2cosA=1⟹cosA=12
The cosine function equals 12 at:
A=60∘andA=300∘(in the range of 0∘ to 360∘)
The solutions to the original equation are:
A=90∘,60∘, and 300∘
The values of A that satisfy the equation (sinA−1)(2cosA−1)=0 are:
A=90∘,60∘,300∘
Topper's Solved these Questions
- BOOK - ICSECHAPTER - TRIGONOMETRICAL RATIOS OF STANDARD ANGLESEXERCISE - EXERCISE 23(B)34videos
TRIGONOMETRICAL RATIOS OF STANDARD ANGLES
- BOOK - ICSECHAPTER - TRIGONOMETRICAL RATIOS EXERCISE - EXERCISE 22(B)50videos
TRIGONOMETRICAL RATIOS
- BOOK - ICSECHAPTER - TRIGONOMETRYEXERCISE - TOPIC -4 ( COMPLEMENTARY ANGLES ) (4 MARKS QUESTIONS )4videos
TRIGONOMETRY
Similar Questions
Find the magnitude of angle A, if :
2sinAcosA−cosA−2sinA+1=0View SolutionFind the magnitude of angle A, if :
2sinAcosA−cosA−2sinA+1=0View SolutionIf cosecA=2, find the value of 1tanA+sinA1+cosA
View SolutionIf cosec A=2 , find the value of 1tanA+sinA1+cosA
View SolutionGiven that 16cotA=12 ; find the value of sinA+cosAsinA−cosA .
View Solution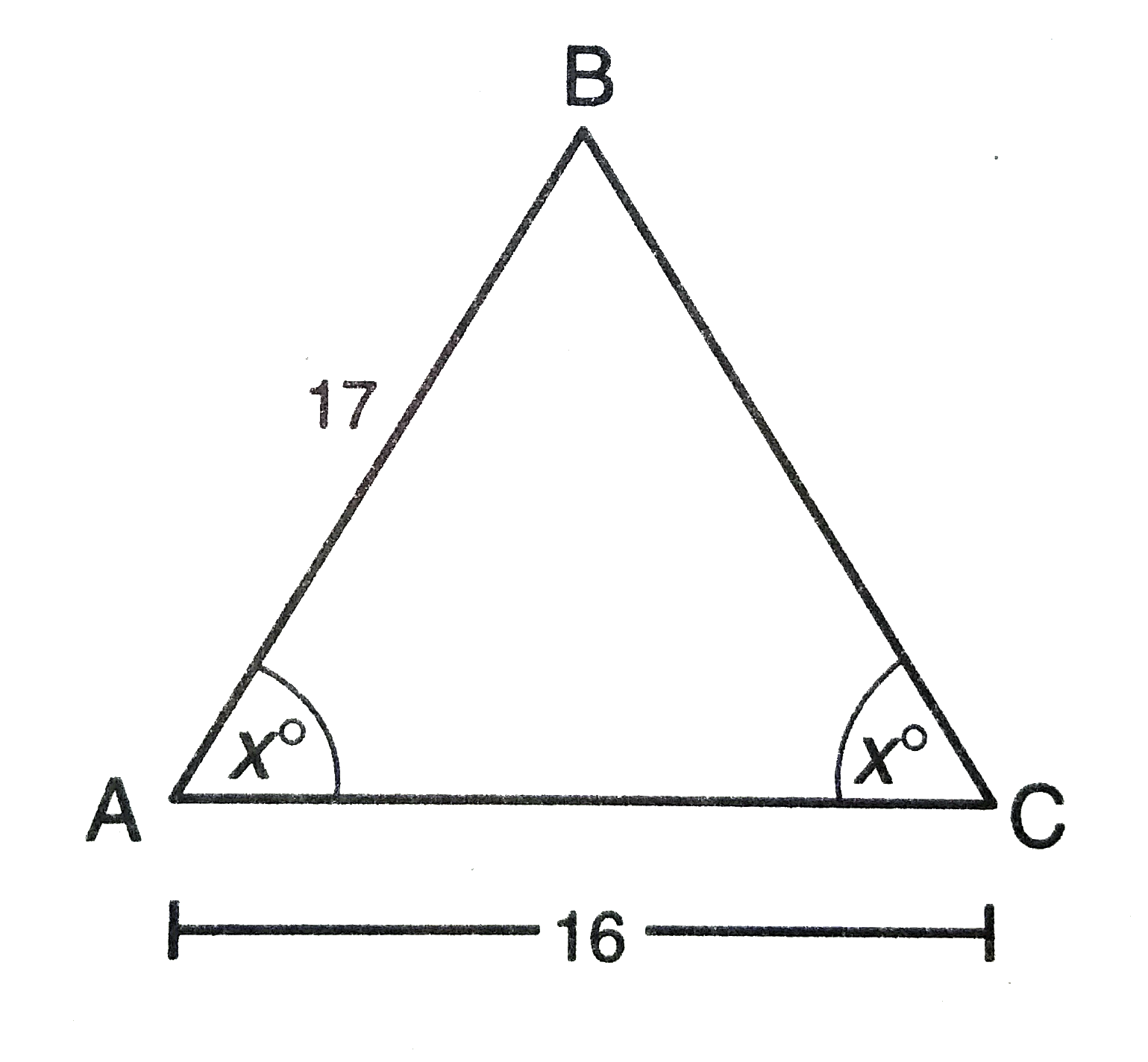
In the figure above, what is the value of sinA−cosA?View SolutionIn a ΔABC the value of ∠A is given by 5 CosA - 3 = 0 then the equation whose roots are sinA and tanA is :
View SolutionThe value of 2sinAcos3A−2sin3AcosA is
View SolutionIf sinA+cosA=0 and A lies in 4th quadrant, find the values of sinA and cosA.
View SolutionIn a right - angled triangle , it is given that A is an acute angle and tanA=512
cosA+sinAcosA−sinAView Solution

