Find the values o x and y if:
(3x−1,2y−7)=(9,−9)
The correct Answer is:x=103 and y=-1
To find the values of x and y from the equation (3x−1,2y−7)=(9,−9), we can set up the following equations based on the equality of the coordinates:
1. Set up the equations:
3x−1=9(1)
2y−7=−9(2)
2. Solve for x:
From equation (1):
3x−1=9
Add 1 to both sides:
3x=9+1
3x=10
Now, divide both sides by 3:
x=103
3. Solve for y:
From equation (2):
2y−7=−9
Add 7 to both sides:
2y=−9+7
2y=−2
Now, divide both sides by 2:
y=−22=−1
4. Final values:
The values of x and y are:
x=103,y=−1
Summary of the solution:
The values of x and y are 103 and −1 respectively.
3x−1=9(1)
2y−7=−9(2)
From equation (1):
3x−1=9
Add 1 to both sides:
3x=9+1
3x=10
Now, divide both sides by 3:
x=103
From equation (2):
2y−7=−9
Add 7 to both sides:
2y=−9+7
2y=−2
Now, divide both sides by 2:
y=−22=−1
The values of x and y are:
x=103,y=−1
The values of x and y are 103 and −1 respectively.
Topper's Solved these Questions
- CO-ORDINATE GEOMETRYBOOK - ICSECHAPTER - CO-ORDINATE GEOMETRYEXERCISE - EXERCISE 26(B)
46 Videos
- CO-ORDINATE GEOMETRYBOOK - ICSECHAPTER - CO-ORDINATE GEOMETRYEXERCISE - EXERCISE 26(C)
35 Videos
- CIRCLES BOOK - ICSECHAPTER - CIRCLES EXERCISE - TOPIC-2
25 Videos
- COMPLEMENTARY ANGLESBOOK - ICSECHAPTER - COMPLEMENTARY ANGLESEXERCISE - EXERCISE
64 Videos
Similar Questions
Find the value of 'x' and 'y' if :
2[x79y−5]+[6−745]=[1072215]
View SolutionFind the value of xand y when
3x+y=3
9x−3y=9
View SolutionFind for values of x and y 3x+2y=5 2x−3y=7
View Solution3x−y=3,<br> find the value of x and y 9x−3y=9
View SolutionFind value of x and y: (5x−4y+8=0),(7x+6y−9=0)
View SolutionFind value of x and y: (5x−4y+8=0),(7x+6y−9=0)
View SolutionFind the value of 27x3+ 8y3, if (i)3x+2y=14 and xy=8 (ii)3x+2y=20 and xy=149
View SolutionFind x and y: 2x+3y=9,3x−y=1
View SolutionFind the values of x and y in the following rectangle :
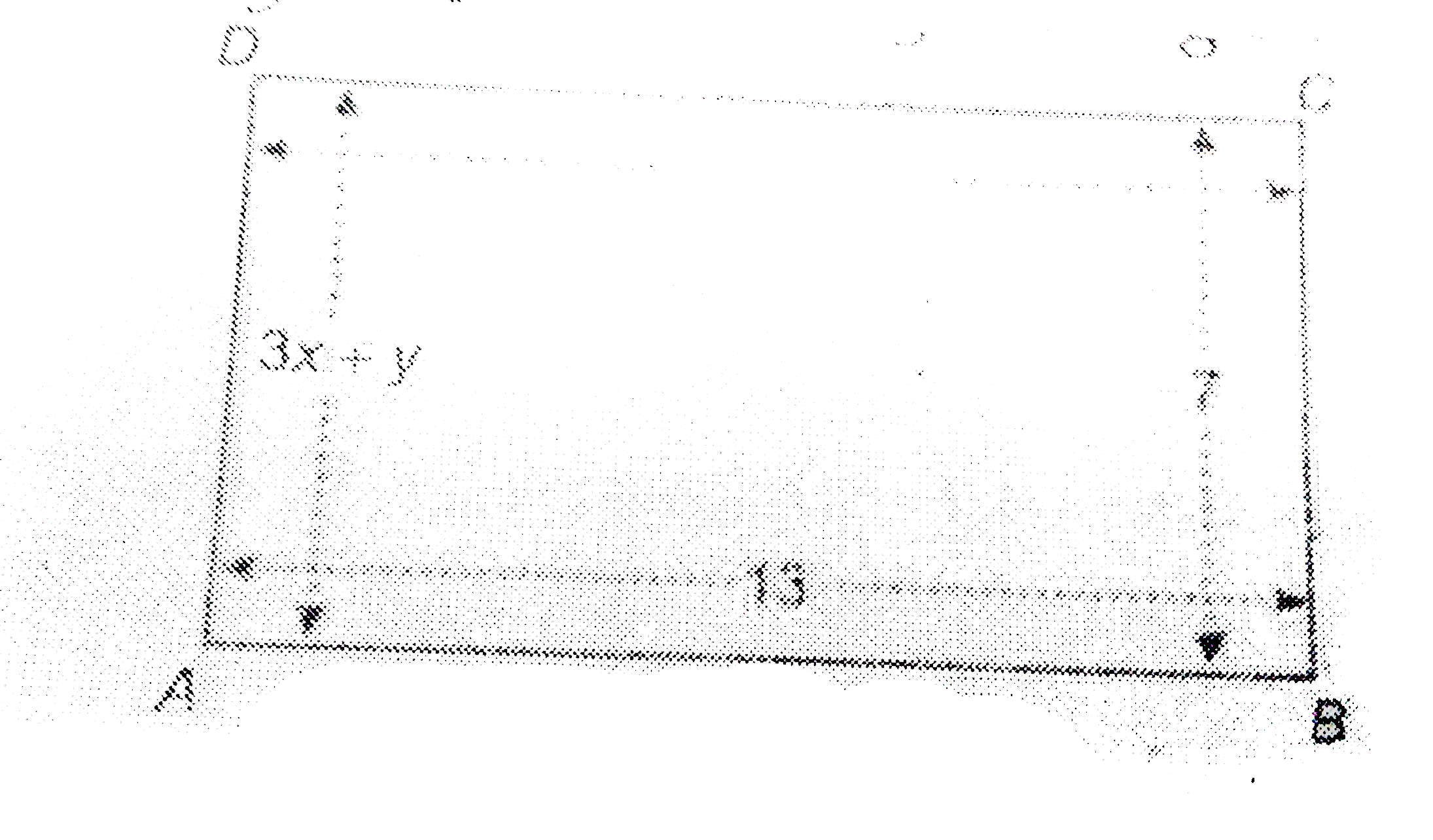
We know that the opposite sides of a rectangle are equal
∴ 3x + y = 7 …(1)
x + 3y = 13 …(2)
9x + 3y = 21 [from (1)]
x + 3y = 13 - - -––––––––––––On subtracting, 8x =8
x = 1
Now, from (1), 3 (1) + y = 7
implies y = 7 - 3
implies y = 4
Hence, the required values of x and y are 1 and 4 respectively.
View SolutionIf 7x−15y=4x+y, find the value of x:y. Hence, use componendo and dividendo to find the values of :
(i) 9x+5y9x−5y
(iI) 3x2+2y23x2−2y2
View Solution
