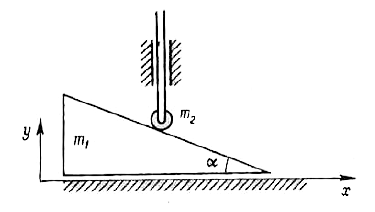
A rod of mass m2 rests on wedge of mass m1 (Fig.). Guides allow the rod to move only in the direction of the y-axis and the wedge only in the direction of the x-axis. Find the acceleration of both bodies and the reaction of the wedge. Neglect friction.
The correct Answer is:a1=−g=m3m2tanα+m1cotα,a2=−gm2tanαm2tanα+m1cotα,Q=m1m2gcosαm2sin2α+m1cos2α.
Three forces act on the rod: the reaction of the wedge Q, the reaction of the guides F and the gravitational force m2g (Fig.). The equation of motion (in the y-direction) is
−m2g+Qcosα=m2a2
Correspondingly, three forces also act on the wedge: the reaction of the table N, the gravitational force m1g, and the reaction of the rod Q
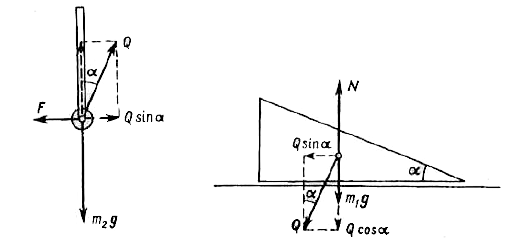
(Fig). The equation of motion (in the x-direction) is
−Qsinα=m1a1
To obtain the third equation, we compare the displacements of the rod Δy=1/2a2t2 with that of the wedge Δx=1/2a1t2. Since Δy=Δxtanα it follows that
a2=a1tanα
Then we solve the system of three equations with three unknowns.
Similar Questions
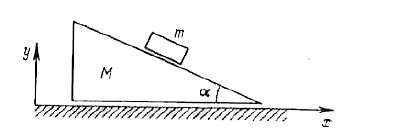
A block of mass m is placed on wedge of mass M (Fig.). Find the accelerations of the block and the wedge in the reference system fixed to the table, and the reaction. Friction is to be neglected.
Analyse the limiting case when the wedge remains stationary.
View SolutionIn the arrangement shown in the fig. the block of mass m lies on the wedge. Wedge is moving with acceleration a = 5 m/sec . Find the wedge acceleration of the block with respect to wedge.
View Solution
Knowledge Check
In fig. mass m is being pulled on the incline of a wedge of mass M. All the surfaces are smooth. Find the acceleration of the wedge.
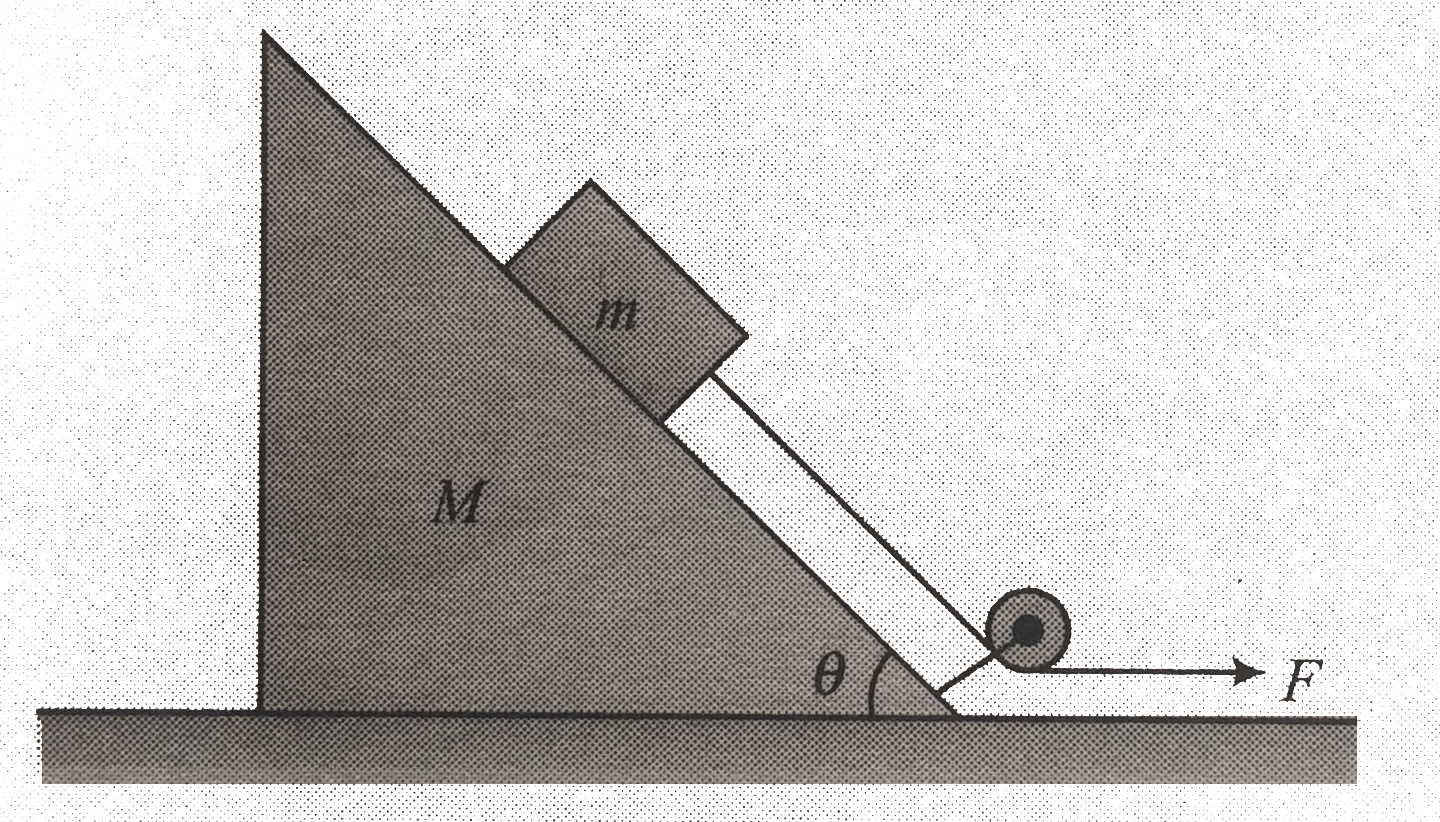 Aa=F(1−cosθ)mg2sinθcosθM+2msin2θBa=F(1+cosθ)+mgsinθcosθM+msin2θCa=F(1−cosθ)−mgsinθcosθM+msin2θDa=F(1+cosθ)−mgsinθcosθM−msin2θ
Aa=F(1−cosθ)mg2sinθcosθM+2msin2θBa=F(1+cosθ)+mgsinθcosθM+msin2θCa=F(1−cosθ)−mgsinθcosθM+msin2θDa=F(1+cosθ)−mgsinθcosθM−msin2θIn the system shown, the initial acceleration of the wedge of mass 5M is (there is no friction anywhere)
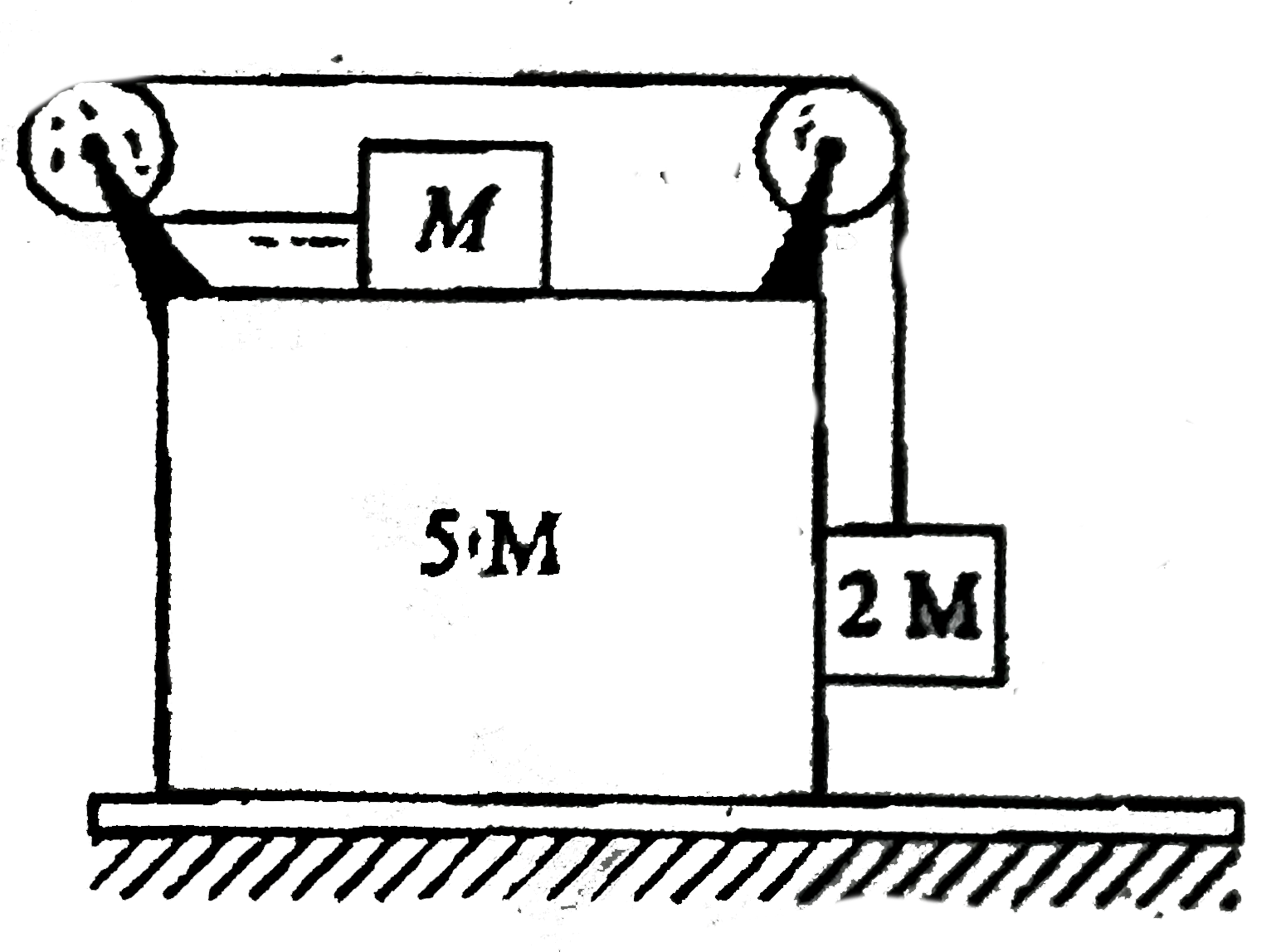 AzeroBg/2Cg/3Dg/4
AzeroBg/2Cg/3Dg/4
In the arrangement shown in the fig. the block of mass m lies on the wedge. Wedge is moving with acceleration a = 5 m/sec Find the wedge acceleration of the block with respect to wedge.
View SolutionIn fig. mass m is being pulled on the incline of a wedge of mass M. All the surfaces are smooth. Find the acceleration of the wedge.

View SolutionIn the Fig. shown, mass 'm' is being pulled on the incline of a wedge of mass M. All the surface are smooth. Find the acceleration of the wedge.
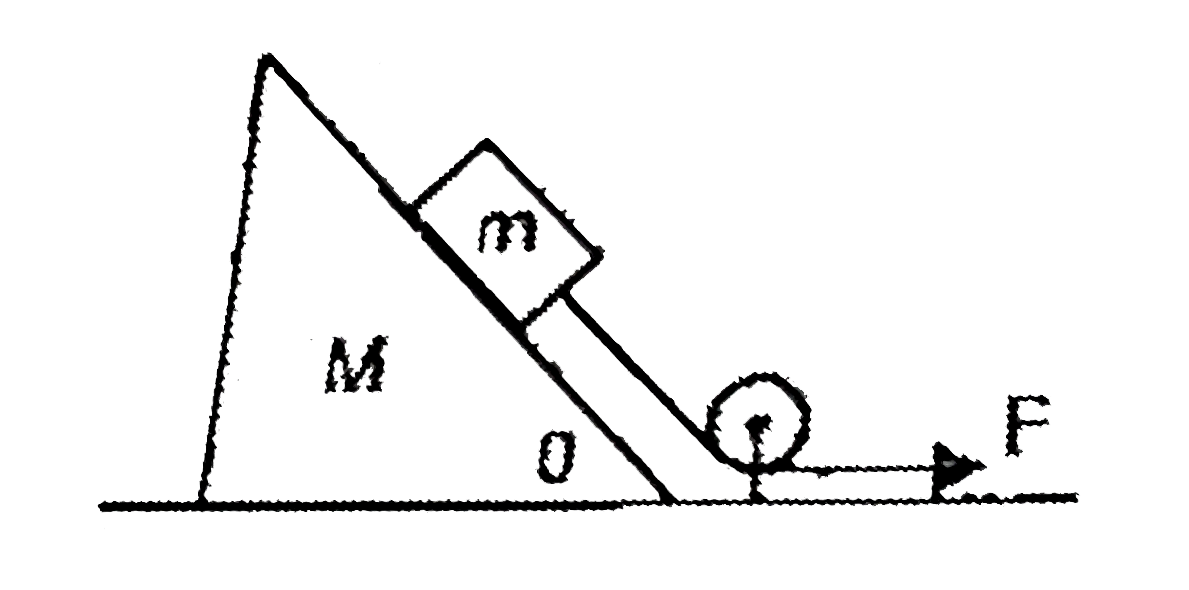
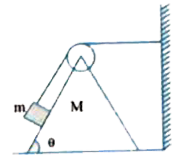
View SolutionA block of mass m is arranged on the wedge as shown in figure . The wedge angle is θ. If the masses of pulley and thread and negligible and friction is absent , find the acceleration of the wedge
View SolutionNeglect friction. Find acceleration of m,2mand3m as shown in the figure. The wedge is find.
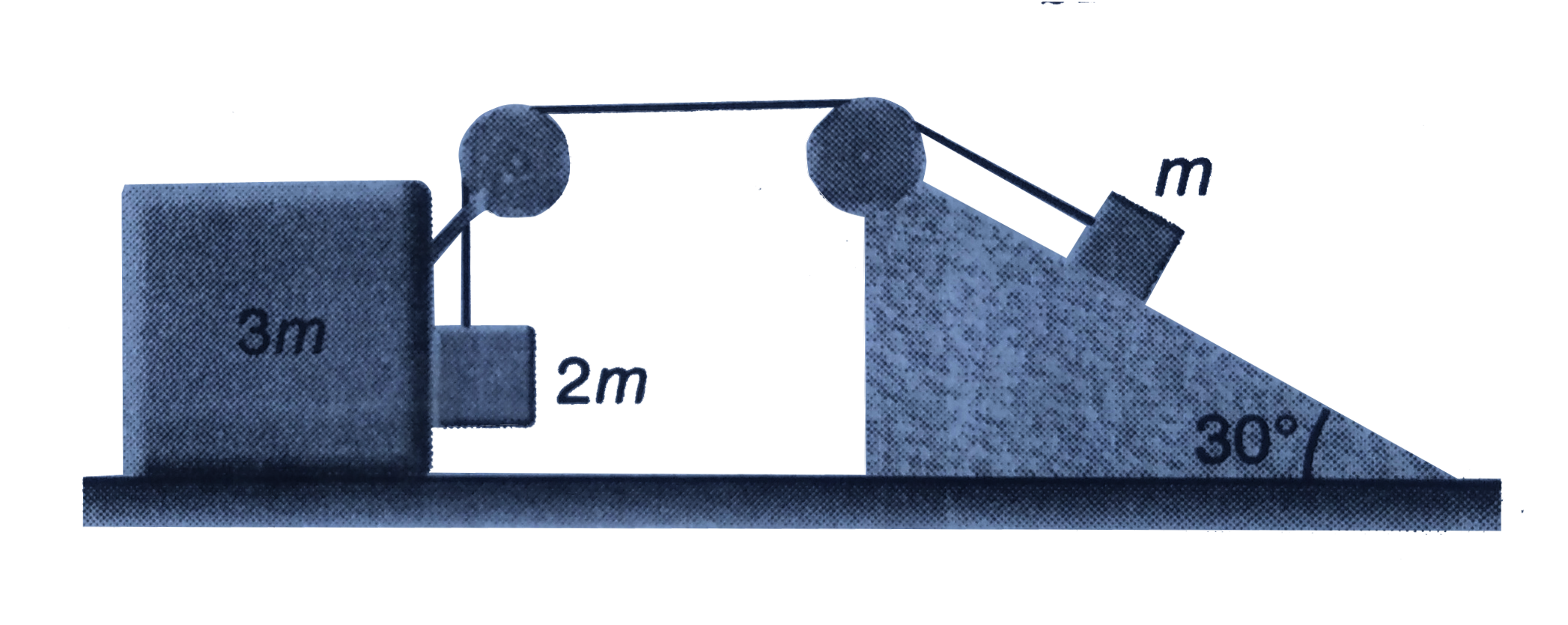
View SolutionNeglect friction. Find acceleration of m,2mand3m as shown in the figure. The wedge is find.

View Solution
