Question

The equation for a parabola is of the form ${\displaystyle x^2=2py}$, where the parameter ${\displaystyle p>0}$. Find the radius of curvature of the parabola at each point.

Answer

`r=p(1+(x^(2))/(p^(2)))^(3//2)`

Explanation

Suppose that an object is thrown in the direction of the x-axis at an initial speed `v_(0)` and that the force of gravity imparts an acceleration g in the positive direction of the y-axis to the object (Fig.). Then the law of motion will assume the form
`x=v_(0)t , y=1//2 g t^(2)`
The equation for the path is obtained by eliminating the time: `x_(2)=(2v_(0)^(2))/(g)y`, i.e. the parameter `p=v_(0)^(2)//g`. It may be seen from the figure that
`v= sqrt(v_(0)^(2) +g^(2)t^(2)) - v_(0) sqrt(1+x^(0)//mu^(0)), a_(n)- g cos alpha = g v_(0)//v`

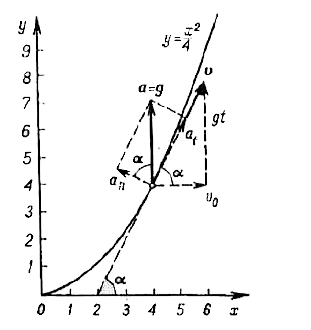
Substituting the results into the expression for the radius of curvature,
We obtain
`r=(v^(2))/(a_(n)) = (v^(3))/(gv_(0)) = p(1+(x^(2))/(p^(2)))^(3//2)`